The chord of contact of the tangent from a point on the circle x2+y2=a2 to the circlex2+y2=b2 touches the circle x2+y2=c2, then a, b, c are in :
A.P
G.P
H.P
A.G.P
The correct Answer is:B
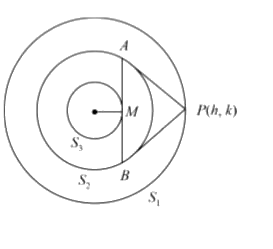
Let P(h,k) lie on S1∴h2+k2=a2 …(i)
Equation of chord of contact from P to S2 is x⋅h+y⋅k=b2 ….(ii)
This line is a tangent to the circle S3.
∴ Distance from centre = radius
(−b2)√h2+k2=c
∣∣b2∣∣√h2+k2=c
∣∣b2∣∣√a2=c⇒b2=ac
∴ a,b,c........in G.P
Similar Questions
If the chord of contact of the tangents drawn from a point on the circle x2+y2+y2=a2 to the circle x2+y2=b2 touches the circle x2+y2=c2 , then prove that a,b and c are in GP.
View Solutionchord of contact of the tangents drawn from a point on the circle x2+y2=81 to the circle x2+y2=b2 touches the circle x2+y2=16, then the value of b is
View Solution
Knowledge Check
The chord of contact of tangents drawn from any point on the circle x2+y2=a2 to x2+y2=b2, touches the circle x2+y2=c2,a>b then a, b, c are in
AA.P.BG.P.CH.P.DNoneIf the chord of contact of tangents from a point P(h, k) to the circle x2+y2=a2 touches the circle x2+(y−a)2=a2, then locus of P is
Ay2=a2−2axBy2=a2+2axCx2=a2+2ayDx2=a2−2ay
If the chord of contact of the tangents drawn from a point on the circle x2+y2=a2 to the circle x2+y2=b2 touches the circle x2+y2=c2 , then prove that a,b and c are in GP.
View SolutionIf the chord of contact of the tangents from a point on the circle x2+y2=a2 to the circle x2+y2=b2 touch the circle x2+y2=c2, then the roots of the equation ax2+2bx+c=0 are necessarily. (A) imaginary (B) real and equal (C) real and unequal (D) rational
View SolutionIf the chord of contact of the tangents from a point on the circle x2+y2=a2 to the circle x2+y2=b2 touch the circle x2+y2=c2, then the roots of the equation ax2+2bx+c=0 are necessarily. (A) imaginary (B) real and equal (C) real and unequal (D) rational
View SolutionIf the chord of contact of the tangents from a point on the circle x2+y2=a2 to the circle x2+y2=b2 touch the circle x2+y2=c2, then the roots of the equation ax2+2bx+c=0 are necessarily. (A) imaginary (B) real and equal (C) real and unequal (D) rational
View Solution(prove that) If the polar of the points on the circle
x2+y2=a2 with respect to the circle
x2+y2=b2 touches the circle x2+y2=c2
then prove that a, b, c, are in Geometrical
progression.
View SolutionIf the chord of contact of tangents from a point P(h, k) to the circle x2+y2=a2 touches the circle x2+(y−a)2=a2, then locus of P is
View Solution
