The circuit diagram shown in fig consists of a very large (infinite) number of elements . The resistances of the resistors in each subsequent element differ by a factor k from the resistance of the resistors in the previous element. Determine the resistance RAB between points A and B if the resistances of the first element are R1andR2
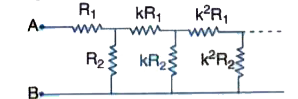
From symmetry considerations , we can remove the first element from the circuit, the resistance of the remaining circuit between point C and D will be RCD=kRAB . Therefore , the equivalent circuit of the infinite chain will have the form shown in figure.
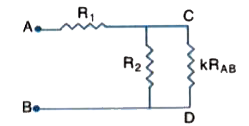
Thus
RAB=R1=R2(kRAB)R2+(kRAB)
RABR2+KRAB=R1[R2+kRAB]+kR2RAB
R2RAB+kR2AB=R1R2+kR1RAB+kR2RAB
or kR2AB+(R2+kR1−kR2)RAB−R1R2=0
∴RAB=−(R2−kR1−kR2)±√(R2−kR1−kR2)2+4kRR_22k
As resistance cannot be negative
RAB=kR1+kR2−R2+√(R2−kR1−kR2)2+4kR1R22k
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE (LONG ANSWER QUESTIONS)
2 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE (SHORT ANSWER QUESTIONS)
1 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH SERIESCHAPTER - COMMUNICATION SYSTEMS EXERCISE - PRACTICE EXERCISE (TV Antenna)
16 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE - III
73 Videos
Similar Questions
The circuit diagram shwon in the fig consist of a large number of elementws (each element has two resistors R1andR2. The resistance of the resistors in each subsequent element differs by a factor of k=12 from the resistance of the resistors in previous elements find the equivalent resistance between A and B shown in
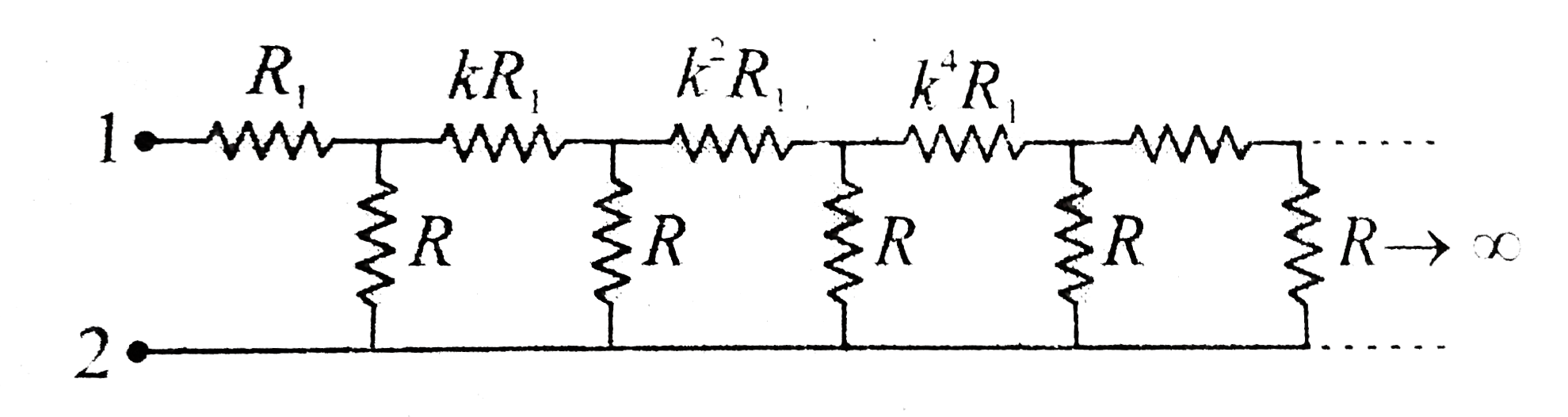
View SolutionThe circuit diagram shwon in the fig consist of a large number of elementws (each element has two resistors R1andR2. The resistacne of the resistors in each subsequnt element differs by a factro of k=12 from the resistance of the resistors in prevouys elelments find the equivalent resistanve between A and B shown in
View Solution
Knowledge Check
Each of the resistor shown in fig. has resistance R. Find the equivalent resistance between A and B
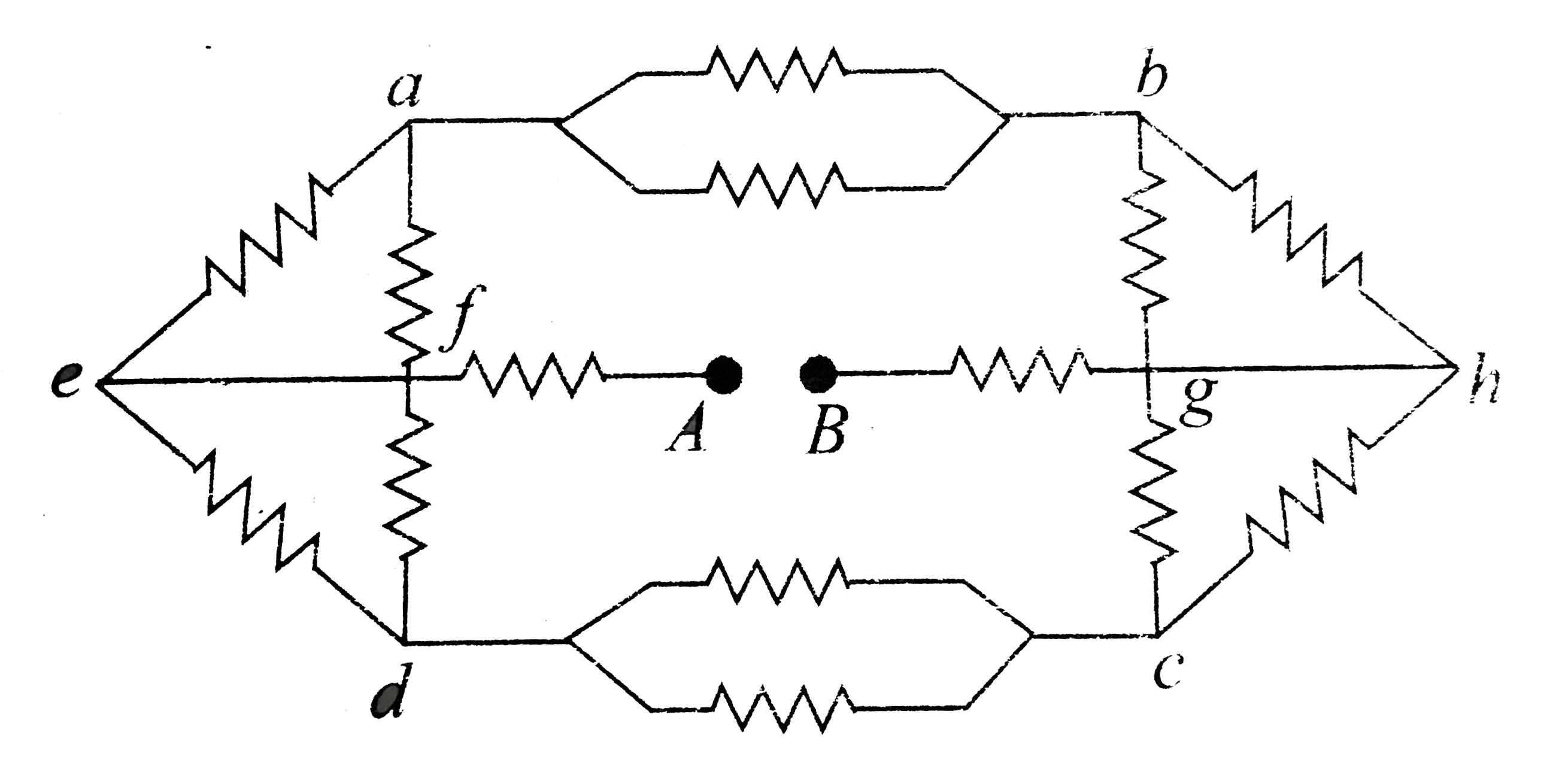 A7R4B5R4C9R4D11R4
A7R4B5R4C9R4D11R4find the equivalent resistance between A and E(resistance of each resistor is R.
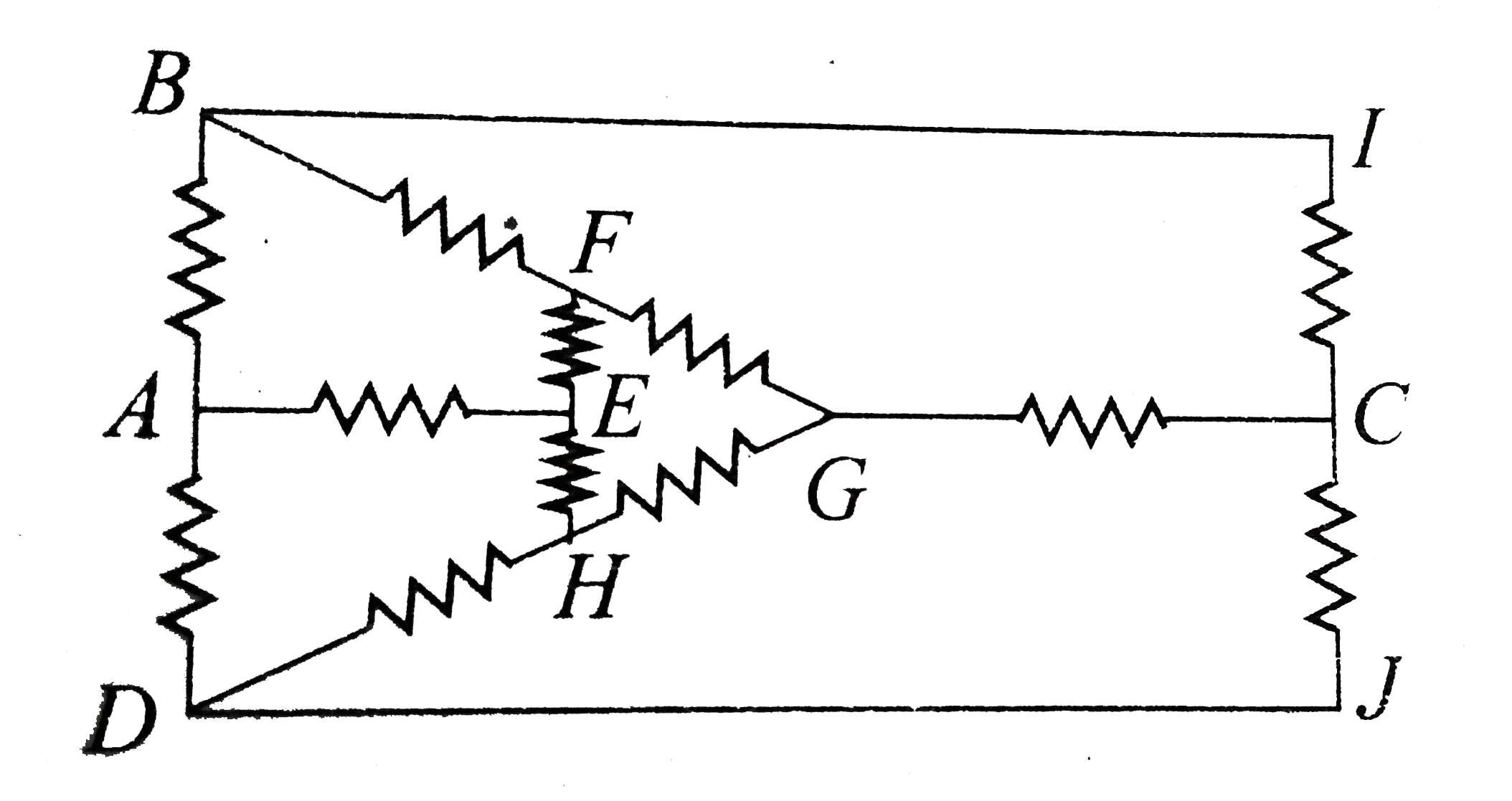 A712RB713RC715RD813R
A712RB713RC715RD813REach resistance of the circuit shown in figure is r. The equivalent resistance between A and B is
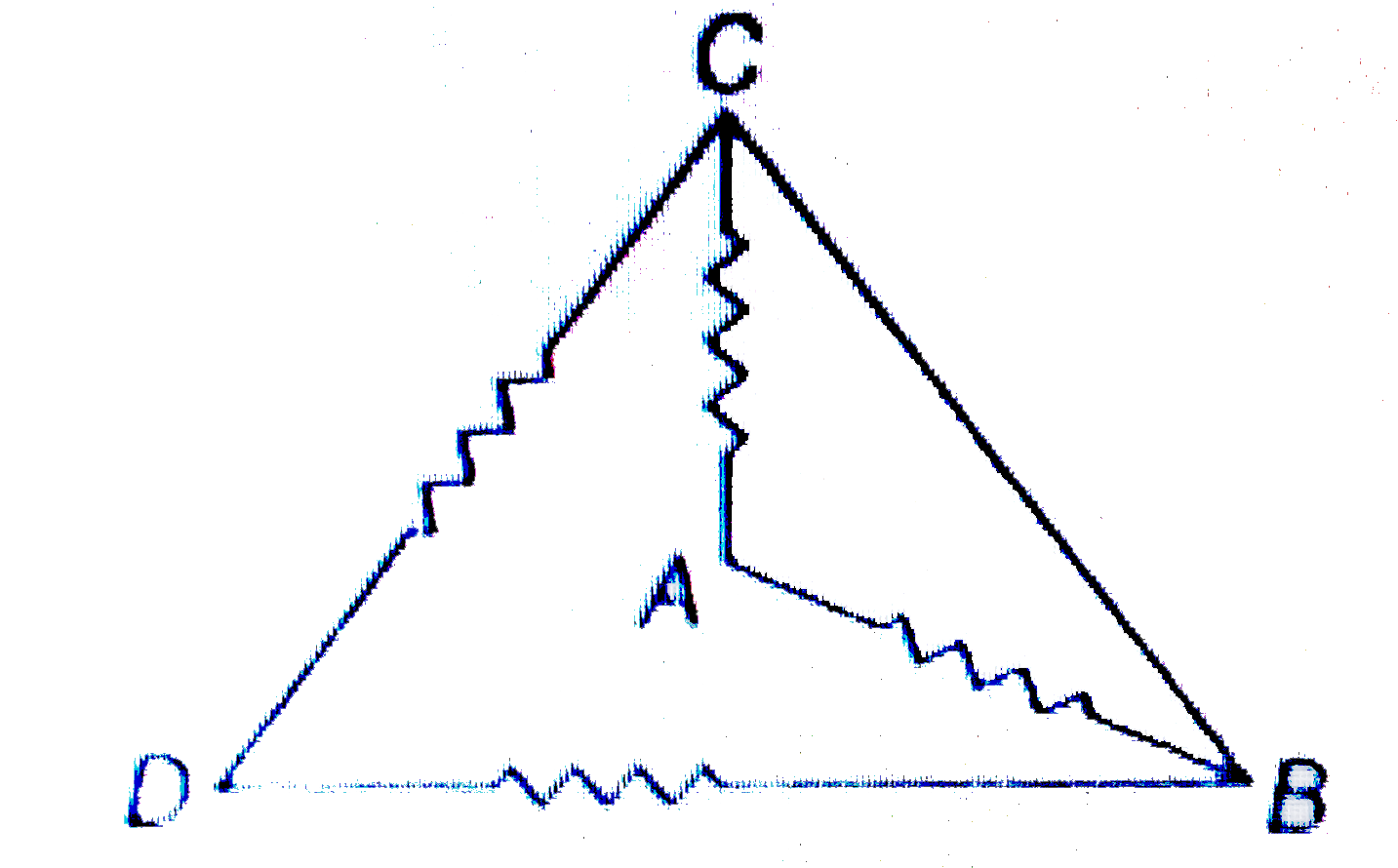 ArBr/4Cr/2D5r/8
ArBr/4Cr/2D5r/8
Each of the resistor shown in fig. has resistance R. Find the equivalent resistance between A and B

View Solutionfind the equivalent resistance between A and E(resistance of each resistor is R.

View Solution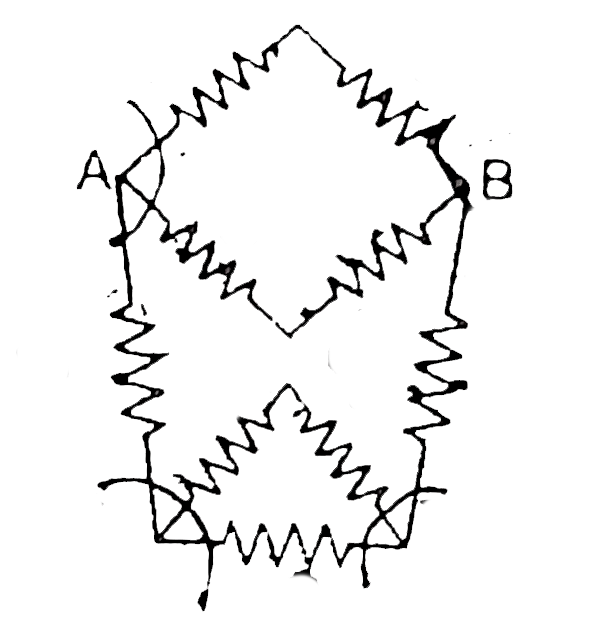
If resistance of each resistor is R then the effective resistance between A&B of the shown network is:
View Solution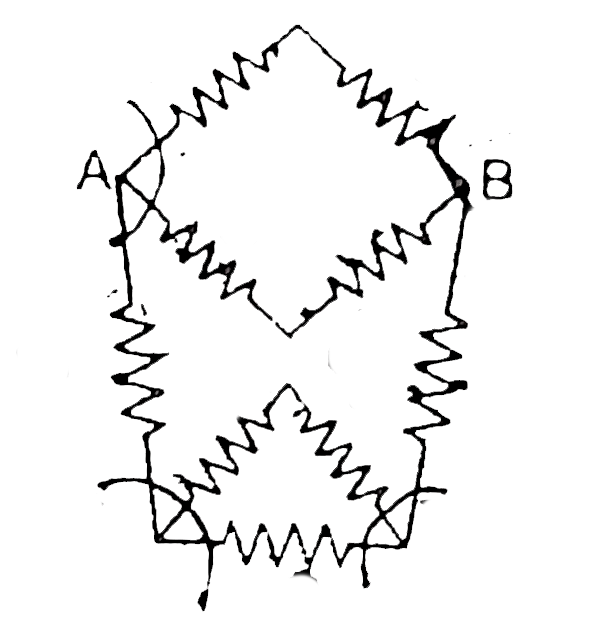
If resistance of each resistor is R then the effective resistance between A&B of the shown network is:
View SolutionEach resistance of the circuit shown in figure is r. The equivalent resistance between A and B is

View Solution
