A block A of mass m connected with a spring of force constant k is executing SHM. The displacement time equation of the block is . An identical block B moving towards negative x-axis with velocity vo collides elastically with block A at time t = 0. Then,

displacement time equation of A after collision will be
displacement time equation of A after collision will be
velocity of B just after collision will be aw towards positive x-direction.
velocity of B just after collision will be vo towards positive x-direction.
The correct Answer is:A, C
Given:
(for block A)
at t=0, and
i.e., block A is at (mean position) and its velocity is in positive x-direction. It collides elastically with an identical block B moving towards negative x-direction with velocity .
So, the blocks will interchange their velocities i.e.,
(in negative x-direction) and
(in positive x-direction) Let A be the new amplitude of block A, then from conservation of mechanical energy
New displacement-time equation for block A will be:
Topper's Solved these Questions
- BOOK - MTG-WBJEECHAPTER - OSCILLATIONS AND WAVESEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 1: SINGLE OPTION CORRECT TYPE (1 MARK))16videos
OSCILLATIONS AND WAVES
- BOOK - MTG-WBJEECHAPTER - OSCILLATIONS AND WAVESEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 2: SINGLE OPTION CORRECT TYPE (2 MARKS))3videos
OSCILLATIONS AND WAVES
- BOOK - MTG-WBJEECHAPTER - OSCILLATIONS AND WAVESEXERCISE - WB JEE WORKOUT (CATEGORY 2: SINGLE OPTION CORRECT TYPE 2 MARKS)15videos
OSCILLATIONS AND WAVES
- BOOK - MTG-WBJEECHAPTER - NUCLEAR PHYSICEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS5videos
NUCLEAR PHYSIC
- BOOK - MTG-WBJEECHAPTER - PARTICLE NATURE OF LIGHT AND WAVE PARTICLE DUALISMEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS15videos
PARTICLE NATURE OF LIGHT AND WAVE PARTICLE DUALISM
Similar Questions
A block A of mass m connected with a spring of force constant k is executing SHM. The displacement time equation of the block is x=x0+asinωt. An identical block B moving towards negative x -axis with velocity v0 collides elastically with block A at time t=0. Then
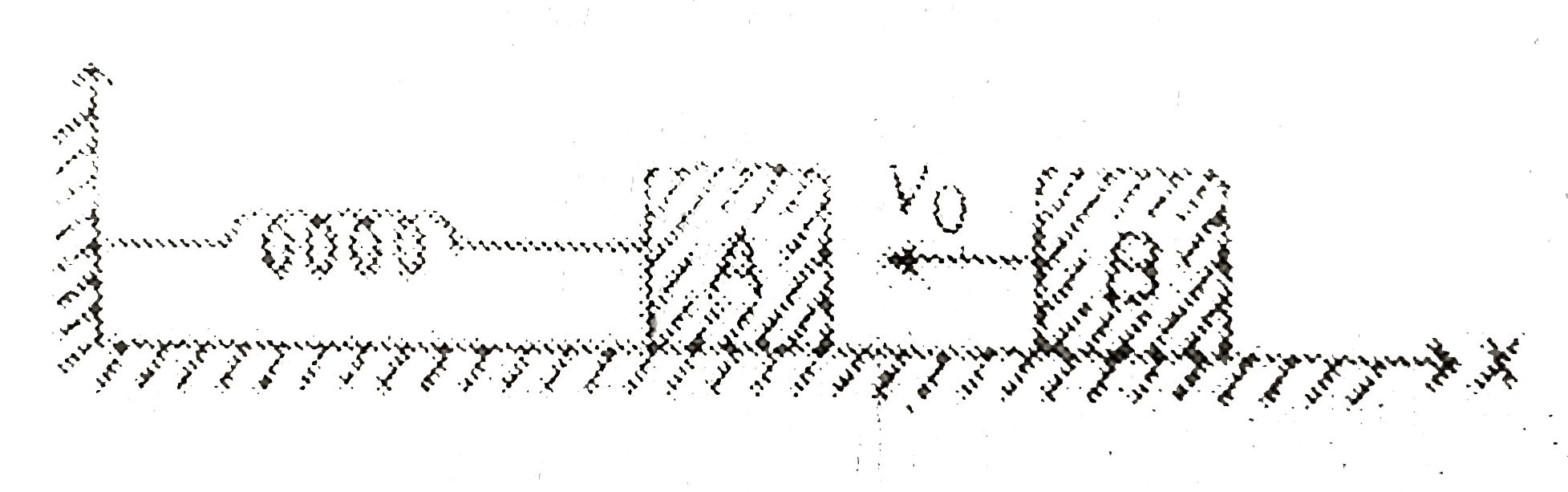 View Solution
View SolutionA block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
View Solution
Knowledge Check
- Question 1 - Select One or More
A block A of mass m connected with a spring of force constant k is executing SHM.The displacement time equation of the block is x=x0=asinωt. An indentical block B moving towards negative x -axis with velocity v0 collides elastically with block A at time t=0. Then
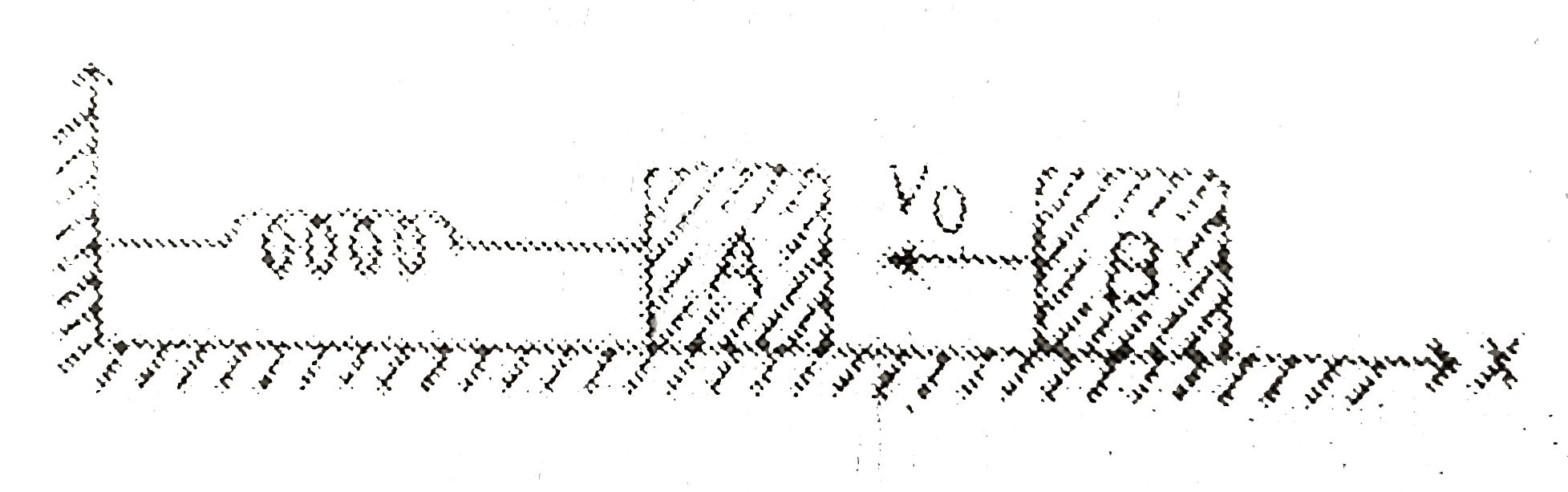 Adisplacement time equation of A after collision will be x=x0−v0√mksinωtBdisplacement time equation of A after collision will be x=x0+v0√mksinωtCVelocity of b just after collision will be a ω towards positive x-direction.Dvelocity of B just after collision will be v0 towards positive x-direction.
Adisplacement time equation of A after collision will be x=x0−v0√mksinωtBdisplacement time equation of A after collision will be x=x0+v0√mksinωtCVelocity of b just after collision will be a ω towards positive x-direction.Dvelocity of B just after collision will be v0 towards positive x-direction. - Question 2 - Select One or More
A block of mass m connected to a spring of stiffness k is placed on horizontal smooth surface. When block is at x=0, spring is in natural length. The block is also subjected to a force F=k|x|∘ thenAMotion of block is SHMBTime period of motion is π√mk[√2+√23]CTime period of motion is π√mk[1+√32]DSum of potential energy and kinetic energy of spring block system is maximum at right extreme. - Question 3 - Select One
A block of mass M is connected to a spring of force constant k and is placed on a smopth horizontal surface. The block is displaced and compressed the spring by ''A''. The block is left free to move form this position, when the block is at distance A/2 form mean position to collides elastically with the identical block. tme to be taken by block to move from exterme position to mean position is....
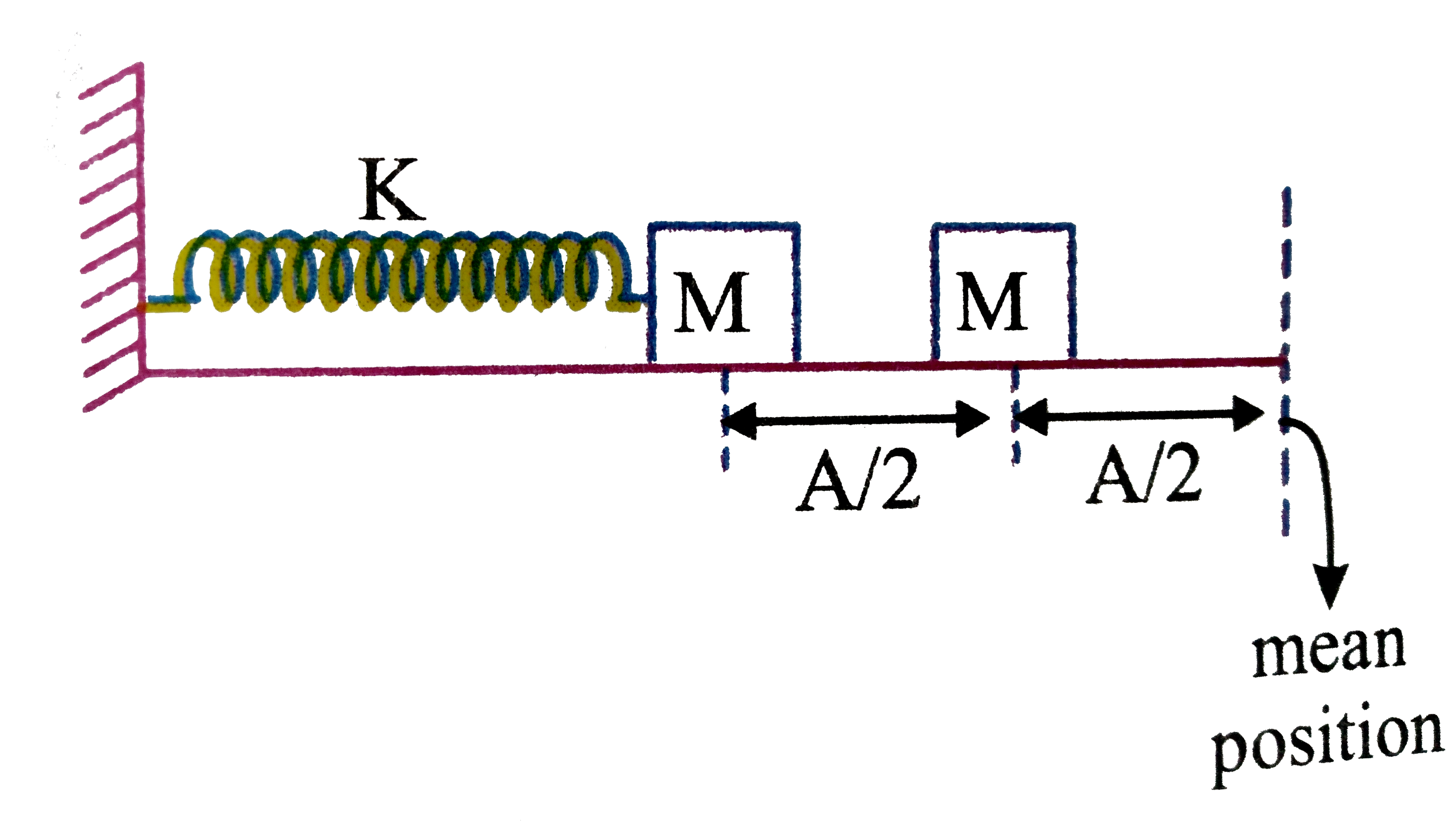 A2π√MKBπ2√MKCπ2√2MKD5π6√MK
A2π√MKBπ2√MKCπ2√2MKD5π6√MK
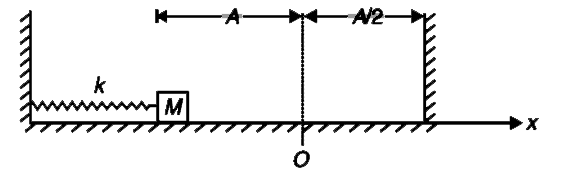
A block of mass M connected to an ideal spring of force constant k, is placed on a smooth surface. The block is pushed to the left so as to compress the spring by a length A and then it is released. The block hits an elastic wall at a distance 3A2 from its point of release. Assume the collision to be instantaneous.
(a) Calculate the time required by the block to complete one oscillation
(b) Draw the velocity - time graph for one oscillation of the block.
(c) Find the value of k for which average force experienced by the wall due to repeated hitting of the block is F0.
 View Solution
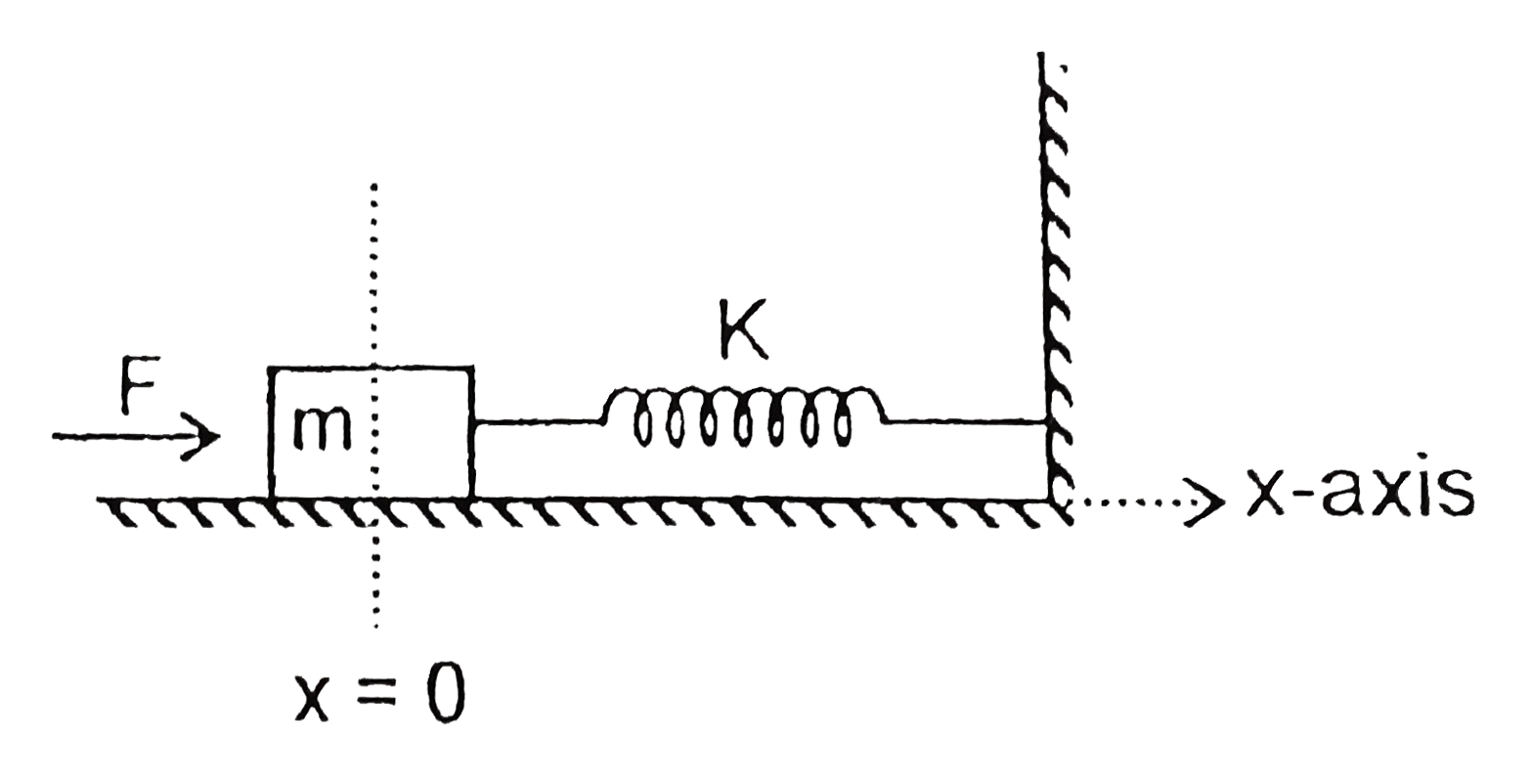
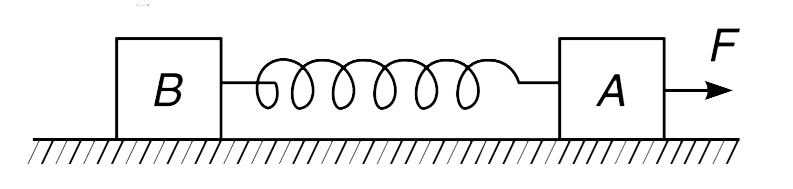
View SolutionA block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:
 View Solution
View SolutionA block of mass m is connected to another .block of mass M by a massless spring of spring constant k. A constant force f starts action as shown in figure, then:
 View Solution
View SolutionTwo blocks A and B, each of mass m, are connected by a spring of force constant K. Initially, the spring is in its natural length. A horizontal constant force F starts acting on block A at time t=0 and at time t , the extension in the spring is seen to be l. What is the displacement of the block A in time t ?
 View Solution
View SolutionA block of mass m at rest is acted upon by a force F for a time t. The kinetic energy of block after time t is
View Solution

