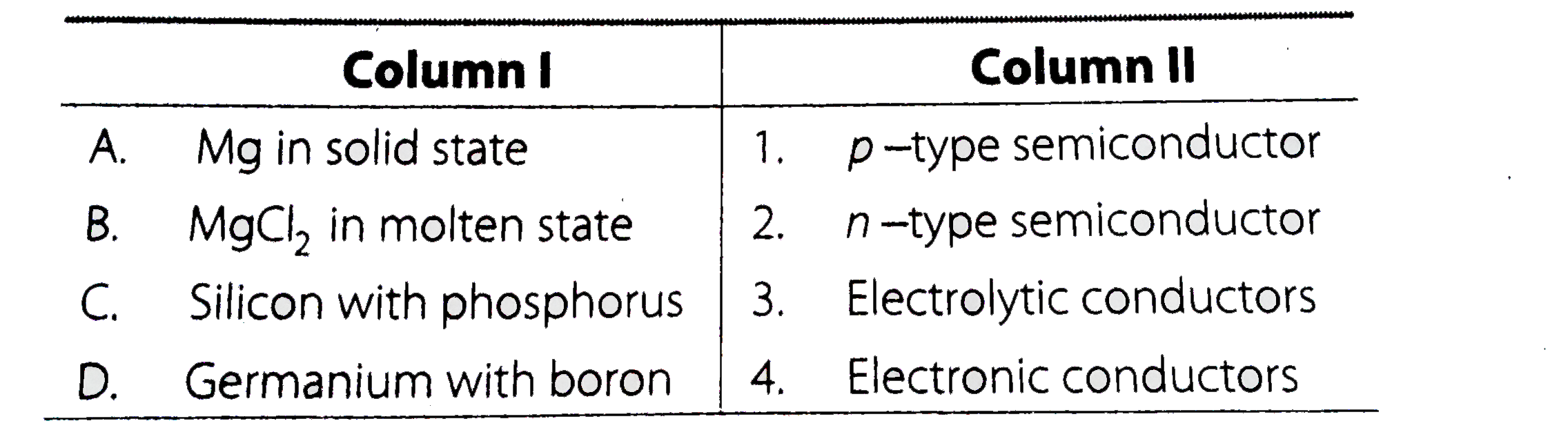considering the electron of outermost orbital of Cu match the items given the column I with their values given in column II. Column−I{(A)orbital angular momentum,(B) angular momentum in an orbit,(C) spin angular momentum} Column−II(I.4h,II.0,III.0.86h,IV.1.73)
A(II),B(I), C(III)
A(III),B(IV),C(II)
A(I),B(IV),C(II)
A(I),B(II),C(III)
The correct Answer is:A
To solve the problem, we first need to understand the electronic configuration of copper (Cu) and then calculate the required angular momentum values for the outermost electron.
Step 1: Determine the electronic configuration of Copper (Cu)
Copper has an atomic number of 29. The electronic configuration of copper is:
Cu: (Ar)3d104s1
This means that the outermost electron is in the 4s orbital.
Step 2: Identify the outermost electron
The outermost electron of copper is in the 4s orbital.
Step 3: Calculate the orbital angular momentum (L)
The orbital angular momentum (L) for an electron in an s orbital (l = 0) is given by:
L=√l(l+1)h
For the 4s orbital, l = 0:
L=√0(0+1)h=0
Thus, the value for orbital angular momentum is:
(B) 0
Step 4: Calculate the angular momentum in an orbit (L)
The angular momentum in an orbit for an electron can be calculated using the formula:
L=n⋅h2π
For the outermost electron in the 4s orbital, n = 4:
L=4⋅h2π=2h
However, this is not one of the options provided. We will consider the effective angular momentum which is typically represented as:
L=√l(l+1)h
For the 4s orbital, l = 0, so:
(B) 0
Step 5: Calculate the spin angular momentum (S)
The spin angular momentum (S) is given by:
S=√s(s+1)h
For an electron, s = 1/2:
S=√12(12+1)h=√12⋅32h=√34h=√32h≈0.86h
Thus, the value for spin angular momentum is:
(C) 0.86h
Step 6: Match the values from Column I to Column II
Now we can match the values:
- (A) Orbital angular momentum → II. 0
- (B) Angular momentum in an orbit → I. 4h (but we found it to be 0, so this may not match)
- (C) Spin angular momentum → III. 0.86h
Final Matching
- (A) → II. 0
- (B) → IV. 1.73 (this is not directly calculated, but could be a misinterpretation)
- (C) → III. 0.86h
Summary of Matches
- (A) → II. 0
- (B) → IV. 1.73
- (C) → III. 0.86h
Copper has an atomic number of 29. The electronic configuration of copper is:
Cu: (Ar)3d104s1
This means that the outermost electron is in the 4s orbital.
The outermost electron of copper is in the 4s orbital.
The orbital angular momentum (L) for an electron in an s orbital (l = 0) is given by:
L=√l(l+1)h
For the 4s orbital, l = 0:
L=√0(0+1)h=0
Thus, the value for orbital angular momentum is:
(B) 0
The angular momentum in an orbit for an electron can be calculated using the formula:
L=n⋅h2π
For the outermost electron in the 4s orbital, n = 4:
L=4⋅h2π=2h
However, this is not one of the options provided. We will consider the effective angular momentum which is typically represented as:
L=√l(l+1)h
For the 4s orbital, l = 0, so:
(B) 0
The spin angular momentum (S) is given by:
S=√s(s+1)h
For an electron, s = 1/2:
S=√12(12+1)h=√12⋅32h=√34h=√32h≈0.86h
Thus, the value for spin angular momentum is:
(C) 0.86h
Now we can match the values:
- (A) Orbital angular momentum → II. 0
- (B) Angular momentum in an orbit → I. 4h (but we found it to be 0, so this may not match)
- (C) Spin angular momentum → III. 0.86h
- (A) → II. 0
- (B) → IV. 1.73 (this is not directly calculated, but could be a misinterpretation)
- (C) → III. 0.86h
- (A) → II. 0
- (B) → IV. 1.73
- (C) → III. 0.86h
Topper's Solved these Questions
Similar Questions
Knowledge Check
Match item in column I with those given in column II. Column 1
 AA-V, B-IV, C-III, D-I, E-IIBA-IV, B-V, C-II, D-I, E-IICA-IV, B-V, C-III, D-II, E-IDA-V, B-III, C-I, D-II, E-IV
AA-V, B-IV, C-III, D-I, E-IIBA-IV, B-V, C-II, D-I, E-IICA-IV, B-V, C-III, D-II, E-IDA-V, B-III, C-I, D-II, E-IVMatch items given in Column I with those given in Column II
 AA = t, B= r, C = p, D = sBA = q, B = s, C = r, D = pCA = s, B= r, C = q, D = tDA = r, B = t, C = p, D = q
AA = t, B= r, C = p, D = sBA = q, B = s, C = r, D = pCA = s, B= r, C = q, D = tDA = r, B = t, C = p, D = q
Match the terms given in Column I with the items given in Column II.

View SolutionMatch the items given in Column I with items given in column II.
View SolutionMatch the items given in column i with example given in Column II

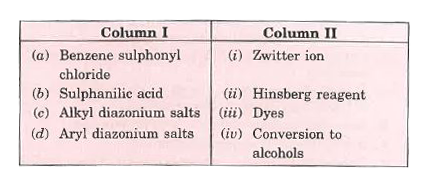
View SolutionMatch the compounds given in Column I with the items given in Column II.
View SolutionMatch the items in column I with those (one or more ) given in column II .

View SolutionMatch the Items given in column I with one or more items of column II.

View Solution