a circular ring carries a chargeQ1 the variation of electric field with distance x measured from centre along Axis for x>>R can be given as (R radius of ring)
E∝1x3
E∝x
E∝1x2
E∝1x
The correct Answer is:C
To solve the problem of how the electric field E varies with distance x from the center of a circular ring carrying a charge Q1 along its axis for x≫R (where R is the radius of the ring), we can follow these steps:
Step 1: Understand the Configuration
We have a circular ring of radius R with a total charge Q1 uniformly distributed along its circumference. We want to find the electric field at a point P located on the axis of the ring at a distance x from its center.
Step 2: Write the Electric Field Expression
The electric field E at a point along the axis of the ring can be expressed using the formula:
E=kQ1x(R2+x2)3/2
where k is Coulomb's constant, Q1 is the charge on the ring, R is the radius of the ring, and x is the distance from the center of the ring to the point where the electric field is being calculated.
Step 3: Analyze the Condition x≫R
Since we are interested in the case where x is much greater than R (i.e., x≫R), we can simplify the expression. In this case, R2 becomes negligible compared to x2:
R2+x2≈x2
Step 4: Simplify the Electric Field Expression
Substituting this approximation into the electric field expression gives:
E≈kQ1x(x2)3/2=kQ1xx3
Step 5: Further Simplification
Now, simplifying this expression:
E≈kQ1x2
Step 6: Conclusion
From the final expression, we see that the electric field E is inversely proportional to the square of the distance x:
E∝1x2
Thus, the variation of the electric field with distance x for x≫R is given by:
E∝1x2
Final Answer
The electric field E varies as 1x2 when x is much greater than the radius R of the ring.
---
We have a circular ring of radius R with a total charge Q1 uniformly distributed along its circumference. We want to find the electric field at a point P located on the axis of the ring at a distance x from its center.
The electric field E at a point along the axis of the ring can be expressed using the formula:
Since we are interested in the case where x is much greater than R (i.e., x≫R), we can simplify the expression. In this case, R2 becomes negligible compared to x2:
Substituting this approximation into the electric field expression gives:
Now, simplifying this expression:
From the final expression, we see that the electric field E is inversely proportional to the square of the distance x:
The electric field E varies as 1x2 when x is much greater than the radius R of the ring.
Topper's Solved these Questions
Similar Questions
Draw a plot showing variation of electric field with distance from the centre of a solid conducting sphere of radius R having a charge Q on its surface.
View SolutionAn isolated solid metal sphere of radius R is given an electric charge. The variation of the intensity of the electric field with the distance r from the centre of the sphere is best shown by
View Solution
Knowledge Check
A circular ring carries a uniformly distributed positive charge .The electric field(E) and potential (V) varies with distance (r ) from the centre of thr ring along its axis as
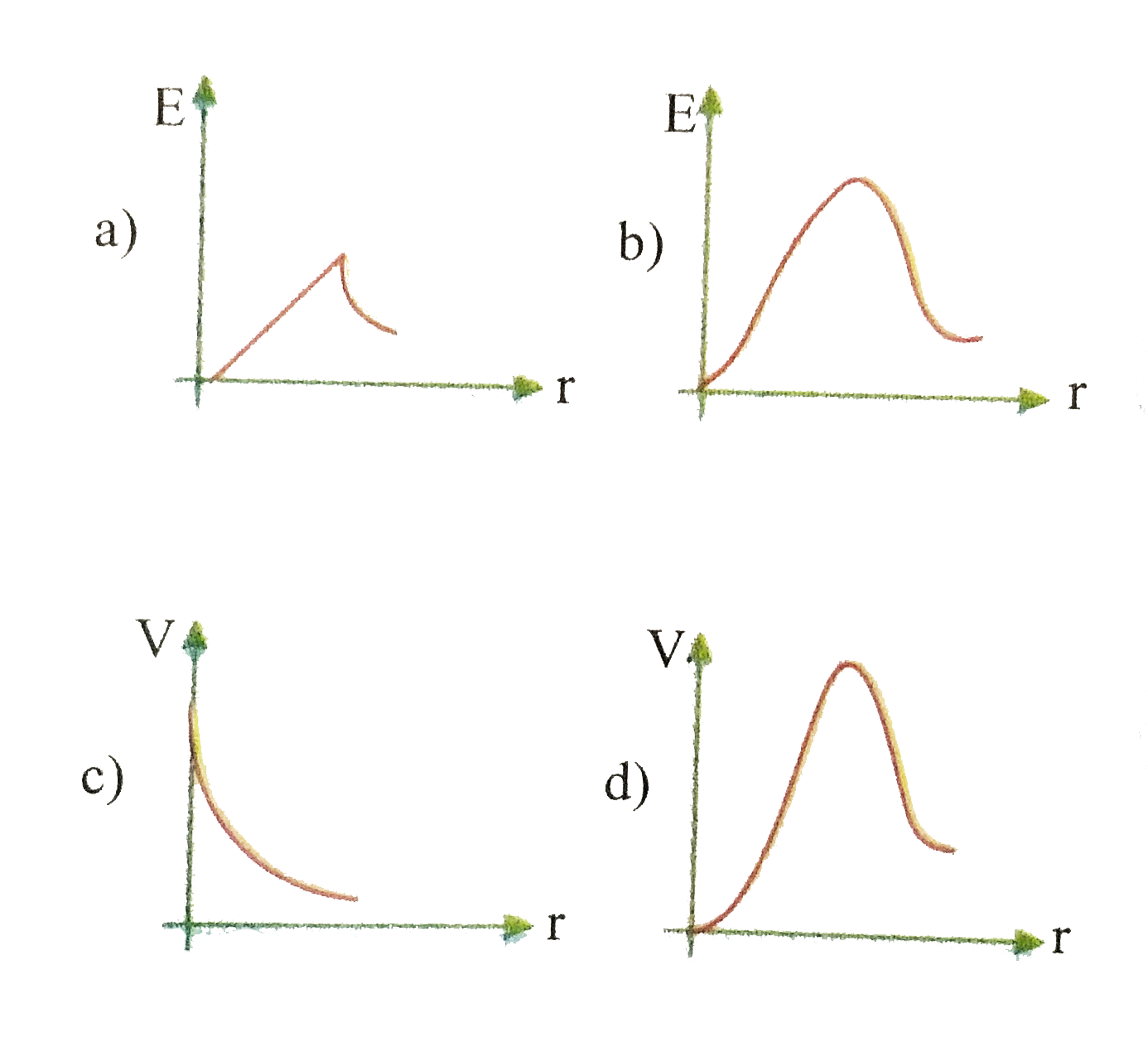 Ab andc are trueBa is trueCa,b,c are trueDa,b,c,d are true
Ab andc are trueBa is trueCa,b,c are trueDa,b,c,d are trueA small circular ring has a uniform charge distribution. On a far-off axial point distance x from the centre of the ring, the electric field is proportional to-
Ax−1Bx3/2Cx−2Dx5/4The maximum electric field intensity on the axis of a uniformly charged ring of charge q and radius R will be
A14πε0q3√3R2B14πε02q3R2C14πε02q3√3R2D14πε03q2√2R2
Potential on the axis of ring of radius 3R and at distance 4R from centre of ring is (charge on the ring is Q )
View SolutionA thin copper ring of radius a is charged with q units of electricity. Calculate the electric field at a point at a distance x from the centre and on the axis of the ring. Use the result to find the electirc field due to a charged disc of surface density of charge ε.
View SolutionA small circular ring has a uniform charge distribution.On a far-off axial point at a distantce x from the centre of the ring,the electric field is proportional to
View SolutionCharge is uniformly distributed around a ring of radius R = 4.60 cm, and the resulting electric field magnitude R is measured along the ring's central axis (perpendicular to the plane of the ring). At what distance from the ring's center is E maximum ?
View SolutionA point charge is projected along the axis of circular ring of charge Q and radius 10√2cm. The distance of the point charge from centre of ring, where acceleration of charged particle is maximum, will be
View Solution
