The average intensity of electromagnetic wave is (where symbols have their usual meanings)
ε0 ×E2_RMS×c
(B2_RMSμ0)×c
(12)×(ε0)×E2_RMS×c
Both (A) & (B)
The correct Answer is:D
To find the average intensity of an electromagnetic wave, we can follow these steps:
Step 1: Understand the concept of intensity
The intensity of an electromagnetic wave is defined as the power per unit area. It can be expressed mathematically as:
I=PA
where I is the intensity, P is the power, and A is the area over which the power is distributed.
Step 2: Relate intensity to the electric field
For electromagnetic waves, the intensity can also be expressed in terms of the electric field (E) and the speed of light (c). The average intensity I can be given by the formula:
I=12ϵ0cE2
where:
- ϵ0 is the permittivity of free space,
- c is the speed of light in vacuum,
- E is the RMS (Root Mean Square) value of the electric field.
Step 3: Substitute the expression for U
The energy density U of the electromagnetic wave can be expressed as:
U=12ϵ0E2
Thus, we can rewrite the intensity in terms of the energy density:
I=c⋅U
Step 4: Final expression for average intensity
Substituting the expression for U into the intensity equation, we get:
I=c⋅(12ϵ0E2)
This gives us the average intensity of the electromagnetic wave in terms of the electric field.
Conclusion
The average intensity of an electromagnetic wave is:
I=12ϵ0cE2
The intensity of an electromagnetic wave is defined as the power per unit area. It can be expressed mathematically as:
I=PA
where I is the intensity, P is the power, and A is the area over which the power is distributed.
For electromagnetic waves, the intensity can also be expressed in terms of the electric field (E) and the speed of light (c). The average intensity I can be given by the formula:
I=12ϵ0cE2
where:
- ϵ0 is the permittivity of free space,
- c is the speed of light in vacuum,
- E is the RMS (Root Mean Square) value of the electric field.
The energy density U of the electromagnetic wave can be expressed as:
U=12ϵ0E2
Thus, we can rewrite the intensity in terms of the energy density:
I=c⋅U
Substituting the expression for U into the intensity equation, we get:
I=c⋅(12ϵ0E2)
This gives us the average intensity of the electromagnetic wave in terms of the electric field.
The average intensity of an electromagnetic wave is:
I=12ϵ0cE2
Topper's Solved these Questions
Similar Questions
Knowledge Check
Intensity of electromagnetic wave will be
AI=cμ0B20/2BI=cε0B20/2CI=B20/cμ0DI=E20/2cε0Bohr magnetonis given by (symbols have their usual meanings)
A4πmeeh2B4πmeehCeh24πmeDeh4πmeThe correct combination is (symbols have their usual meanings)
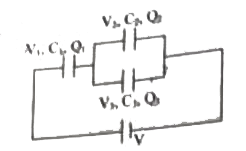 AQ1=Q2=Q3andV1=V2=V3=VBQ1=Q2+Q3andV=V1+V2+V3CQ1=Q2+Q3andV=V1+V2DQ2=Q3andV2=V3
AQ1=Q2=Q3andV1=V2=V3=VBQ1=Q2+Q3andV=V1+V2+V3CQ1=Q2+Q3andV=V1+V2DQ2=Q3andV2=V3
Select the incorrect relation. (Where symboles have their usual meanings):-
View SolutionChoose the correct arrangement. The symbols have their usual meanings
View SolutionMechanically work done is equal to (symbols have their usual meanings)
View SolutionMechanically work done is equal to (symbols have their usual meanings)
View SolutionThe correct form of Stroke's law is (symbols have their usual meanings)
View Solution
