xm∝Kp1n. Find the value of n
To solve the problem, we need to analyze the relationship given in the question:
xm∝K⋅P1n
Step 1: Understand the Variables
- x: mass of the adsorbate
- m: mass of the adsorbent
- K: a constant that depends on the nature of the adsorbent and adsorbate
- P: pressure
- n: a constant we need to determine
Step 2: Rewrite the Proportionality
We can express the proportionality as an equation by introducing a constant of proportionality C:
xm=C⋅K⋅P1n
Step 3: Analyze the Behavior at Different Pressures
1. Low Pressure:
- At low pressure, we can assume that the adsorption follows first-order kinetics. This means that the relationship simplifies to:
xm=K⋅P
Here, 1n=1 implies n=1.
2. High Pressure:
- At high pressure, the adsorption reaches saturation, and we can assume zero-order kinetics. This means:
xm=K
Here, P1n approaches P0 which implies n approaches infinity.
3. Moderate Pressure:
- In a moderate pressure range, n can take values greater than 1 and less than 10, indicating a transition between first-order and zero-order kinetics.
Step 4: Conclusion on the Value of n
From the analysis:
- At low pressure, n=1.
- At high pressure, n approaches infinity.
- In moderate conditions, n is typically greater than 1 but less than 10.
Thus, we conclude that n can take a range of values depending on the conditions, but it is always greater than 1.
Final Answer
The value of n is always greater than 1.
- x: mass of the adsorbate
- m: mass of the adsorbent
- K: a constant that depends on the nature of the adsorbent and adsorbate
- P: pressure
- n: a constant we need to determine
We can express the proportionality as an equation by introducing a constant of proportionality C:
1. Low Pressure:
- At low pressure, we can assume that the adsorption follows first-order kinetics. This means that the relationship simplifies to:
xm=K⋅P
Here, 1n=1 implies n=1.
- At high pressure, the adsorption reaches saturation, and we can assume zero-order kinetics. This means:
xm=K
Here, P1n approaches P0 which implies n approaches infinity.
- In a moderate pressure range, n can take values greater than 1 and less than 10, indicating a transition between first-order and zero-order kinetics.
From the analysis:
- At low pressure, n=1.
- At high pressure, n approaches infinity.
- In moderate conditions, n is typically greater than 1 but less than 10.
The value of n is always greater than 1.
Similar Questions
Knowledge Check
When am⋅an=amn,find the value of n(m-2) + m(n -2) is :
A0B1C-1Dnone of theseFreundlich adsorption isotherm is given by the expression xm=kP1/n . Then the slope of the line in the following plot is
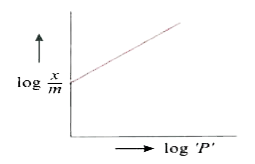 A√nB1/nCx/mDP
A√nB1/nCx/mDPFor Freundlich adsorption isotherm, xm=kp1/n , the value of n is
Aawlays greater tham 1Balways smaller than 1Calways equal to 1Dgreater than 1 at low temperature and smaller than 1 at high temperatute
If the G.M. of a and b is an+bnan−1+bn−1 then find the value of n.
View SolutionIf f(x)=xn & f′(1)=10, find the value of n.
View SolutionIf f(x ) = nn+1 , then find the value of f(n)+1f(n)
View SolutionIf x1,x2,………,xn are n values of a variable x such that ∑(xi−3)=170 and ∑(xi−6)=50.
Find the value of n and the mean of n values.
View SolutionFind the value of m so that (−3)m^(+1)×(−3)5=(−3)7
View Solution
