Consider the parametric forms x=(t)+(1t) and y=(t)−(1t) of a curve
Find dydxSolution in Malayalam
Step by step video & image solution for Consider the parametric forms x=(t)+(1/t) and y=(t)-(1/t) of a curve Find dy/dx by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
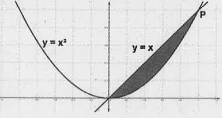
Consider the following figure
find the point of intersection (P) of the parabola and the line.
View SolutionThe curve described parametrically by x=t^(2)+t+1,y=t^(2)-t+1 represents :
View SolutionIf x=t+1t,y=t−1t, then dydx=
View SolutionIf x=a(t+t−1),y=a(t−t−1), then dydx=
View SolutionIf x=t+1t,y=t−1t, then: dydx=
View SolutionThe curve describe parametrically by x =t^(2) +t+1,y=t^(2) -t+1 represents
View SolutionThe curve described parametrically by : x=t^2+t+1,y=t^2-t+1 represents
View SolutionConsider the parametrically defined curve x(t)=1−sint,y=4−3cost then the equation in cartesian form is given by.
View SolutionThe curve described parametrically by x=t^(2)+t+1,y=t^(2)-t+1 represents
View SolutionIf x=a(t−1t),y=a(t+1t) then dydx=
View SolutionFind dydx of each of the functions expressed in parametric form:
x=1+logtt2,y=3+2logtt
View SolutionFind dydx:
x=t+1tandy=t−1t
View SolutionConsider the parametric forms x=(t)+(1t) and y=(t)−(1t) of a curve
Find the equation of the tangent at t=2.
View SolutionConsider the parametric forms x=(t)+(1t) and y=(t)−(1t) of a curve
Find the equation of the normal at t=2.
View SolutionIf x=(t+1t),y=(t−1t), then dydx=?
View Solution
