When a man holding spring balance in stationary lift then it's reading is 60kg. Now if lift starts descends with constant acceleration 1.8 m/s2 then what is the new reading of spring balance in newton (take g =10 m/s2)
The correct Answer is:B, D
To solve the problem step by step, we need to analyze the forces acting on the man in the lift when it is descending with constant acceleration.
Step 1: Understand the forces acting on the man in the lift
When the lift is stationary, the forces acting on the man are:
- The weight of the man (downward), which is given by mg.
- The normal force (upward), which is the reading of the spring balance N.
Since the lift is stationary, these forces are balanced:
N=mg
Step 2: Calculate the weight of the man
Given that the reading of the spring balance when stationary is 60kg, we can calculate the weight:
- m=60kg
- g=10m/s2
Thus, the weight W is:
W=mg=60kg×10m/s2=600N
Step 3: Analyze the situation when the lift is descending
When the lift descends with a constant acceleration a=1.8m/s2, we need to find the new reading of the spring balance N′.
In the accelerating frame of reference (the lift), the effective weight of the man is reduced because the lift is accelerating downwards. The net force acting on the man can be expressed as:
N′=mg−ma
Step 4: Substitute the values into the equation
Now, substituting the known values into the equation:
- m=60kg
- g=10m/s2
- a=1.8m/s2
We get:
N′=mg−ma
N′=60kg×10m/s2−60kg×1.8m/s2
N′=600N−108N
N′=492N
Final Answer
The new reading of the spring balance when the lift is descending with a constant acceleration of 1.8m/s2 is 492N.
---
When the lift is stationary, the forces acting on the man are:
- The weight of the man (downward), which is given by mg.
- The normal force (upward), which is the reading of the spring balance N.
N=mg
Given that the reading of the spring balance when stationary is 60kg, we can calculate the weight:
- m=60kg
- g=10m/s2
W=mg=60kg×10m/s2=600N
When the lift descends with a constant acceleration a=1.8m/s2, we need to find the new reading of the spring balance N′.
N′=mg−ma
Now, substituting the known values into the equation:
- m=60kg
- g=10m/s2
- a=1.8m/s2
N′=mg−ma
N′=60kg×10m/s2−60kg×1.8m/s2
N′=600N−108N
N′=492N
The new reading of the spring balance when the lift is descending with a constant acceleration of 1.8m/s2 is 492N.
Topper's Solved these Questions
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-A
Explore 80 Videos
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-B
Explore 40 Videos
- JEE MAINBook:JEE MAINS PREVIOUS YEARChapter:JEE MAINExercise:All Questions
Explore 473 Videos
- JEE MAIN 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2022Exercise:Question
Explore 492 Videos
Similar Questions
A person standing on a spring balance inside a stationary lift measures 60 kg. The weight of that person if the lift descends with uniform downward acceleration of 1.8m/s2 will be_N.
[g=10m/s2]
View SolutionA block of mass m is placed on weighing machine and it is also attached with a spring balance. The whole system is placed in a lift as shown in figure. Left is moving with constant acceleration 10m/s2 in upward direction. The reading of the weighing machine is 500 N and the reading of spring balance is 300 N. Find the actual mass of the block in kg. (Takr g=10m/s2)
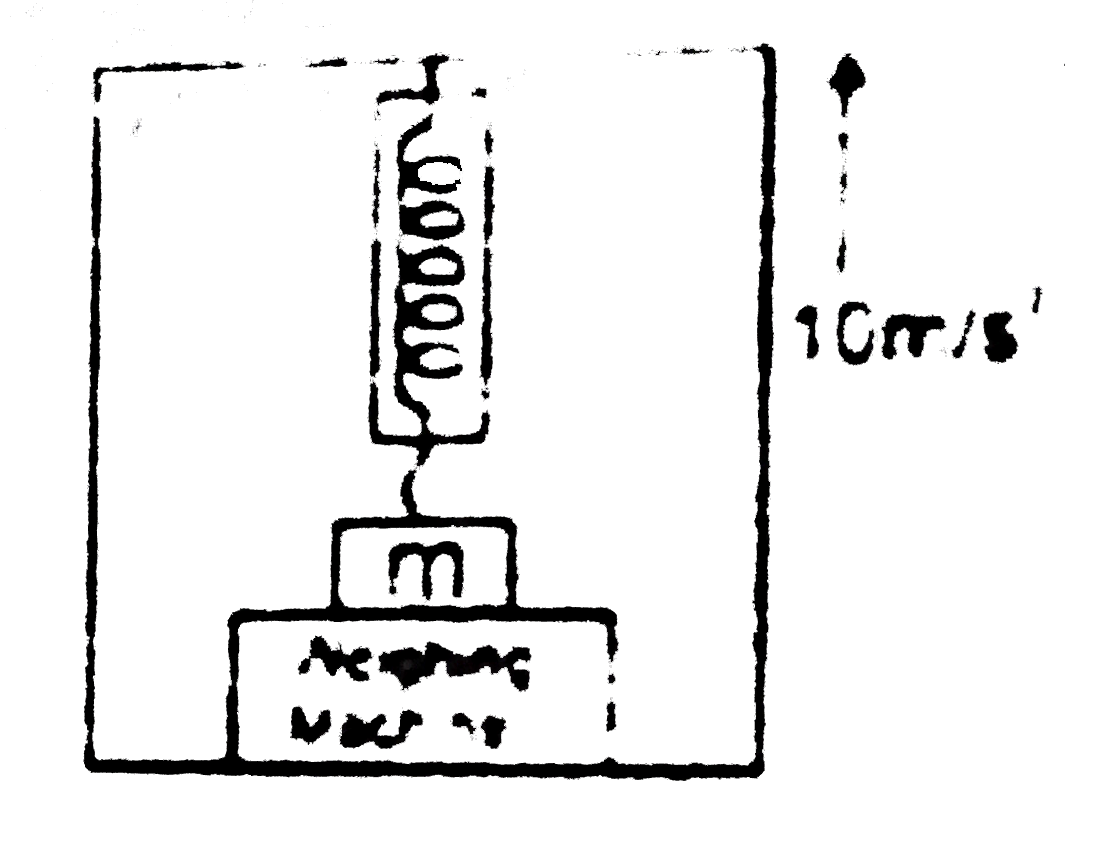
View Solution
Knowledge Check
Mass of a person sitting in a lift is 50 kg . If lift is coming down with a constant acceleration of 10 m/sec2 . Then the reading of spring balance will be (g=10m/sec2)
A0B1000 NC100 ND10 NA man is standing on a weighing machine placed in a lift. When stationary his weight is recorded as 40 kg . If the lift is accelerated upwards with an acceleration of 2m/s2 , then the weight recorded in the machine will be (g=10m/s2)
A32 kgB40 kgC42 kgD48 kgThe mass of lift is 500 kg. When it ascends with an acceleration of 2m/s2,the tension in the cable will be [g=10m/s2]
A6000 NB5000 NC4000 ND50 N
A spring balance is attached to the ceiling of a lift. A man hangs his bag on the spring and the spring reads 49 N, when the lift is stationary. If the moves downwards with an acceleration of 5 ms−2, then reading of the spring balance will be
View SolutionA body of mass 2 kg is hung on a spring balance mounted vertically in a lift. If the lift descends with an acceleration equal to the acceleration due to gravity ‘ g ’, the reading on the spring balance will be
View SolutionA boy whose mass is 50 kg stands on a spring balance inside a lift. The lift starts to ascent with an acceleration of 2ms−2. The reading of the machine or balance (g=10ms−2) is
View SolutionReading of spring balance S1 and S2 (Pulley are ideal)
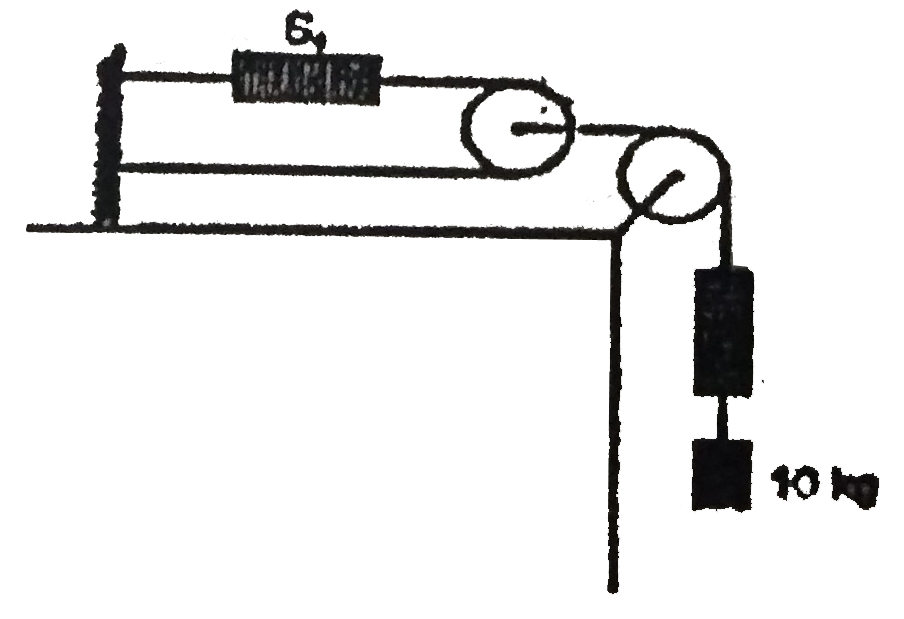
View SolutionA lift of mass 100 kg is moving upwards with an acceleration of 1 m/s2. The tension developed in the string, which is connected to lift is (g=9.8m/s2)
View Solution