□ABCD is a parallelogram. (A1) and B1 are midpoints of the sides ¯¯¯¯¯¯BC and ¯¯¯¯¯¯AD respectively. If −−→AA1+−−→AB1=λ−−→AC then λ = …………Solution in Gujrati
12
1
32
2
The correct Answer is:C
Step by step video, text & image solution for squareABCD is a parallelogram. (A_(1)) and B_(1) are midpoints of the sides bar(BC) and bar(AD) respectively. If vec("AA")_(1)+vec(AB)_(1)=lambda vec(AC) then lambda = ………… by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- VECTOR ALGEBRA BOOK - KUMAR PRAKASHANCHAPTER - VECTOR ALGEBRA EXERCISE - Practice Paper - 10 (Section-A)
6 Videos
- VECTOR ALGEBRA BOOK - KUMAR PRAKASHANCHAPTER - VECTOR ALGEBRA EXERCISE - Practice Paper - 10 (Section-B)
4 Videos
- VECTOR ALGEBRA BOOK - KUMAR PRAKASHANCHAPTER - VECTOR ALGEBRA EXERCISE - Solutions of NCERT Exemplar Problems (True/False)
5 Videos
- THREE DIMENSIONAL GEOMETRYBOOK - KUMAR PRAKASHANCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - PRACTICE PAPER -11
16 Videos
Similar Questions
ˆi⋅(ˆj׈k)+ˆj⋅(ˆi׈k)+ˆk⋅(ˆi׈j) =……………
View SolutionIf a unit vector →a makes an angle π3 with ˆi,π4 sith ˆj and an acute angle θ with ˆk, then find the component of →a.
View SolutionIf |¯a|=∣∣¯b∣∣, then necessarily it implies ¯a=±¯b.
View SolutionFind the direction cosines of a line which makes equal angles with the coordinate axes.
View SolutionIn /_ABC if D.E.F are the midpoints of sides BC,CA, AB respectively and vec AD+(2)/(3)vec BE+(1)/(3)vec CF=kvec AC then k=
View SolutionThe base vectors vec a_ (1), vec a_ (2), vec a_ (3) are given in terms vectors vec b_ (1), vec b_ (2), vec b_ (3) vec a_ (1) = 2vec b_ (1) + 3vec b_ (1) -vec b_ (1), vec a_ (2) = vec a_ (1) -2vec b_ (2) + 2vec b_ (3), vec a_ (3) = - 2vec b_ ( 1) + vec b_ (2) -2vec b_ (3) if vec F_ (1) = 3vec b_ (1) -vec b_ (2) + 2vec b_ (3), Express vec F in terms of vec a_ (1) , vec a_ (2), vec a_ (3)
View SolutionLet B_(1),C_(1) and D_(1) are points on AB,AC and AD of the parallelogram ABCD , such that AB_(1)=k_(1)vec AC,AC_(1)=k_(2)vec AC and vec AD_(1)=k_(2)vec AD where k_(1),k_(2) and k_(3) are scalar.
View SolutionIf vec a and vec b are two vectors and lambda>0, then the least value of (1+lambda)|vec a|^(2)+(1+(1)/(lambda))|vec b|^(2) is
View SolutionIf D is the mid point of side BC of a triangle ABC such that →AB+→AC=λ →AD, write the value of λ.
View SolutionABCD समांतर चतुर्भुज है। E,AB का मध्य बिंदु है और F,BC का मध्य बिंदु है। यदि −−→AB=→a और −−→AD=→b तो −−→EF का मान बताओ।
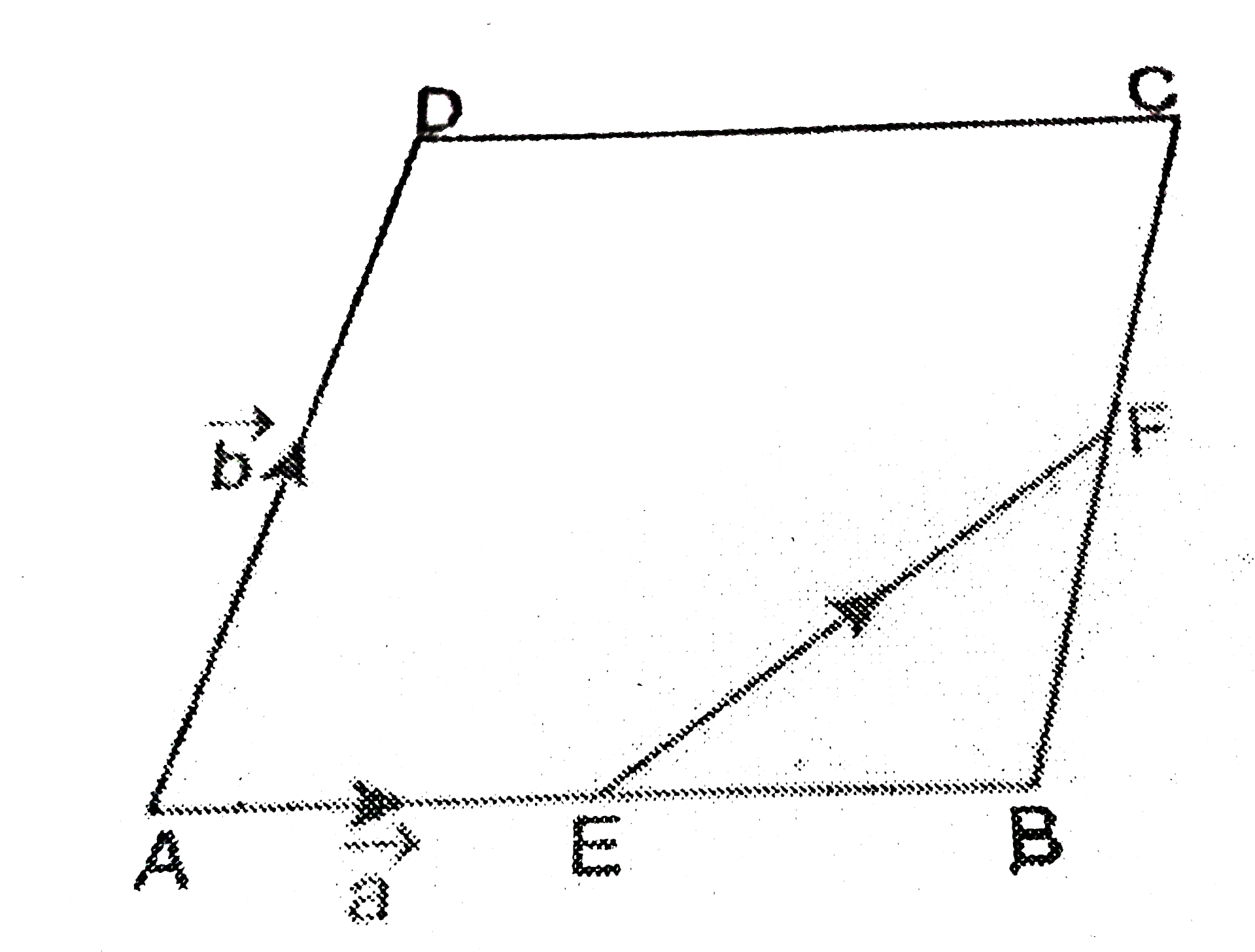
View SolutionABCD a parallelogram, and A1andB1 are the midpoints of sides BC and CD, respectively. If −→aA1 +−−→AB1=λ−−→AC, then λ is equal to
View SolutionIf D, E are the midpoints of AB, AC of Δ ABC and →DE=λ→BC then λ =
View SolutionLet D, E, F are the midpoints of the sides ¯¯¯¯¯¯BC,¯¯¯¯¯¯CA and ¯¯¯¯¯¯AB of the triangle ABC. Prove that −−→AD+−−→BE+−−→CF=→0.
View Solution¯¯¯¯¯¯AC and ¯¯¯¯¯¯BD are the diagonals of the parallelogram ABCD. Prove that,
−−→AC+−−→BD=2−−→BC and −−→AC−−−→BD=2−−→AB
View SolutionABCD is a parallelogram and A1 and B1 are the midpoints of sides BC and CD respectively. If −−→AA1+−−→AB1=λ2−−→AC, then λ is equal to -
View Solution
