A random variable X takes four values with probabilities 1+3x4,(1−x),1+2x4 and 1−4x4. Find the set of values of x for the variable X to have probability distribution.
The correct Answer is:−13≤x≤14
Topper's Solved these Questions
Similar Questions
A random variable X takes values −1,0,1,2 with probabilities 1+3p4,1−p4,1+2p4,−14p4 respectively, where p varies over R. Then the minimum and maximum values of the mean of X are respectively
View SolutionA random variable X takes values 0, 1, 2, 3,… with probability proportional to (x+1)(15)x.
P(X≥2) equals
View Solution
Knowledge Check
A random variable X takes values 0, 1, 2, 3,… with probability proportional to (x+1)(15)x.
P(X≥2) equalsA1125B1325C11125D13125If the random variable X takes the values x1,x2,x3……x10 with probabilities P(X=x1)=ki then the value of k is
A(1/10)B(1/4)C(1/55)D(7/12)
A random variable X has the follwing probability distribution:
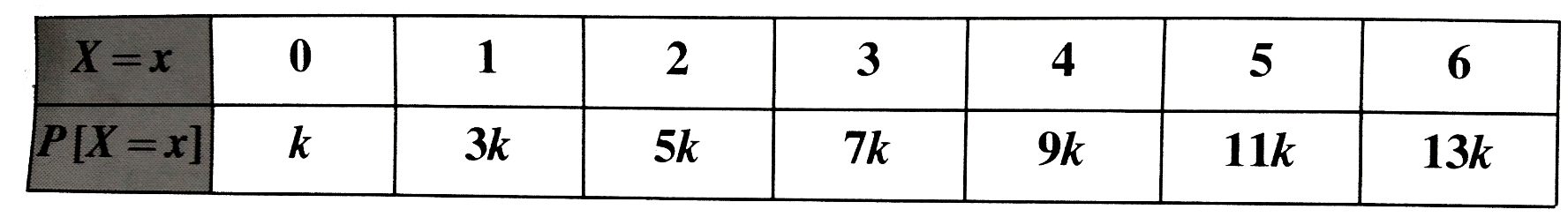
Find P(0<X<4)
View SolutionFind the value of k for probability distribution of a random variable X is give by:

View SolutionGiven a random variable that takes values X={0,1,2} and has a probability distribution, P(0)=14,P(1)=12,P(2)=14. Then find the variance of X.
View SolutionA random variable X has the following probability distribution: [X=x0123P(X=x)181214k]
View SolutionA random variable Xtakes the values0,1and2.If P(X=1)=P(X=2)andP(X=0)=0.4,then the mean value of the random variableX is
View SolutionIf a random variable x takes values 0,1,2 and 3 and the probability distribution is given by P(x)=kx, then find k and hence find the expected value of the distribution.
View Solution
