When a stopper is pulled from a filled wash basin, the water drains out while circulating like a small whirl pool. The angular velocity of a fluid element about a vetical axis through the orifice appears to be greater near the orifice. The angular velocity of fluid element varies
Inversely as the square of its distance from the axis through the orifice
inversely as the distance from the axis through the orifice.
directly as the square of its distance from the axis through the orifice
directly as the distance from the axis through the orifice.
The correct Answer is:A
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Exercise-II
109 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE
99 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Problems
103 Videos
- SURFACE TENSIONBOOK - AAKASH SERIESCHAPTER - SURFACE TENSIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS )
8 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) PRACTICE SHEET (ADVANCED) Integer/Subjective Type Questions
2 Videos
Similar Questions
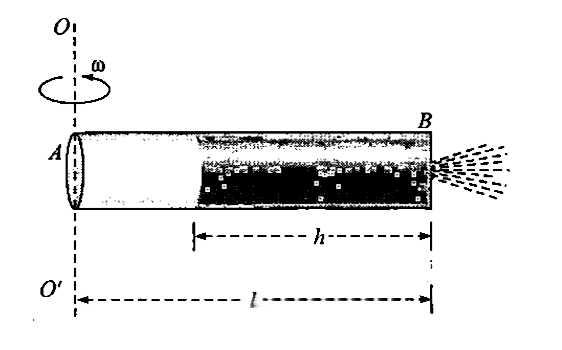
A horizontally oriented tube AB of length l rotates with a constant angular velocity ω about a stationary vertical axis OO′ passing through the end A (figure). The tube is filled with an ideal fluid. The end A of the tube is open, the closed end B has a very small orifice. Find the velocity of the fluid relative to the tube as a function of the column "height" h.

View SolutionA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.
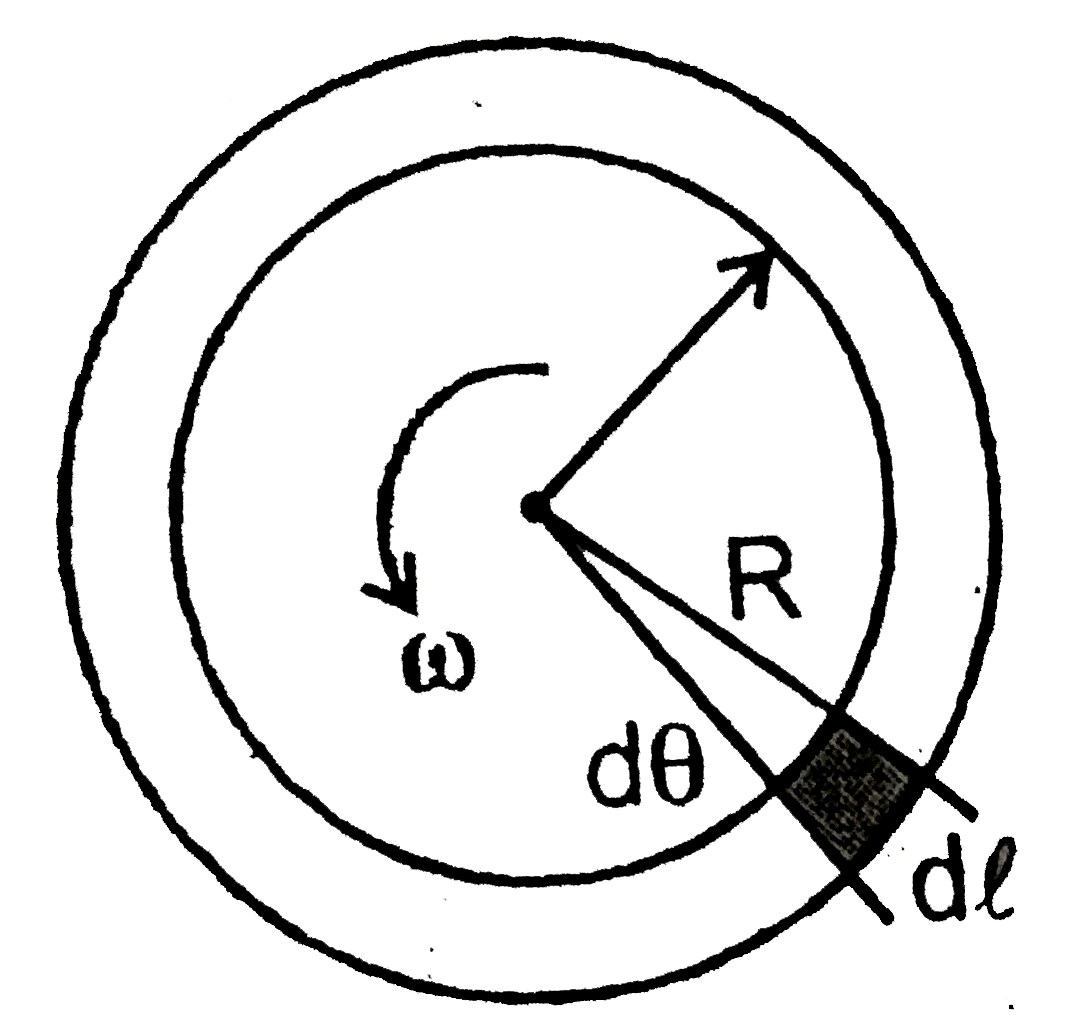
If T is the tension in the ring, then
View Solution
Knowledge Check
A ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

The centripetal force acting on the element isA(a.ρdlω2R)BR2dθ.ω2C12aρdlω2RDzeroA ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If T is the tension in the ring, thenAT=aρR2ω22BT=aρR2ω2Ca2ρω2DT=2aρR2ω2A ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will beAT/2BTC2TD4T
A ring of radius R is made of a thin wire of material of density ρ having cross section area a. The ring rotates with angular velocity ω about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length dl of angular width dθ is shown in the figure.

If for a given mass of the ring and angular velocity, the radius R of the ring is increased to 2R, the new tension will be
View SolutionA horizontal oriented tube AB of length l rotates with a constant angular velocity ω about a stationary vertical axis OO' passing thorugh the end A figure. The tube is filled with an ideal fluid. The end A of the tube is open, the closed end B has a very small orifice. find the velocity of the fluid relative to the tube as a function of the column height h.
View SolutionA small body of mass m=0.30kg starts sliding down from the top of a smooth sphere of radius R=1.00m. The sphere rotates with a constant angular velocity ω=6.0rad/s about a vertical axis passing through its centre. Find the centrifugal force of inertia and the Coriolis force at the moment when the body breaks off the surface of the sphere in the reference frame fixed to the sphere.
View SolutionA horizontally oriented tube AB of length l rotates with a constant angular velocity ω about a stationary vertical axis OO′ passing through the end A (figure). The tube is filled with an ideal fluid. The end A of the tube is open, the closed end B has a very small orifice. Find the velocity of the fluid relative to the tube as a function of the column "height" h.

View Solution_S01_079_Q01.png)
A horizontal oriented tube AB of length 5m rotates with a constant angular velocity 0.5 rad/s about a stationary vertical axis OO' passing through the end A. the tube is filled with ideal fluid. The end of the tube is open, the closed end B has a very small orifice. The velocity with which the liquid comes out from the hole (in m/s) is
View Solution
