A spherical balloon of radius 5 subtends an angle 60∘ at a point on the horzontal. If the angle of elevation of its centre is 30∘, the height of the centre of the balloon is Solution in Telugu
5√2 units
10 units
15 units
20 units
The correct Answer is:A
Step by step video, text & image solution for A spherical balloon of radius 5 subtends an angle 60^(@) at a point on the horzontal. If the angle of elevation of its centre is 30^(@), the height of the centre of the balloon is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - HEIGHTS AND DISTANCESEXERCISE - PRACTICE SHEET Exercise-I (Level-II) (Straight Objective Type Questions)13videos
HEIGHTS AND DISTANCES
- BOOK - AAKASH SERIESCHAPTER - HEIGHTS AND DISTANCESEXERCISE - LECTURE SHEET Exercise-I (Straight Objective Type Questions)27videos
HEIGHTS AND DISTANCES
- BOOK - AAKASH SERIESCHAPTER - FUNCTIONS EXERCISE - PRACTICE EXERCISE50videos
FUNCTIONS
- BOOK - AAKASH SERIESCHAPTER - HYPERBOLIC FUNCTIONS EXERCISE - PRACTICE SHEET EXERCISE-II (STRAIGHT OBJECTIVE TYPE QUESTIONS)18videos
HYPERBOLIC FUNCTIONS
Similar Questions
The angle of elevation of the top of a tower standing on a harzontal plane from the two points lying on a line passing through the foot of the tower at distances a and b respectively are complementary angles. If the line joining the two points sutends an angle θ at the top of the tower, then sinθ is
05:03View SolutionA tower of height 30 metres casts a shadow of length 40 meters at a certain instant. When the sun's elevation increases by Tan−117, the length of the shadow cast by the tower, in meters is
04:26View SolutionIf f(x)=3x−75x−3 then (fof)(x)=
04:09View SolutionThe domain of cosh−1(2x) is
07:56View SolutionA round balloon of radius r subtends an angle alpha at the eye of the observer while the angle of elevation of its centre is beta Prove that the height of the centre of the balloon is r sin beta cos ec[(alpha)/(2)]
03:59View SolutionA round balloon of radius r subtends an angle alpha at the eye of the observer while the angle of elevation of its centre is beta. Prove that the height of the centre of the balloon is r sin(beta)cos ec(alpha)/(2)
03:33View SolutionA round balloon of radius r subtends an angle at the eye of the observer while the angle of elevation of its centre is β. Prove that the height of the centre of the balloon is rsinβcosecα2.
02:27View SolutionA spherical balloon of radius 50 cm, subtends an angle of 60∘ at a man's eye when the elevation of its centre if 45∘. Then the height of the centre of the ballon is
05:37View SolutionA spherical baloon of radius 50 cm, subtends an angle of 60∘ at a man's eye when the evevatin of its centre is 45∘. Then the height of the centre of the balloon is
View SolutionA spherical ballon of radius r subtends angle 60∘ at the eye of an observer. If the angle of elevation of its centre is 60∘ and h is the height of the centre of the ballon, then which one of the following is correct ?
05:13View SolutionA round balloon of radius 'r' subtends an angle α at the eye of the observer, while the angle of elevation of its centre is β. Find the height of the centre of balloon.
04:41View SolutionA round balloon of radius r subtends an angle a at the eye of the observer while the angle of elevation of its centre is p. Prove that the height of the centre of the balloon B is r sin β cosec α2.
03:50View SolutionA spherical balloon subtends an angle 2α at a man's eye and the elevation of its centre is β. If θ is the elevation of the hightest point of the balloon at A then tanθ is equal to
04:55View SolutionA round balloon of radius r subtends an angle at the eye of the observer while the angle of elevation of its centre is β. Prove that the height of the centre of the balloon is rsinβcosecα2.
02:47View SolutionA spherical balloon of radius r subtends an angle θ at the eye of an observer. If the angle of elevation of its centre is ϕ, find the height of the centre of the balloon.
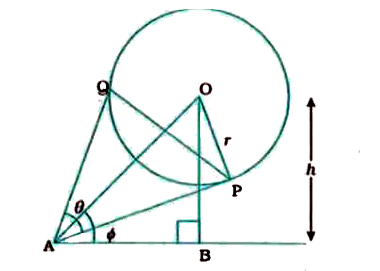 05:28View Solution
05:28View SolutionA spherical balloon of radius 5 subtends an angle 60∘ at a point on the horzontal. If the angle of elevation of its centre is 30∘, the height of the centre of the balloon is
02:27View Solution

