The number of octahedral voids per lattice site in a lattice is _____ (Rounded off to the nearest integer)
The correct Answer is:1
To determine the number of octahedral voids per lattice site in a lattice, particularly in a face-centered cubic (FCC) structure, we can follow these steps:
1. Identify the Lattice Points in FCC Structure:
- In a face-centered cubic (FCC) lattice, there are atoms located at the corners and the face centers of the unit cell.
- There are 8 corner atoms, each contributing 18 of an atom to the unit cell. Therefore, the total contribution from the corners is:
8×18=1 atom
- There are 6 face-centered atoms, each contributing 12 of an atom to the unit cell. Therefore, the total contribution from the face centers is:
6×12=3 atoms
- Thus, the total number of lattice points (atoms) in an FCC unit cell is:
1+3=4 atoms
2. Determine the Number of Octahedral Voids:
- In an FCC structure, the octahedral voids are located at the body center and at the edge centers.
- There is 1 body center in the unit cell, contributing 1 octahedral void.
- There are 12 edges in the FCC unit cell, and each edge contributes 14 of an octahedral void (since each edge is shared by 4 unit cells). Therefore, the total contribution from the edges is:
12×14=3 octahedral voids
- Thus, the total number of octahedral voids in an FCC unit cell is:
1+3=4 octahedral voids
3. Calculate the Ratio of Octahedral Voids to Lattice Points:
- Now, we can find the ratio of octahedral voids to lattice points:
Ratio=Number of Octahedral VoidsNumber of Lattice Points=44=1
Final Answer
The number of octahedral voids per lattice site in a lattice is 1 (rounded off to the nearest integer).
- In a face-centered cubic (FCC) lattice, there are atoms located at the corners and the face centers of the unit cell.
- There are 8 corner atoms, each contributing 18 of an atom to the unit cell. Therefore, the total contribution from the corners is:
8×18=1 atom
- There are 6 face-centered atoms, each contributing 12 of an atom to the unit cell. Therefore, the total contribution from the face centers is:
6×12=3 atoms
- Thus, the total number of lattice points (atoms) in an FCC unit cell is:
1+3=4 atoms
- In an FCC structure, the octahedral voids are located at the body center and at the edge centers.
- There is 1 body center in the unit cell, contributing 1 octahedral void.
- There are 12 edges in the FCC unit cell, and each edge contributes 14 of an octahedral void (since each edge is shared by 4 unit cells). Therefore, the total contribution from the edges is:
12×14=3 octahedral voids
- Thus, the total number of octahedral voids in an FCC unit cell is:
1+3=4 octahedral voids
- Now, we can find the ratio of octahedral voids to lattice points:
Ratio=Number of Octahedral VoidsNumber of Lattice Points=44=1
The number of octahedral voids per lattice site in a lattice is 1 (rounded off to the nearest integer).
Topper's Solved these Questions
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - CHEMISTRY (SECTION A)40videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - CHEMISTRY (SECTION B)20videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - CHEMISTRY (SECTION-A)20videos
JEE MAIN 2021
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - CHEMISTRY150videos
JEE MAIN
- BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question561videos
JEE MAIN 2022
Similar Questions
Knowledge Check
- Question 1 - Select One
The number of octahedral sites per sphere in a fcc structure is :
A8B4C2D1 - Question 2 - Select One
The number of octahedral sites per sphere in a fcc structure is :
A8B4C2D1 - Question 3 - Select One
The number of octahedral sites per sphere in fcc structure is
A8B4C2D1
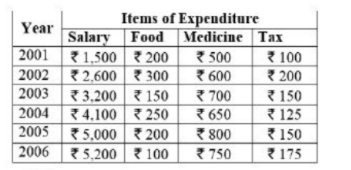
Based on the given table. the percentage salary increase per year during the period 2001-2006 was _____ (round off to the nearest integer).
 View Solution
View SolutionThe number of octahedral sites per sphere in fcc structure is
View SolutionThe number of octahedral sites per sphere in fcc structure is
View SolutionThe number of octahedral voids present in a lattice is _A_. The number of closed packed particles, the number of tetrahedral voids generated is _B_ the number of closed packed particles
View Solution4152 rounded off to the nearest thousand is
View Solution

