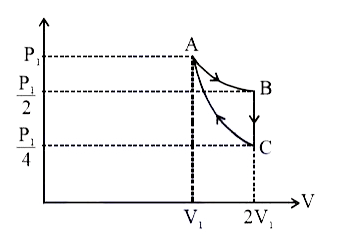
If one mole of an ideal gas at (P1,V1) is allowed to expand reversibly and isothermally (A to B) its pressure is reduced to one-half of the original pressure (see figure). This is followed by a constant volume cooling till its pressure is reduced to one-fourth of the initial value (B→C) . Then it is restored to its initial state by a reversible adiabatic compression (C to A). The net workdone by the gas is equal to :
RT(ln2−12(γ−1))
−RT2(γ−1)
0
RT ln 2
The correct Answer is:A
Step by step video & image solution for If one mole of an ideal gas at (P_1, V_1) is allowed to expand reversibly and isothermally (A to B) its pressure is reduced to one-half of the original pressure (see figure). This is followed by a constant volume cooling till its pressure is reduced to one-fourth of the initial value (BrarrC) . Then it is restored to its initial state by a reversible adiabatic compression (C to A). The net workdone by the gas is equal to : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - (SECTION - B)
10 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - PHYSICS (SECTION A)
60 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - PHYSICS (SECTION-B)
10 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
1 mole of an ideal gas is allowed to expand isothermally at 27∘C till its volume is tripled . If the expansion iscarried out reversibly then the ΔSuniverse will be?
View SolutionIf the pressure of 2 litres of an ideal gas is 4 atm then what will be the pressure of the gas if the volume is reduced to one- fourth of its present value?
View Solution
Knowledge Check
A gas is suddenly compressed to one fourth of its original volume. What will be its final pressure, if its initial pressure is p
ALess then pBMoer then PCpDEither(a) or ©10 moles of an ideal gas are compressed isothermally and reversibly at 200°C from a pressure of 1 atm to a pressure of S atm. Then
AΔU=0,ΔH=0BΔU≠0,ΔH=0CΔU=0,ΔH≠0DΔU≠0,ΔH≠0If n moles of an ideal gas are expanded is isothermally and reversibly from an initial state in which it has pressure p1 and volume V1 to the final state of volume V2 and pressure P2, then
AΔSsys=−2.303nRlog(P1p2)BΔSsys=2.303Rnlog(P1p2)CΔSsys=−2.303Rnlog(P1p2)DΔSsys=2.303nRlog(P1p2)
Two moles of a certain ideal gas at 300K is cooled at constant volume so that the pressure is reduced to half the original value. Now the gas is heated at constant pressure so that its temperature becomes equal to the initial temperature. Find the total amount of heat absorbed by the gas in the process.
View SolutionOne mole of an ideal gas is allowed to expand isothermally and reversibly at 27∘C until its volume is tripled.Find ΔSsystem in JK−1mol−1
View SolutionOne gram mole of an ideal gas at N.T.P is first expanded isothermally to twice the origional volume. It is then compressed at constant volume, till its pressure is raised to the original value. Calculate the total amount of work done. Given R=8.3mole_1K−1.
View SolutionA sample of an ideal gas in a cyclinder is compressed adiabatically to 13rd of its volume. Will final pressure be more or less than 3× the initial pressure?
View SolutionIn the isothermal reversible compression of 52.0m mol of a perfect gas at 260K, the volume of the gas is reduced to one−third of its initial value. Calculate w of this provess y.
View Solution
