The number of points at which the function f(x)=max{a−x,a+x,b},−∞<x<∞,0<a<b cannot be differentiable Solution in Bengali
0
1
2
3
Step by step video & image solution for The number of points at which the function f(x)=max {a-x, a+x, b}, -oo lt x lt oo, 0 lt a lt b cannot be differentiable by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In a certain town, 60% of the families own a car, 30% own a house and 20% own both a car and a house. If a family is randomly chosen, what is the probability that this family owns a car or a house but not both?
View SolutionTransforming to parallel axes through a point (p, q). The equation
2x2+3xy+4y2+x+18y+25=0 becomes 2x2+3xy+4y2=1. Then
View SolutionLet f:[0,1]→R (the set of all real numbers) be a function. Suppose the function f is twice differentiable, f(0)=f(1)=0 and satisfies f''(x)−2f'(x)+f(x)≥ex,x∈[0,1]Which of the following is true for 0<x<1 ? (A) 0<f(x)<∞ (B) −12<f(x)<12 (C) −14<f(x)<1 (D) −∞<f(x)<0
View SolutionThe number of points at which the function f(x)=max{a-x,a+x,b},-oo
View SolutionIf f(x)=x3+bx3+cx+dand0<b2<cthen in(−∞,∞)
View Solutionबिंदु x=1, पर फलन
f(x)={x3−11<x<∞x−1−∞<x≤1 है-
View SolutionConsider the function f:(−∞,∞)→(−∞,∞) defined by f(x)=x2−ax+1x2+ax+1;0<a<2. Which of the following is true?
View SolutionFind the inverse of each of the following functions :
f(x)=⎧⎪⎨⎪⎩x,−∞<x<1x2,1≤x≤42x,4<x<∞
View Solutionबिन्दुओ की संख्या जिन पर फलन f(x)=max{a−x,a+x,b},−∞<x<∞,0<a<b अवकलनीय नहीं है-
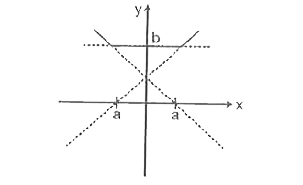
View Solutionफलन f(x)=sec(π4cos2x),−∞<x<∞ का परिसर (रेंज) है
View SolutionIf f(x)=x^(3)+bx^(2)+cx+d and 0 lt b^(2) lt c , then in (-oo, oo), f(x) :
View SolutionIf 0<a<c,0<b<c then ∫∞0ax−bxcxdx=
View Solutionमाना फलन f(x)=⎧⎪ ⎪⎨⎪ ⎪⎩sgn(x)+x−∞<x<0−1+sinx0≤x<π2cosxπ2≤x<∞ है। बिंदु x=0 तथा x=π2 पर संततता तथा अवकलनीयता की जाँच कीजिये।
View Solutionमाना f(x)=⎧⎪ ⎪ ⎪⎨⎪ ⎪ ⎪⎩xtanx−∞<x<02x=0ln(1+2x)x0<x<∞ तथा g(x)=⎧⎪⎨⎪⎩x+4−∞<x<1x2−5x+111≤x<2x−32≤x<∞ तो निन्न कौन (से) कथन सही है?
View SolutionLet f:[0,1]→R (the set of all real numbers) be a function. Suppose the function f is twice differentiable, f(0)=f(1)=0 and satisfies f''(x)−2f'(x)+f(x)≥ex,x∈[0,1] Which of the following is true for 0<x<1 ? (A) 0<f(x)<∞ (B) −12<f(x)<12 (C) −14<f(x)<1 (D) −∞<f(x)<0
View SolutionConsider the function f:(−∞,∞)→(−∞,∞) defined by f(x)=x2−ax+1x2+ax+1;0<a<2. Which of the following is true ?
View Solution
