There are two incline planes AO and OB inclined at and respectively with the horizontal as shown in the figure. When a man moves on the incline plane AO with speed 10 m/s. he oberves that the rain drops are falling with the vertical. When the man moves on the inclined plane OB with speed 20 m/s. he observes that the raindrops are falling vertically downward. Then actual speed of rain is
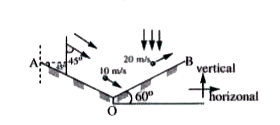
None of these
The correct Answer is:C
Topper's Solved these Questions
- KINEMATICSBOOK - AAKASH SERIESCHAPTER - KINEMATICSEXERCISE - LECTURE SHEET (EXERCISE-VII) (Relative motion) (More than One correct answer Type Questions)
3 Videos
- KINEMATICSBOOK - AAKASH SERIESCHAPTER - KINEMATICSEXERCISE - LECTURE SHEET (EXERCISE-VII) (Relative motion) (Linked Comprehension Type Questions)
5 Videos
- KINEMATICSBOOK - AAKASH SERIESCHAPTER - KINEMATICSEXERCISE - LECTURE SHEET (EXERCISE-VI) (Horizontal Projectile ) (Integer Type Questions)
1 Videos
- HORIZONTAL CIRCULAR MOTIONBOOK - AAKASH SERIESCHAPTER - HORIZONTAL CIRCULAR MOTIONEXERCISE - ADDITIONAL PRACTICE EXERCISE (PRACTICE SHEET (ADVANCED) Integer Type Questions)
3 Videos
- KINETIC THEORY OF GASESBOOK - AAKASH SERIESCHAPTER - KINETIC THEORY OF GASESEXERCISE - Practice Exercise
15 Videos
Similar Questions
There are two incline planes AO and OB inclined at 45∘ and 60∘ respectively with the horizontal as shown in the figure. When a man moves on the inclined plane AO he is oberves that the rain drops are falling 45∘ with the vertical. When the man moves on the inclined plane OB, he observes that the raindrops are falling vertically downward with the horizontal. Then actual speed of rain is
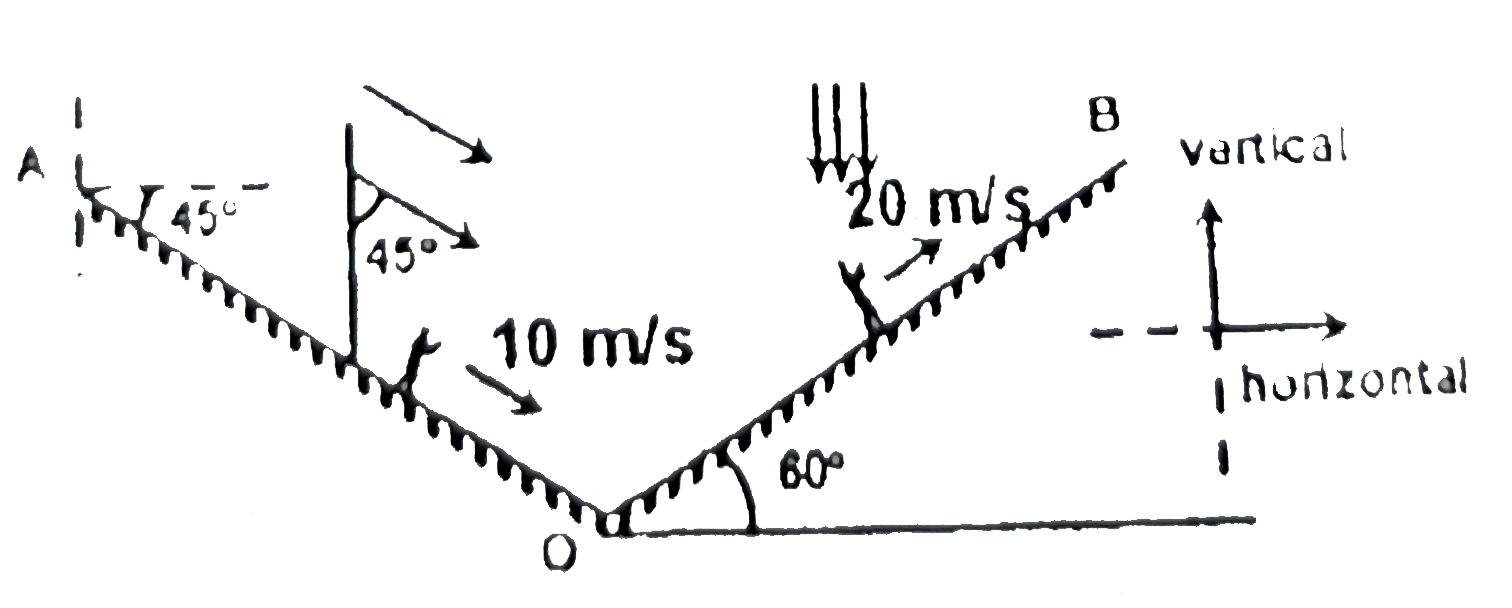
View SolutionWhen a boy is stationary, he observes that rain drops are falling at 30∘ to vertical direction. When he starts running with speed 10ms, he observed that rain drops are falling vertically downwards. Calculate the actual velocity of rain drops.
View Solution
Knowledge Check
A man standing on a inclined plain observes rain is falling verticaly. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The actual velocity of rain isA3m/sB3√3m/sC4m/sDnone of theseWhen a man moves down the inclined plane with a constant speed 5ms−1 which makes an angle of 37∘ with the horizontal, he finds that the rain is falling vertically downward. When he moves up the same inclined plane with the same speed, he finds that the rain makes an angle θ=tan−1(78) with the horizontal. The speed of the rain is
A√116ms−1B√32ms−1C5ms−1D√73ms−1To a stationary man, rain appears to be falling at his back at an angle 30∘ with the vertical. As he starts moving forward with a speed of 0.5ms−1, he finds that the rain is falling vertically.
The speed of rain with respect to the moving man is.A0.5ms−1B1.0ms−1C0.5√3ms−1D0.45ms−1
A man moving in rain holds his unbrella inclined to the vertical even though the rain drops are falling vertically downwards . Why ?
View SolutionA man moving with a velocity of 5m/s on a horizontal road observes that raindrops fall at an angle of 45∘ with the vertical. When he moves with a velocity of 16m/s along an inclined plane, which is inclined at 30∘ with the horizontal , he observes raindrops falling vertically downward as shown in the figure. Find the actual velocity of the raindrops.
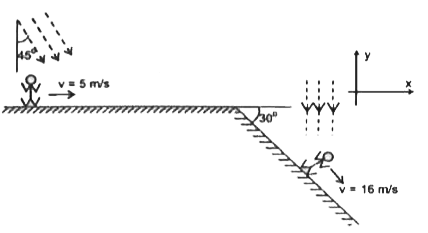
View SolutionA man moving with a velocity of 5m/s on a horizontal road observes that raindrops fall at an angle of 45∘ with the vertical. When he moves with a velocity of 16m/s along an inclined plane, which is inclined at 30∘ with the horizontal , he observes raindrops falling vertically downward as shown in the figure. Find the actual velocity of the raindrops.

View SolutionA man standing on a inclined plain observes rain is falling verticaly. When he starts moving down the inclined plain with velocity v=6m/s observes rain hitting him horizontally.

The actual velocity of rain is
View SolutionWhen a man moves down the inclined plane with a constant speed 5ms−1 which makes an angle of 37∘ with the horizontal, he finds that the rain is falling vertically downward. When he moves up the same inclined plane with the same speed, he finds that the rain makes an angle θ=tan−1(78) with the horizontal. The speed of the rain is
View Solution
