Question

Draw the resultant simplified logie cireuit.
(i) $A\bullet \overline{B}+\overline{A}\bullet B=Y$ (ii) $(A+\overline{b})\bullet (\overline{A}+B)=Y$

Explanation

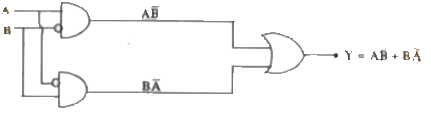
(i) The required logic diagram for the given Boolean expression is given in figure. Here the input B before applying to first AND gate and input A before applying to second AND gate have been inverted. The output of these gates are, therefore, `AbarB and barAB` respectively. These outputs are fed to OR gate which gives `T=AbarB+barAB` as shown in figure.

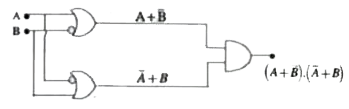
ii) The required logic diagram for the given Boolean expression is shown in figure. The input B to first OR gate and input A to second OR gate have been inverted. The output of these gates are, therefore, `A +barB and barA + B` as shown. These inputs when applied to AND gate give the required output. `Y=(A+barb).(barA+B)`