¯¯¯¯¯¯AB সরলরেখাংশের B বিন্দুতে পেনসিল কম্পাসের সাহায্যে BC লম্ব আঁকো ও পেনসিল কম্পাসের সাহায্যে ∠ABC-কে সমদ্বিখন্ডিত করো |
Step by step video & image solution for overline(AB) সরলরেখাংশের B বিন্দুতে পেনসিল কম্পাসের সাহায্যে BC লম্ব আঁকো ও পেনসিল কম্পাসের সাহায্যে angleABC-কে সমদ্বিখন্ডিত করো | by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Topper's Solved these Questions
- জ্যামিতিBOOK - CHAAYA PRAKASHINI (BENGALI)CHAPTER - জ্যামিতিEXERCISE - EXERCISE
12 Videos
- চতুর্ভুজের শ্রেণিবিভাগBOOK - CHAAYA PRAKASHINI (BENGALI)CHAPTER - চতুর্ভুজের শ্রেণিবিভাগEXERCISE - EXERCISE
14 Videos
- ত্রিভুজ অঙ্কনBOOK - CHAAYA PRAKASHINI (BENGALI)CHAPTER - ত্রিভুজ অঙ্কনEXERCISE - EXERCISE
21 Videos
Similar Questions
স্কেল ও পেনসিলের সাহায্যে PQ একটি 9 সেমি দৈর্ঘ্যের সরলরেখাংশ আঁকো। এই সরলরেখাংশকে সমদ্বিখণ্ডিত করে ‘প্রতি ভাগের দৈর্ঘ্য লেখো।
View Solutionত্রিভুজের বাহুর সংখ্যা 3টি। কর্ণের সংখ্যা □টি ?
View Solutionত্রিভুজের তিনটি বাহুর দৈর্ঘ্য দেওয়া আছে | যদি ত্রিভুজ আঁকা সম্ভব তবে ত্রিভুজ আঁকার চেষ্টা করো ও আর যদি ত্রিভুজ আঁকা সম্ভব নয় তবে কারণ দেখাও : 9 সেমি , 4 সেমি ও 4 সেমি
View SolutionIf A =B=C=1 and X= overline(ABC)+B overline(BC) + C overline(AB) , then X=
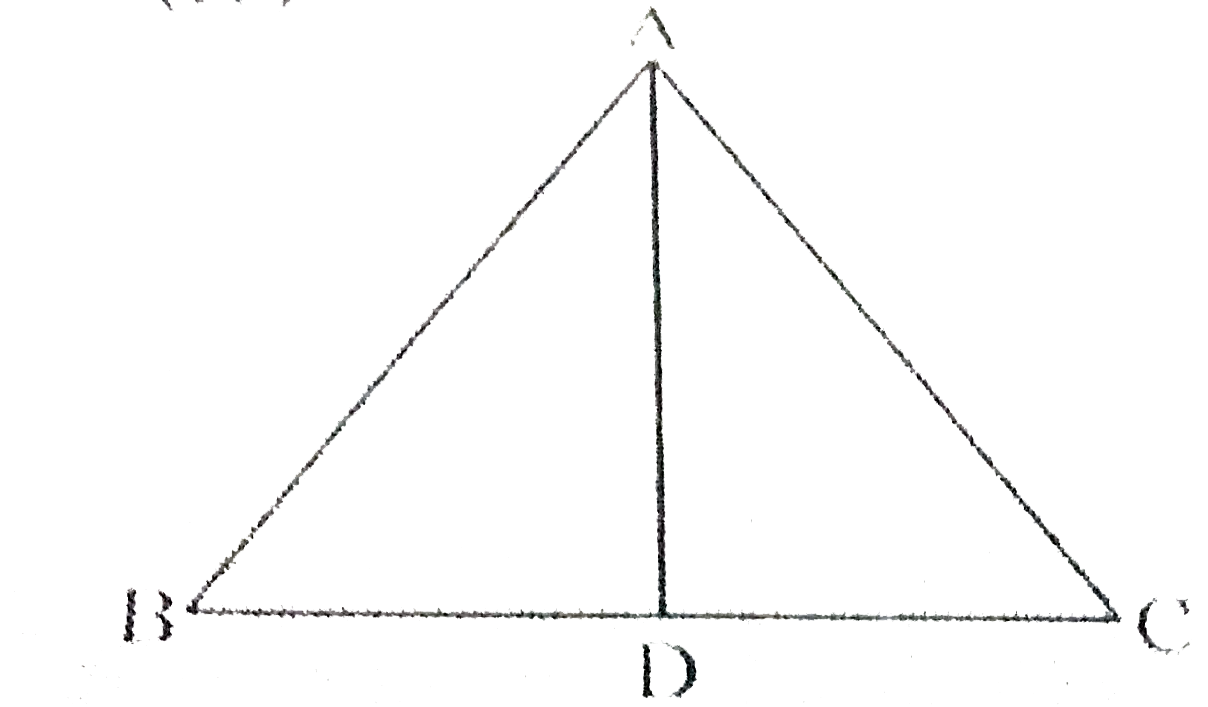
View Solutionआकर्ति में AB=AC और D,¯¯¯¯¯¯BC का मध्य बिंदु है!
View Solution¯¯¯¯¯¯OA+6¯¯¯¯¯¯BC+¯¯¯¯¯¯AB+5¯¯¯¯¯¯OB=
View SolutionIn an isosceles △ABC, If AB=AC and AD is the median, then ¯¯¯¯¯¯AD⋅¯¯¯¯¯¯BC=
View SolutionIn parallelogram ABCD, if ¯¯¯¯¯¯AB=¯aand¯¯¯¯¯¯AD=¯b, then the diagonals interm of ¯aand¯b are
View SolutionIf ABCD is a quadrilateral, then 2¯¯¯¯¯¯AB+3¯¯¯¯¯¯BC+2¯¯¯¯¯¯CD+¯¯¯¯¯¯DA+¯¯¯¯¯¯CA+¯¯¯¯¯¯DB=
View SolutionABCDE is a pentagon. The resultant of the vectors ¯¯¯¯¯¯AB,¯¯¯¯¯¯AE,¯¯¯¯¯¯BC,¯¯¯¯¯¯DC,¯¯¯¯¯¯EDand¯¯¯¯¯¯AC in terms of ¯¯¯¯¯¯AC is
View SolutionIf ABCDE is a pentago, then ¯¯¯¯¯¯AB+¯¯¯¯¯¯BC+¯¯¯¯¯¯CD+¯¯¯¯¯¯DE−¯¯¯¯¯¯AE=
View Solution¯a,¯b are vectors, ¯¯¯¯¯¯AB,¯¯¯¯¯¯BC determined by two adjacent sides of a regular hexagon ABCDEF.The vector represented by ¯¯¯¯¯¯EF is
View SolutionIf ¯aand¯b represent the sides ¯¯¯¯¯¯ABand¯¯¯¯¯¯BC of a regular hexagon ABCDEF, then ¯¯¯¯¯¯FA=
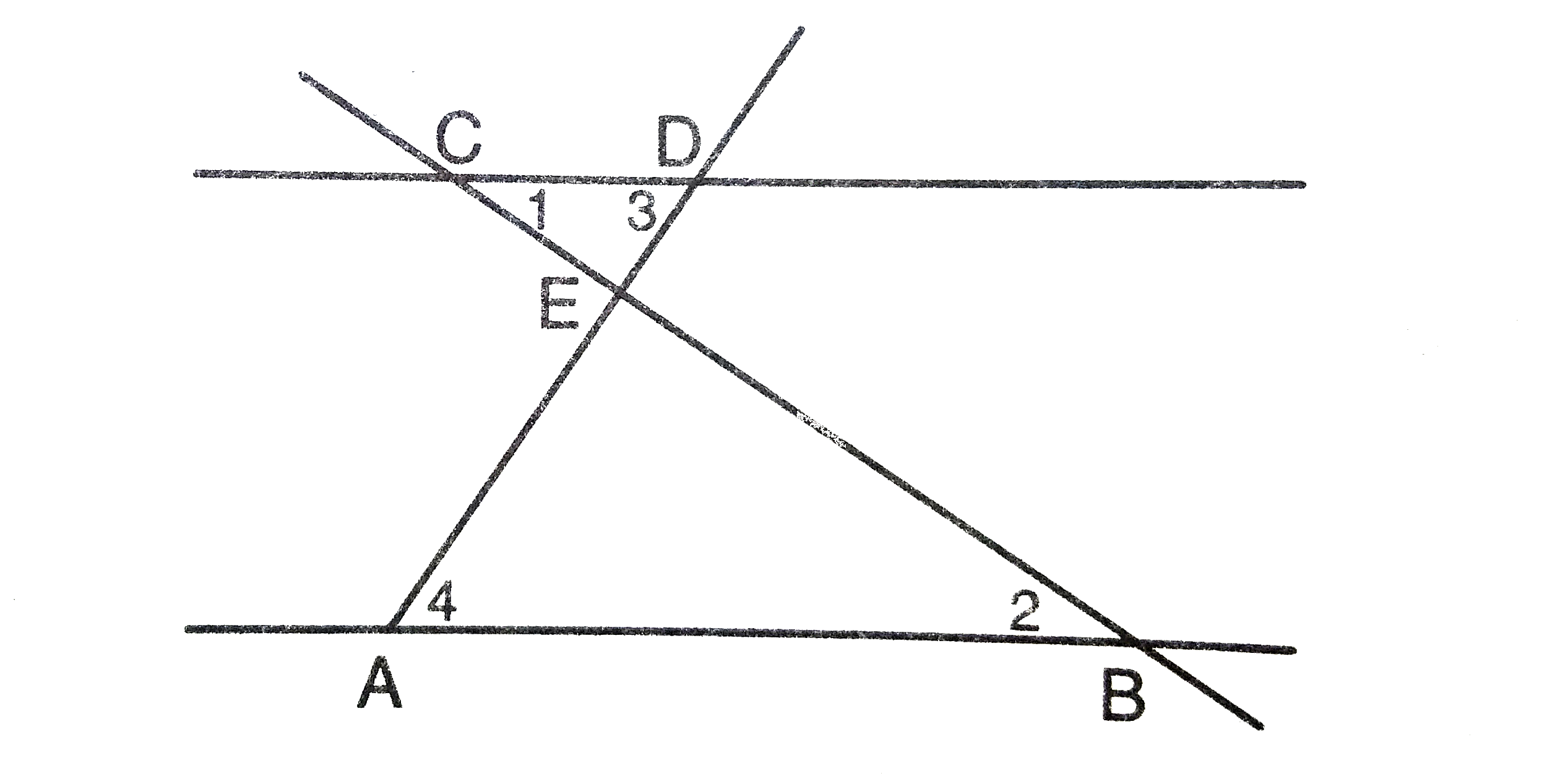
View SolutionIn the accomapanying figure, ¯¯¯¯¯¯AB∣∣¯¯¯¯¯¯CD. If AB=40,CD=16,andBC=49, what is the length of ¯¯¯¯¯¯BE?
View Solutionমনে করো xy সমতলে অবস্থিত S একটি বৃত্ত, যা x2+y2 = 4 সমীকরণ দ্বারা সংজ্ঞাত। মনে করো S বৃত্তের ওপর P এমন একটি বিন্দু যার স্থানাঙ্ক দুটি (x স্থানাঙ্ক ও yস্থানাঙ্ক) ধনাত্মক। মনে করো P বিন্দুতে S বৃত্তের স্পর্শক অক্ষদ্বয়কে M ও N বিন্দুতে ছেদ করেছে। তবে MN সরলরেখার মধ্যবিন্দু যে বক্রের ওপর অবস্থিত, তা হল—
View Solution¯¯¯¯¯¯AB সরলরেখাংশের B বিন্দুতে পেনসিল কম্পাসের সাহায্যে BC লম্ব আঁকো ও পেনসিল কম্পাসের সাহায্যে ∠ABC-কে সমদ্বিখন্ডিত করো |
View Solution
