A ball of mass m moving with a speed v makes a head on collision with an identical ball at rest. The kinetic energy after collision of the balls is three fourth of the original kinetic energy. The coefficient of restitution is

A

$\frac{1}{2}$

B

$\frac{1}{3}$

C

$\frac{1}{\sqrt{2}}$

D

$\frac{1}{\sqrt{3}}$

Text Solution

Verified by Experts

The correct Answer is:C

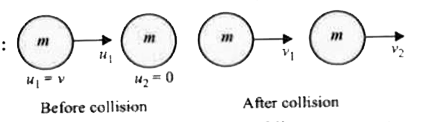
Applying the principle of conservation of linear momentum , we get
$m v = m v_{1} + m v_{2} or v = v_{1} + v_{2} \dots . (i)$
By defination of coefficient of restitution
$e = \frac{v_{2} - v_{1}}{u_{1} - u_{2}} = \frac{v_{2} - v_{1}}{v - 0} or v_{2} - v_{1} = e v ... . . (i i)$
As KE after collision = $\frac{3}{4} K E$ before collision
$∴ \frac{1}{2} m (v_{1}^{2} + v_{2}^{2}) = \frac{3}{4} \times \frac{1}{2} m v^{2} or v_{1}^{2} + v_{2}^{2} = \frac{3}{4} v^{2} ... . . (i i i)$
Squaring eq. (i), we get $v_{1}^{2} + v_{2}^{2} + 2 v_{1} v_{2} = v^{2} ... ... ... (i v)$
Subtracting (iiii), from equation (iv), we get
$2 v_{1} v_{2} = \frac{1}{4} v^{2} ... . . (v)$
Squaring (ii), we get
$v_{2}^{2} + v_{1}^{2} - 2 v_{1} v_{2} = e^{2} v^{2} ... . (v i)$
Using (iii) and (v) in (vi), we get
$\frac{3}{4} v^{2} - \frac{1}{4} v^{2} = e^{2} v^{2}, \frac{1}{2} v^{2} = e^{2} v^{2} or e = \frac{1}{\sqrt{2}}$ .

Updated on:21/07/2023

|

Class 11 PHYSICS WORK, ENERGY AND POWER

Topper's Solved these Questions

Similar Questions

Knowledge Check

Choose the most appropriate option.
A ball of mass m moving at a speed v makes a head on collision with an identical ball at rest. The kinetic energy at the balls after the collision is 3/4th of the original. What is the coefficient of restitution?

A $1 / \sqrt{3}$
B $1 / \sqrt{2}$
C $\sqrt{2}$
D $\sqrt{3}$

MTG GUIDE-WORK, ENERGY AND POWER-NEET CAFE (TOPICWISE PRACTICE QUESTIONS) (COLLISIONS)

Home
Ask Doubt
Profile