D and E are points on sides AB and AC respectively of ΔABC such that ar (DBC) = ar (EBC). Prove that DEIIBC.
Step by step video & image solution for D and E are points on sides AB and AC respectively of DeltaABC such that ar (DBC) = ar (EBC). Prove that DE II BC. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
D and E are points on sides AB and AC respectively of ti⟩ABC such that ar (DBC) = ar (EBC) . Prove that DE || BC
View SolutionAs shown in the map /_\ABC In points D and E are the points AB, AC above the sides and (/_\DBC) Y || = (/_\EBC) Show y is DE // BC.
View Solutionಮಧ್ಯ The midpoints of AB and AC sides of ABC are D and E respectively. Prove that V (DBC) = V (EBC) but DE || BC?
View SolutionIn the figure D, E are points on the sides AB and AC respectively of ΔABC such that ar(ΔDBC)=ar(ΔEBC) . Prove that DE || BC.
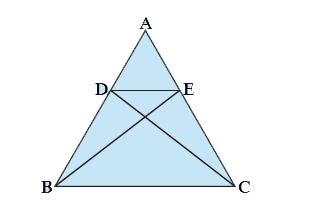
View SolutionIn the figure D, E are points on the sides AB and AC respectively of ΔABC such that ar(ΔDBC)=ar(ΔEBC) . Prove that DE || BC.
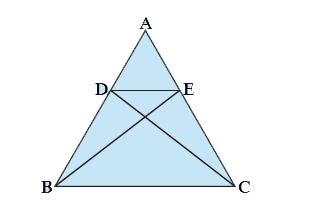
View SolutionIn the figure D, E are points on the sides AB and AC respectively of ΔABC such that ar(ΔDBC)=ar(ΔEBC) . Prove that DE || BC.
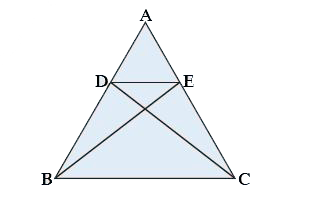
View SolutionIn △ABC, point D lies on side BC. E is the midpoint of AD. Prove that,
ar(EBC)=12ar(ABC)
View Solution