If (m + l)th term of an A.P. is twice the (n + l)th term, prove that (3m + l)th term is twice the (m + n + l)th term.Solution in Punjabi
Step by step video & image solution for If (m + l)th term of an A.P. is twice the (n + l)th term, prove that (3m + l)th term is twice the (m + n + l)th term. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Find out which of the following sequences are arithmetic progressions. For those which are arithmetic progressions, find out the common difference. :- 3,6,12,24,….
View SolutionA bicycle wheel makes 500 revolutions in moving 11 km. Find the diameter of the wheel.
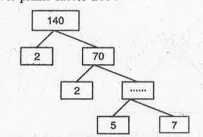
View SolutionComplete the prime factor tree :
View SolutionIf (m+1) th term of an A.P is twice the (n+1)th term,prove that (3m+1) th term is twice the (m+n+1) th term.
View SolutionIf (m+1) term of an A.P. is twice the(n+1) th term, prove that (3m+1) th terms is twice the (m+n+1) th term.
View SolutionIf (m+1)th term of an A.P. is twice the (n+1)th term, prove that (3m+1)th term is twice the (m+n+1)th term.
View SolutionIf an A.P.consists of n terms with first term a and n^(th) term l then show that the sum of the m^(th) term from the beginning and the m^(th) term from the end is (a+l) .
View Solution32.Show that the sum of (m+n)^(th) and (m-n)^(th) terms of an A.P.is equal to twice the m^(th) term
View SolutionShow that the sum of (m+n)thand (m−n)th term of an A.P. is equal to twice the mth term.
View Solutionदिखाइये कि समान्तर श्रेणी के (m+n)वें तथा (m-n) वें पदों का योग m वें पद का दोगुना होता है |
View SolutionIf (m+1)th term of an A.P. is twice the (n+1)th term, then prove that (3m+1)th term is twice the (m+n+1)th term
View SolutionShow that the sum of (m+n)thand(m−n)th term of an A.P is equal to twice the mth term.
View SolutionShow that the sum of (m+n)thand(m–n)th terms of an A.P. is equal to twice the mth term.
View SolutionShow that the sum of (m+n)thand(m–n)th terms of an A.P. is equal to twice the mth term.
View SolutionShow that the sum of (m+n)thand(m–n)th terms of an A.P. is equal to twice the mth term.
View Solution