कथन-I : माना फलन f(x)={−x2,x≤07x+8π≥0
क्योकिं
कथन-II : यदि f(a)<f(a−h) तथा f(a)<f(a+h) जहाँ 'h' पर्याप्त रूप से छोटा है, तब x) का स्थानीय निम्निष्ठ x=a पर होगा।
कथन-I सत्य है, कथन-II सत्य है, कथन-II, कथन-I का सही स्पष्टीकरण है।
कथन-I सत्य है, कथन-II सत्य है , कथन-I, कथन-I का सही स्पष्टीकरण नहीं है।
कथन - I सत्य है परन्तु कथन - II असत्य है।
कथन - I असत्य है परन्तु कथन - II सत्य है।
The correct Answer is:D
Topper's Solved these Questions
- MAXIMA-MINIMABOOK - ALLEN HINDICHAPTER - MAXIMA-MINIMAEXERCISE - EXERCISE-03 (MISCELLANEOUS TYPE QUESTIONS) (गद्यांश आधारित प्रश्न) गद्यांश-I
3 Videos
- MAXIMA-MINIMABOOK - ALLEN HINDICHAPTER - MAXIMA-MINIMAEXERCISE - EXERCISE-04 [A] (CONCEPTUAL SUBJECTIVE EXERCISE)
20 Videos
- MAXIMA-MINIMABOOK - ALLEN HINDICHAPTER - MAXIMA-MINIMAEXERCISE - EXERCISE-03 (MISCELLANEOUS TYPE QUESTIONS) (स्तम्भ सुमेलन)
2 Videos
- LIMIT BOOK - ALLEN HINDICHAPTER - LIMIT EXERCISE - Do yourself
29 Videos
- METHODS OF DIFFERENTIATIONBOOK - ALLEN HINDICHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - EXERCISE - 05 [B]
12 Videos
Similar Questions
माना f(x), घात 6 का वास्तविक मान बहुपद फलन है, जो निम्न प्रतिबंधों को संतुष्ट करता है -
(a) x=0 व 2 पर f का न्यूनतम मान है।
(b) x=1 पर f का महत्तम मान है।
(c) सभी x के लिए limx→01xln∣∣ ∣ ∣∣f(x)/x1001/x1101/x∣∣ ∣ ∣∣
समीकरण 8f(x)−1=0 के हलों की संख्या है -
View Solutionनिम्न फलनों के स्थानीयउच्चिष्ठ/ निम्नष्ठ बिन्दु ज्ञात कीजिए :
(a) f(x)=2x3−21x3+36x−20 (b)f(x)=−(x−1)3(x+1)2
(c) f(x)=xlnx (d)y=3x2+4x+4x2+x+1
View Solutionचार बिन्दु A, B, C तथा D परवलय y=ax2+bx+c पर क्रम से स्थित है तथा A, B व D के निर्देशांक A(-2.3), B(-1, 1), D(2,7) है।
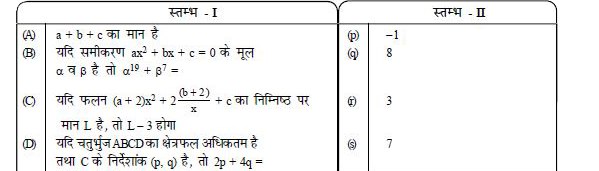
उपरोक्त जानकारी के आधार पर निम्न को सुमेलित कीजिए
View Solutionफलन y = f (x) के आरेख के सापेक्ष निम्न में से कौन से कथन सत्य या असत्य होंगे :
limn→−1+f(x)=1

View Solutionयदि ln (x+y) = 2xy हो , तो y'(0) =
View SolutionHow many orders are correct:
(a) H—F lt H-Cl lt H—Br lt H—I (Bond length)
(b) H—F lt H—I ltH—Br ltH—I (Acidic strength)
(C) H—I H—Br lt H—Cl ltH—F (Bond strength)
(d) H—Fgt H—Cl gt H—Br gt H—I (Thermodynamic stability)
(e) H—F lt H-Cl lt H—Br lt H—I (Reducing power)
(f) H—Fgt H-Igt H—Brgt H-Cl (Boiling point)
View SolutionLet f be a twice differentiable function such that
f''(x)=−f(x) and f'(x)=g(x).If h'(x)=[f(x)]2+[g(x)]2,
h(a)=8 and h(0)=2, then h(2)=
View SolutionLet f be a function such that f(x+f(y))=f(x)+y,∀x,y∈R, then find f(0). If it is given that there exists a positive real δ such that f(h) = h for 0<h<δ, then find f'(x)
View SolutionIn the mean value theorem, f(x+h)=f(x)+hf'(x+θh)(0<θ<1), if f(x)=ex, then show that the value of θ is independent of x.
View SolutionIf f(x)=1x, then find the value of θ in the mean value theorem
f(x+h)=f(x)+hf'(x+θh),0<θ<1.
View SolutionIf f(x)={2+x,x≥04−x,x<0, then f(f(x)) is given by :
View SolutionHow many of the following orders are correct ?
(a) H–F<H–Cl<H–Br<H–I (Bond length)
(b) H–F<H–Cl<H–Br<H–I (Acidic strength)
(c) H–F<H–Br<H–Cl<H–F (Bond strength)
(d) H–F>H–Cl>H–Br>H–I (thermodynamic stability)
(e) H–F<H–Cl<H–Br<H–I (reducing power)
(f) H–F>H–I>H–Br>H–Cl (Melting Point)
(g) H–F>H–I>H–Br>H–Cl (Boiling point)
View SolutionIf f(x)=x2 , then f(x+h)−f(x)h=
View Solutionनिम्न कथन सत्य या असत्य है
यदि f(x)=√x−2 तो : fof=f2
View Solutionमाना फलन निम रूप से परिभाषित है
f(x)={sin−1λ+x2,0<x<12x,x≥1
x = 1 पर F(x) का मान निम्निष्ठ होगा, यदि λ का मान है
View Solution
