(2,3) is a point on the side AB of ΔABC. The third vertex C moves such that the sides AC, BC are bisected by x2−y2=0 at right angles. Then C lies one
2x−3y=0
3x−2y=0
2x+3y=0
3x−2y=0
The correct Answer is:C
Topper's Solved these Questions
- STRAIGHT LINESBook:AAKASH SERIESChapter:STRAIGHT LINESExercise:LECTURE SHEET(EXERCISE-IV Problems on Anle between lines, Foot, Image Orthocentre, Circumcentre, Incentre, Angle Bisectors and Locus )(More than one correct answer Type Question)
Explore 6 Videos
- STRAIGHT LINESBook:AAKASH SERIESChapter:STRAIGHT LINESExercise:LECTURE SHEET(EXERCISE-IV Problems on Anle between lines, Foot, Image Orthocentre, Circumcentre, Incentre, Angle Bisectors and Locus )(Matrix Matching Type Question)
Explore 3 Videos
- STRAIGHT LINESBook:AAKASH SERIESChapter:STRAIGHT LINESExercise:LECTURE SHEET(EXERCISE-III Problems on Point of intersection of line and concurrency of lines)(Integer Type Questions Type Question)
Explore 2 Videos
- REVISION EXERCISEBook:AAKASH SERIESChapter:REVISION EXERCISEExercise:PROPERTIES OF TRIANGLES
Explore 57 Videos
- TANGENT AND NORMAL Book:AAKASH SERIESChapter:TANGENT AND NORMAL Exercise:ADVANCED SUBJECTIVE TYPE QUESTIONS
Explore 48 Videos
Similar Questions
If (2, 1), (-1, -2), (3, -3) are the mid points of the sides BC, CA, AB respectively of ΔABC, then the equation of the perpendicular bisector of AB is ax+by+c=0, then (a+b+c) is _______.
View SolutionIf (2, 1), (-1, -2), (3, -3) are the mid points of the sides BC, CA, AB respectively of ΔABC, then the equation of the perpendicular bisector of AB is ax+by+c=0, then (a+b+c) is _______.
View Solution
Knowledge Check
D is a point on the side BC of a ΔABC such that AD bisects ∠BAC . Then
ABD=CDBBA>BDCBD>BADCD>CAP and Q are the mid-points of the sides AB and BC, respectively, of ΔABC, right angled at B. Then:
AAQ2+CP2=AC2BAQ2+CP2=45AC2CAQ2+CP2=43AC2DAQ2+CP2=54AC2Mid-points of the sides AB and AC of a ΔABC are (3, 5) and (-3, -3) respectively, then the length of the side BC is
A10 unitB20 unitC15 unitD30 unit
The perpendicular from A on side BC of a ΔABC meets BC at D such that DB = 3CD .Prove that 2AB2=2AC2+BC2.
View SolutionABC is a right triangle, right - angled at the vertex A. A circle is drawn to touch the sides AB and AC at points P and Q respectively such that the other end points of the diameters passing through P and Q lie on the side BC. IF AB = 6 units, then the area (in sq. units) of the circular sector which lies inside the triangle is
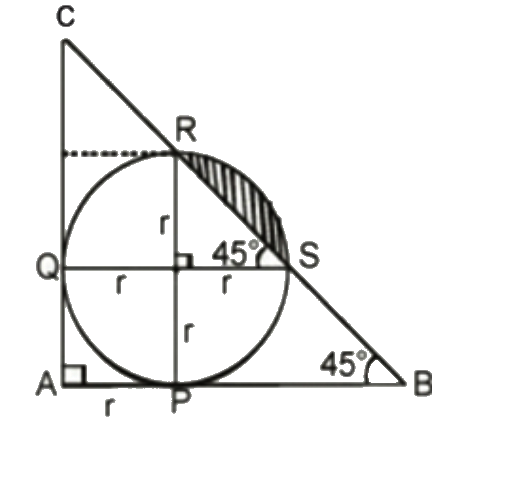
View SolutionMid points of the sides Ab and AC of a ΔABC are (3,5) and (-3,-3) respectively, then the length of the side BC is
View SolutionMid-points of the sides AB and AC of a ΔABC are (3, 5) and (-3, -3) respectively, then the length of the side BC is
View SolutionP and Q are points on the sides CA and CB respectively of ABC , right angled at C . Prove that AQ2+BP2=AB2+PQ2 .
View Solution
