A straight line passing through the point (87,33) cuts the positive direction of the co=ordinate axes at the point P and Q. If O is the origin then minimum area of the triangle OPQ is P then P-5740 is
The correct Answer is:7
Topper's Solved these Questions
- STRAIGHT LINESBOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - PRACTICE SHEET (EXERCISE-II Problems on normal form, symmetric from, perpendicular distances and Ratio) (LEVEL - I Straight objective Type Question)
31 Videos
- STRAIGHT LINESBOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - PRACTICE SHEET (EXERCISE-II Problems on normal form, symmetric from, perpendicular distances and Ratio) (LEVEL - II More than One correct answer Type Quesions)
3 Videos
- STRAIGHT LINESBOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - PRACTICE SHEET(EXERCISE-I Problems on slopes, Equation of Lines and Interceptes of a line , Areas) (LEVEL -II More than One correct answer Type Questions)
2 Videos
- REVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - REVISION EXERCISEEXERCISE - PROPERTIES OF TRIANGLES
57 Videos
- TANGENT AND NORMAL BOOK - AAKASH SERIESCHAPTER - TANGENT AND NORMAL EXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
48 Videos
Similar Questions
A straight line passing through the point (87, 33) cuts the positive direction of the coordinate axes at the point P and Q. If Q is the origin then the minimum area of the triangle OPQ is.
View SolutionLet (h,k) be a fixed point, where h>0,k>0. A straight line passing through this point cuts the positive direction of the coordinate axes at the point PandQ . Find the minimum area of triangle OPQ,O being the origin.
View Solution
Knowledge Check
Let (h,k) be a fixed point, where h>0,k>0. A straight line passing through this point cuts the positive direction of the coordinate axes at the point PandQ . Find the minimum area of triangle OPQ,O being the origin.
AhkB2hkC12hkDNone of theseA straight line L with negative slope passes through the point (8,2) and cuts the positive coordinate axes at the points P and Q .as L varies, the absolute minimum value of OP+OQ2O is origin is
A10B18C16D112
Let (h,k) be a fixed point, where h>0,k>0. A straight line passing through this point cuts the positive direction of the coordinate axes at the point PandQ . Find the minimum area of triangle OPQ,O being the origin.
View SolutionA straight line L with negative slope passes through the point (8,2) and cuts the positive coordinate axes at the points P and Q .as L varies, the absolute minimum value of OP+OQ2O is origin is
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
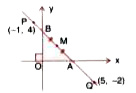
The equation of the line.
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
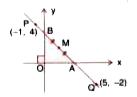
The co-ordinates of M.
View SolutionA line is drawn through the point (1, 2) to meet the coordinate axes at P and Q such that it forms a triangle OPQ, where O is the origin. If the area of the triangle OPQ is least, then the slope of the line PQ is
View SolutionA line is drawn through the point (1,2) to meet the coordinate axes at P and Q such that it forms a triangle OPQ ,where 0 is the origin.If the area of the triangle OPQ is least,the slope of the line PQ is k ,then |k|=
View Solution
