There are two concentric conducting spherical shells of radius $r_{A}$ and $r_{B}$ respectively $(r_{B} = 2 r_{A})$ . If the inner shell is given a charge Q and outer shell is given a charge 2Q, then the potential difference between the surfaces of the shells will be $K = \frac{1}{4 π ε_{0}}$

$k \frac{Q}{2 r_{A}}$

$k \frac{Q}{r_{A}}$

$k \frac{Q}{2 r_{B}}$

$k \frac{Q}{4 r_{B}}$

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the potential difference between the surfaces of two concentric conducting spherical shells, we can follow these steps:
Step 1: Understand the System
We have two concentric spherical shells:
- Inner shell with radius $r_{A}$ and charge $Q$ .
- Outer shell with radius $r_{B} = 2 r_{A}$ and charge $2 Q$ .
Step 2: Write the Formula for Electric Potential
The electric potential $V$ at a distance $r$ from a point charge $Q$ is given by:
$V = \frac{K Q}{r}$
where $K = \frac{1}{4 π ϵ_{0}}$ .
Step 3: Calculate the Potential at the Inner Shell (Surface A)
The potential $V_{A}$ at the surface of the inner shell (radius $r_{A}$ ) due to its own charge $Q$ and the charge on the outer shell $2 Q$ is:
$V_{A} = \frac{K Q}{r_{A}} + \frac{K (2 Q)}{r_{A}} = \frac{K Q}{r_{A}} + \frac{2 K Q}{r_{A}} = \frac{3 K Q}{r_{A}}$
Step 4: Calculate the Potential at the Outer Shell (Surface B)
The potential $V_{B}$ at the surface of the outer shell (radius $r_{B} = 2 r_{A}$ ) due to its own charge $2 Q$ and the charge on the inner shell $Q$ is:
$V_{B} = \frac{K (2 Q)}{2 r_{A}} + \frac{K Q}{2 r_{A}} = \frac{2 K Q}{2 r_{A}} + \frac{K Q}{2 r_{A}} = \frac{3 K Q}{2 r_{A}}$
Step 5: Calculate the Potential Difference
The potential difference $Δ V$ between the inner shell and the outer shell is given by:
$Δ V = V_{A} - V_{B}$
Substituting the values calculated:
$Δ V = \frac{3 K Q}{r_{A}} - \frac{3 K Q}{2 r_{A}}$
To simplify:
$Δ V = \frac{3 K Q}{r_{A}} - \frac{3 K Q}{2 r_{A}} = \frac{3 K Q}{r_{A}} (1 - \frac{1}{2}) = \frac{3 K Q}{r_{A}} \cdot \frac{1}{2} = \frac{3 K Q}{2 r_{A}}$
Final Result
Thus, the potential difference between the surfaces of the shells is:
$Δ V = \frac{3 K Q}{2 r_{A}}$

Updated on:18/9/2024

Consider two concentric spherical metal shells of radii $r_{1}$ and $r_{2} (r_{2} > r_{1})$ . If the outer shell has a charge q and the inner one is grounded, then the charge on the inner shell is
A $\frac{- r_{2}}{r_{1}} q$
Bzero
C $\frac{- r_{1}}{r_{2}} q$
D $- q$
There are two concentric spherical shell of radii r and $2 r$ . Initially, a charge Q is given to the inner shell and both the switches are open.

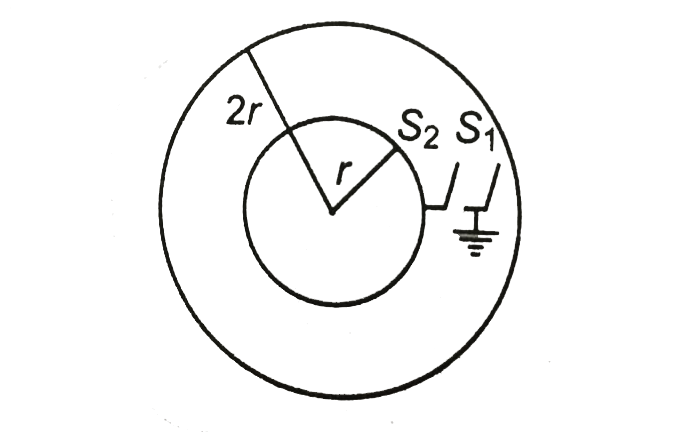
The twio steps of the above two problems are repeated b times, the potential differences between the shells will be
A $\frac{1}{2^{n + 1}} [\frac{Q}{4 π ε_{0} r}]$
B $\frac{1}{2^{n}} [\frac{Q}{4 π ε_{0} r}]$
C $\frac{1}{2^{n}} [\frac{Q}{4 π ε_{0} r}]$
D $\frac{1}{2^{n - 1}} [\frac{Q}{2 π ε_{0} r}]$
There are two concentric hollow conducting spherical shells of radii r and $R (R > r)$ . The charge on the outer shell is Q. What charge should be given to the inner shell, so that the potential at a point P , at a distance 2R from the common centre is zero ?
A $\frac{- Q r}{R}$
B $\frac{- Q R}{r}$
C $\frac{- 2 Q R}{r}$
D $- Q)$