Refractive index of a thin soap film of a uniform thickness is 1.38. The smallest thickness of the film that gives an interference maxima in the reflected light when light of wavelength 5520 Å falls at normal incidence is
500Å
1500Å
1000 Å
2000 Å
To find the smallest thickness of a thin soap film that gives an interference maximum in the reflected light, we can follow these steps:
Step 1: Understand the condition for interference maxima
For constructive interference (maxima) in a thin film, the condition is given by:
2μt=(m+1/2)λ
where:
- μ is the refractive index of the film,
- t is the thickness of the film,
- λ is the wavelength of light in vacuum,
- m is the order of interference (for the smallest thickness, we take m=0).
Step 2: Rearranging the formula
For the smallest thickness that gives an interference maximum, we can simplify the equation:
2μt=λ2
Thus, we can express t as:
t=λ4μ
Step 3: Substitute the values
Given:
- Refractive index μ=1.38
- Wavelength λ=5520 Å (1 Å = 10−10 m)
First, convert the wavelength into meters:
λ=5520Å=5520×10−10m=5.52×10−7m
Now, substitute the values into the formula for t:
t=5.52×10−74×1.38
Step 4: Calculate the denominator
Calculate 4×1.38:
4×1.38=5.52
Step 5: Calculate the thickness
Now substitute this back into the equation for t:
t=5.52×10−75.52
This simplifies to:
t=10−7m
Step 6: Convert to appropriate units
Convert t back to Ångströms:
t=10−7m=10−7×1010Å=103Å=1000Å
Final Answer
The smallest thickness of the film that gives an interference maximum is:
t=1000Å
---
For constructive interference (maxima) in a thin film, the condition is given by:
2μt=(m+1/2)λ
where:
- μ is the refractive index of the film,
- t is the thickness of the film,
- λ is the wavelength of light in vacuum,
- m is the order of interference (for the smallest thickness, we take m=0).
For the smallest thickness that gives an interference maximum, we can simplify the equation:
2μt=λ2
Thus, we can express t as:
t=λ4μ
Given:
- Refractive index μ=1.38
- Wavelength λ=5520 Å (1 Å = 10−10 m)
λ=5520Å=5520×10−10m=5.52×10−7m
t=5.52×10−74×1.38
Calculate 4×1.38:
4×1.38=5.52
Now substitute this back into the equation for t:
t=5.52×10−75.52
t=10−7m
Convert t back to Ångströms:
t=10−7m=10−7×1010Å=103Å=1000Å
The smallest thickness of the film that gives an interference maximum is:
t=1000Å
Topper's Solved these Questions
Similar Questions
Refractive index of a thin soap film of a uniform thickness is 1.34. Find the smallest thickness of the film that gives in interference maximum in the reflected light when light of wavelength 5360Å fall at normal incidence.
View SolutionAn oil film covers the surface of a small pond. The refractive index of the oil is greater than that of water. At one point on the film, the film has the smallest non-zero thickness for which there will be destructive interference in the reflected light when infrared radiation with wavelength 800 nm is incident normal to the film. When this film is viewed at normal incidence at this same point, for what visible wavelengths, if any, will there be constructive interference? (Visible light has wavelengths between 400nm and 700 nm )
View Solution
Knowledge Check
A ray of light is incident at an angle of 45∘ (ralative to the normal) on a thin film of a liquid with an index of refraction of √2. Assuming that the medium of both sides of the film is air, find the minimum thickness of the film required for constructive interference in the reflected light for sodium light of wavelenght 600 nm.
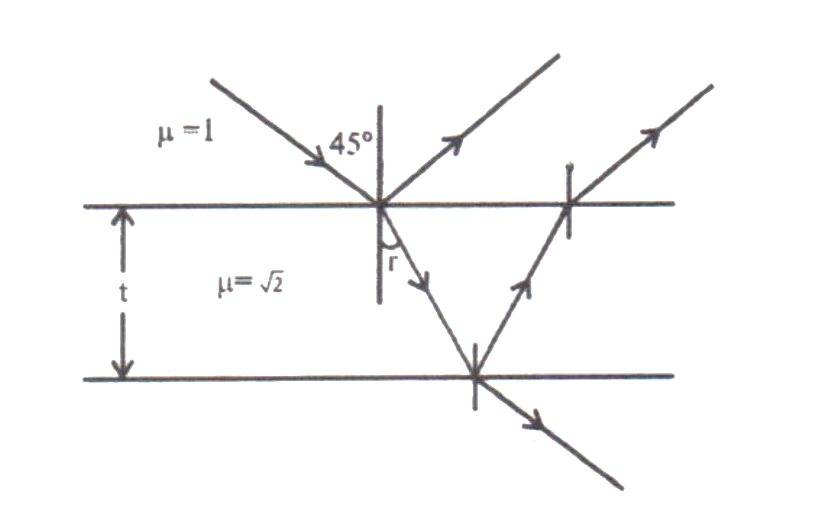 A122 nmB212 nmC173 nmD320 nm
A122 nmB212 nmC173 nmD320 nmA light of wavelength 5890Å falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is
A2.945×10−7mB3.945×10−7mC4.95×10−7mD1.945×10−7mA light of wavelength 5890Å falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is
A(a) 2.945×10−7mB(b) 3.945×10−7mC(c) 4.95×10−7mD(d) 1.945×10−7m
A lens (μ=1.5) is coated with a thin film of refractive index 1.2 order to reduce the reflected form its surface atλ=4800Å.Find the maximum thickness of the film which will minimized the intensity of the reflected light .(Assume near normal incidence)
View SolutionIf the wavelength of incident light on a soap film is 700 nm then its minimum thickness 10 t nm so that constructive interference in reflected light can be observed. The value of t is ………..[Take refractive index of the film as 43]
View SolutionWhat is the minimum thickness of a thin film (μ=1.2) that results in constructive interference in the reflected light? If the film is illuminated with light whose wavelength in free space is λ=500nm ?
View SolutionWhat is the minimum thickness of thin film required for constructive interference in the reflected light through it ?
(Given, the refractive index of the film =1.5, wavelength of the lilght incident on the film =600 nm.
View SolutionFor observing interference in thin films with a light of wave length λ the thickness of the film:
View Solution