The magnetic field at the centre of a tightly wound spiral coil with inner radius R and outer radius 4.5 R with number of turns 22, when current i is flowing through it, is
Zero
To find the magnetic field at the center of a tightly wound spiral coil, we can use the formula for the magnetic field due to a coil:
B=μ0NI2π(B−A)ln(BA)
Where:
- B is the outer radius of the coil,
- A is the inner radius of the coil,
- N is the number of turns,
- I is the current flowing through the coil,
- μ0 is the permeability of free space.
1. Identify the given values:
- Inner radius A=R
- Outer radius B=4.5R
- Number of turns N=22
- Current I=i
2. Substitute the values into the formula:
B=μ0NI2π(4.5R−R)ln(4.5RR)
3. Calculate B−A:
B−A=4.5R−R=3.5R
4. Calculate ln(BA):
ln(4.5RR)=ln(4.5)
5. Substitute these values back into the equation:
B=μ0⋅22⋅I2π⋅3.5R⋅ln(4.5)
6. Simplify the expression:
B=μ0⋅22⋅I7πR⋅ln(4.5)
Final Result:
Thus, the magnetic field at the center of the tightly wound spiral coil is:
B=22μ0I7πRln(4.5)
- B is the outer radius of the coil,
- A is the inner radius of the coil,
- N is the number of turns,
- I is the current flowing through the coil,
- μ0 is the permeability of free space.
- Inner radius A=R
- Outer radius B=4.5R
- Number of turns N=22
- Current I=i
B=μ0NI2π(4.5R−R)ln(4.5RR)
B−A=4.5R−R=3.5R
ln(4.5RR)=ln(4.5)
B=μ0⋅22⋅I2π⋅3.5R⋅ln(4.5)
B=μ0⋅22⋅I7πR⋅ln(4.5)
Thus, the magnetic field at the center of the tightly wound spiral coil is:
B=22μ0I7πRln(4.5)
Topper's Solved these Questions
Similar Questions
How does the strength of the magnetic field at the centre of a circular coil of a wire depend on: (a) radius of the coil (b) number of turns in the coil.
View SolutionHow does the strength of the magnetic field at the centre of a circular coil of a wire depend on: (a) radius of the coil (b) number of turns in the coil.
View Solution
Knowledge Check
The magnetic induction at the centre of a current carrying circular of coil radius r, is
ADirectly proportional to rBInversely proportional rCDirectly proportional to rDInversely proportional to rRatio of magnetic field at the centre of a current carrying coil of radius R and at a distance of 3R on its axis is
A10√10B20√10C2√10D√10The magnitude of the magnetic field at the centre of the tightly wound 150 turn coil of radius 12 cm carrying a current of 2 A is
A18GB19.7GC15.7GD17.7G
What will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionA long insulated copper wire is closely wound as a spiral of N turns. The spiral has inner radius a and outer radius b. The spiral lies in the xy-plane and a steady current I flows through the wire. Thez-component of the magetic field at the centre of the spiral is
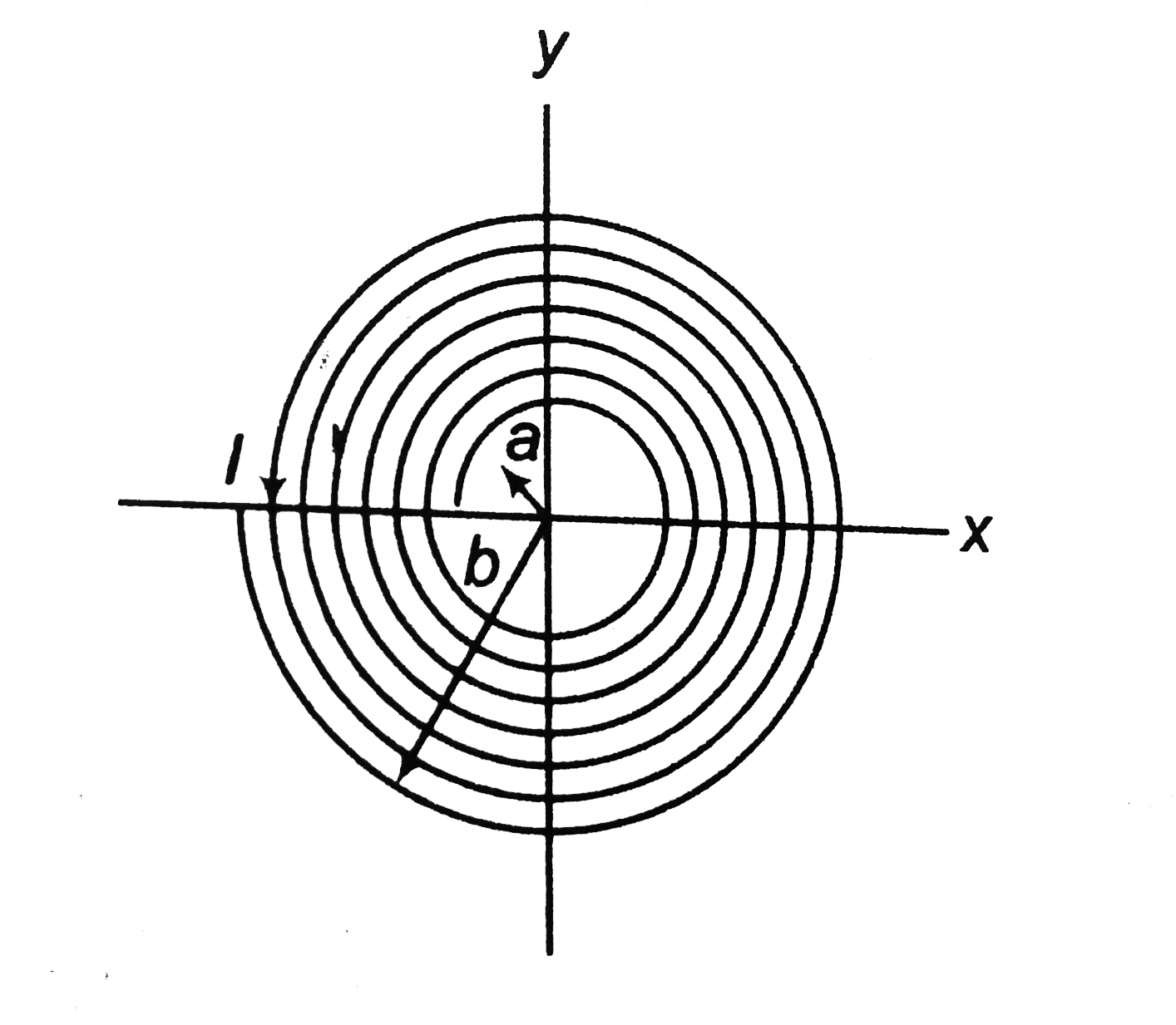
View SolutionA long insulated copper wire is closely wound as a spiral of N turns. The spiral has inner radius a and outer radius b. The spiral lies in the xy-plane and a steady current I flows through the wire. Thez-component of the magetic field at the centre of the spiral is

View SolutionA tightly wound 90 turn coil of radius 15 cm has a magnetic field of 4×10−4T at its centre. The current flowing through it is
View SolutionThe magnetic moment of a current I carrying circular coil of radius r and number of turns N varies as
View Solution