A charge Q is enclosed by a Gaussian surface. If surface area is doubled and charge Q is tripled then the outward electric flux will
Be tripled
Be32 times of the initial flux
Remain same
Reduced by one third of initial flux
To solve the problem, we need to analyze how the electric flux through a Gaussian surface is affected by changes in the enclosed charge and the surface area.
1. Understanding Electric Flux:
The electric flux (Φ) through a closed surface is given by Gauss's law, which states:
Φ=Qencϵ0
where Qenc is the charge enclosed by the surface and ϵ0 is the permittivity of free space.
2. Initial Condition:
Let's denote the initial charge as Q. The electric flux through the Gaussian surface when the charge is Q is:
Φ=Qϵ0
3. Changes in Charge and Surface Area:
According to the problem, the charge is tripled, so the new charge Q′=3Q. The surface area is doubled, but according to Gauss's law, the flux depends only on the charge enclosed and not on the surface area.
4. New Electric Flux Calculation:
The new electric flux Φ′ when the charge is 3Q is:
Φ′=Q′ϵ0=3Qϵ0
5. Comparing the Two Fluxes:
Now, we compare the new flux with the initial flux:
Φ′=3⋅Qϵ0=3Φ
6. Conclusion:
The outward electric flux will be tripled when the charge is tripled, regardless of the change in surface area. Therefore, the final answer is that the outward electric flux will be three times the original flux.
Final Answer:
The outward electric flux will triple.
The electric flux (Φ) through a closed surface is given by Gauss's law, which states:
Φ=Qencϵ0
where Qenc is the charge enclosed by the surface and ϵ0 is the permittivity of free space.
Let's denote the initial charge as Q. The electric flux through the Gaussian surface when the charge is Q is:
Φ=Qϵ0
According to the problem, the charge is tripled, so the new charge Q′=3Q. The surface area is doubled, but according to Gauss's law, the flux depends only on the charge enclosed and not on the surface area.
The new electric flux Φ′ when the charge is 3Q is:
Φ′=Q′ϵ0=3Qϵ0
Now, we compare the new flux with the initial flux:
Φ′=3⋅Qϵ0=3Φ
The outward electric flux will be tripled when the charge is tripled, regardless of the change in surface area. Therefore, the final answer is that the outward electric flux will be three times the original flux.
The outward electric flux will triple.
Topper's Solved these Questions
Similar Questions
A positive charge q is enclosed by a Guassian spherical surface of radius 'a' . If its radius is increased to 4a then the net outward flux will
View SolutionA charge Q is enclosed by a spherical Gaussian surface of radius R. If the radius of the sphere is double, how will the outward electric flux charge ? If a charge −Q is brought outside the Gaussian surface, will the electric flux reduce to zero ?
View Solution
Knowledge Check
A charge Q is enclosed by a Gaussian spherical surface of radius R, If the radius is doubled, then the outward electric flux will
Abe reduced to halfBremains the sameCbe doubledDincreases four timesA charge Q is enclosed by a Gaussian spherical surface of radius R. If the radius is doubled, then the outward electric flux will
Abe reduced to halfBremain the sameCbe doubledDincrease four timesAnswer questions on the basis of your understanding of the following paragraph and the related studied concept.
Electric flux, in general , through any surface is defined as per relation:
ϕE=∫→E.→ds , where integration has to be performed over the entire surface through which flux is required. The surface under consideration may be a closed one or an open surface. When flux through a closed surface is required we use a small circular sign on the integration symbol. Thus flux over a closed surface ∮E=∮→E.→ds. it is customary to take the outward normal as positive in this case.
A German physicist Gauss established a fundamental law to find electric flux over a closed surface. As per Gauss' law , the flux of the net electric field through a closed surface equals the net charge enclosed by the surface divided by ∈0.Mathematically ∮→E.→ds=1∈0[qen] , where qcn is the net charge enclosed within the surface. It is possible to derive Gauss' law from Coulomb's laws. Gauss' law can be applied to obtain electric field at a point due to continuous charge distribution for a number of symmetric charge configurations.
A charge q is enclosed by a Gaussian spherical surface of raidus R. If the radius is doubled then the electric flux will.Abe doubledBincreases four timesCbe reduced to half.Dremain the same.
Can charge lie on a Gaussian surface?
View SolutionDefine electric flux. Write its SI unit. A charge q is enclosed by a spherical surface of radius R. If the radius is reduced to half, how would the electric flux through the surface change ?
View SolutionA charge q is enclosed as shown below the electric flux is
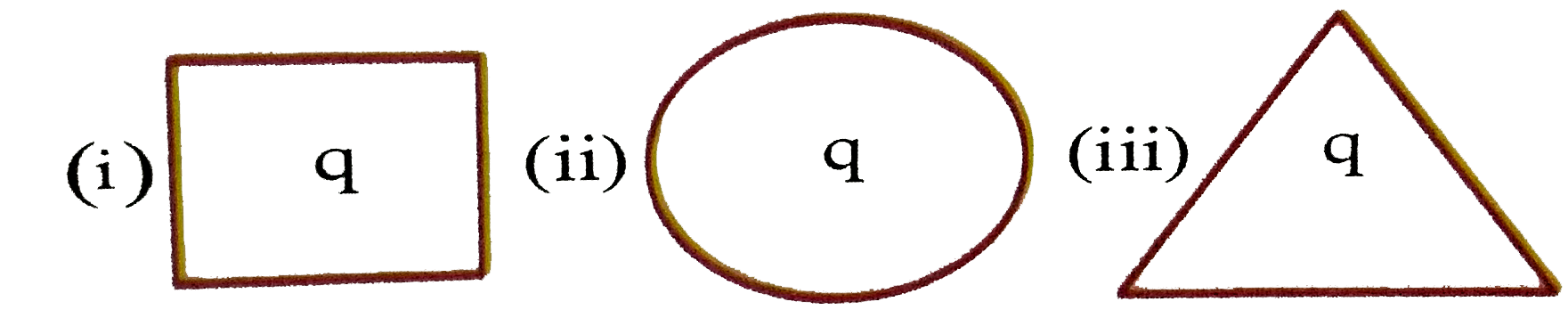
View SolutionA charge q is enclosed as shown, below the electric flux is
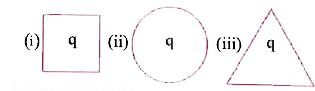
View SolutionA charge q is enclosed by an imaginary Gaussian surface.

If radius of surface is increasing at a rate drdt=K, then
View Solution
