Four thin rods of same mass and same length L form a square Radius of gyration of this system about an axis which is perpendicular to plane of square and passes through its centre is
L√3
√3L
L√2
L
To find the radius of gyration of a system of four thin rods forming a square about an axis perpendicular to the plane of the square and passing through its center, we can follow these steps:
Step 1: Understand the System
We have four thin rods of the same mass m and length L arranged to form a square. The center of the square is point C.
Step 2: Moment of Inertia of One Rod
The moment of inertia I0 of a single thin rod about its center is given by the formula:
I0=mL212
Step 3: Use the Parallel Axis Theorem
To find the moment of inertia of one rod about the center of the square (point C), we apply the parallel axis theorem. The distance from the center of the rod to the center of the square is L2. The moment of inertia about point C is:
IC=I0+m(L2)2
Substituting I0:
IC=mL212+m(L24)
IC=mL212+mL24
Step 4: Simplify the Moment of Inertia for One Rod
To combine the terms, we need a common denominator:
mL24=3mL212
Thus,
IC=mL212+3mL212=4mL212=mL23
Step 5: Total Moment of Inertia for Four Rods
Since there are four identical rods, the total moment of inertia Itotal is:
Itotal=4×IC=4×mL23=4mL23
Step 6: Relate Moment of Inertia to Radius of Gyration
The radius of gyration k is defined by the equation:
Itotal=mk2
Substituting for Itotal:
4mL23=mk2
Dividing both sides by m:
4L23=k2
Step 7: Solve for Radius of Gyration
Taking the square root of both sides gives:
k=L√43=2L√3
Final Answer
Thus, the radius of gyration k of the system about the specified axis is:
k=2L√3
We have four thin rods of the same mass m and length L arranged to form a square. The center of the square is point C.
The moment of inertia I0 of a single thin rod about its center is given by the formula:
I0=mL212
To find the moment of inertia of one rod about the center of the square (point C), we apply the parallel axis theorem. The distance from the center of the rod to the center of the square is L2. The moment of inertia about point C is:
IC=I0+m(L2)2
Substituting I0:
IC=mL212+m(L24)
IC=mL212+mL24
To combine the terms, we need a common denominator:
mL24=3mL212
Thus,
IC=mL212+3mL212=4mL212=mL23
Since there are four identical rods, the total moment of inertia Itotal is:
Itotal=4×IC=4×mL23=4mL23
The radius of gyration k is defined by the equation:
Itotal=mk2
Substituting for Itotal:
4mL23=mk2
Dividing both sides by m:
4L23=k2
Taking the square root of both sides gives:
k=L√43=2L√3
Thus, the radius of gyration k of the system about the specified axis is:
k=2L√3
Similar Questions
Four thin rods of same mass M and same length l, form a square as shown in figure. Moment of inertia of this system about an axis through centre O and perpendicular to its plane is
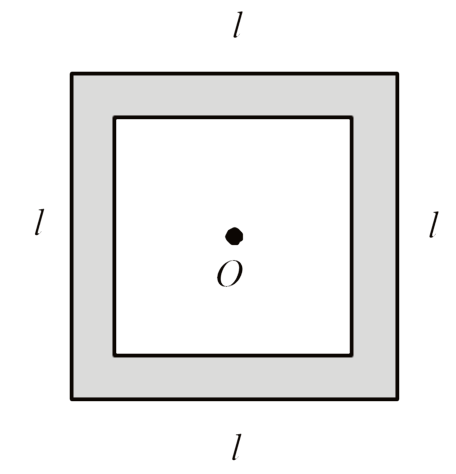 View Solution
View SolutionFour point size bodies each of mass m are fixed at four corners of light square frame of side length 1m. The radius of gyration of these four bodies about an axis perpendicular to the plane of frame passing through its centre is
View Solution
Knowledge Check
- Question 1 - Select One
Four particles each of mass m are placed at the corners of a square of side length l. The radius of gyration of the system about an axis perpendicular to the plane of square and passing through its centre is
Al√2Bl2ClD√2l - Question 2 - Select One
Four thin rods of same mass m and same length L form a square as shown in the figure. M.I. of this system about an axis through center O and perpendicular to its plane is
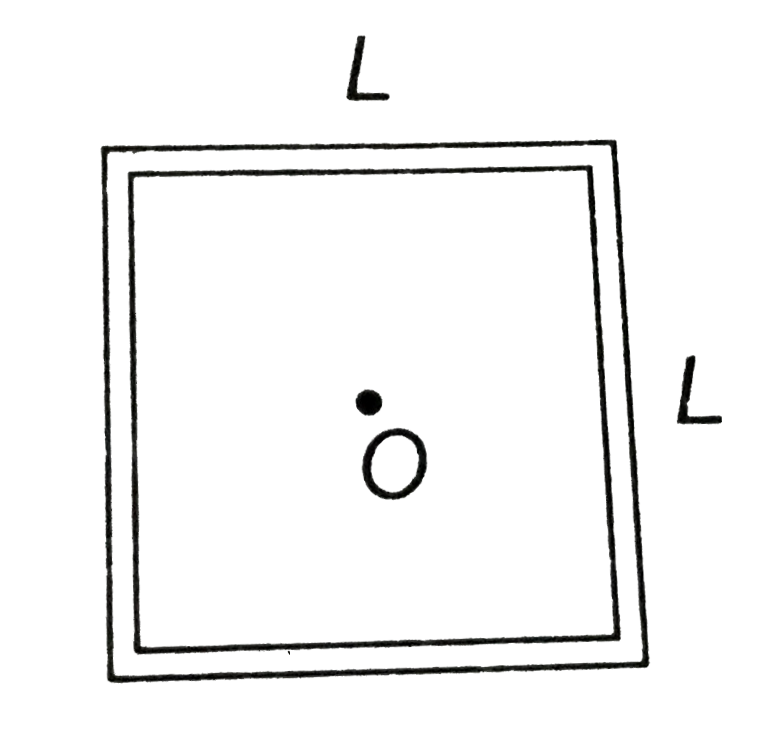 A4mL23BmL23CmL26D4mL23
A4mL23BmL23CmL26D4mL23 - Question 3 - Select One
Four particles each of mass m are placed at the corners of a square of side length l. The radius of gyration of the system about an axis perpendicular to the plane of square and passing through its centre is
Al√2Bl2ClD(√2)l
Four thin rods of same mass M and same length l, form a square as shown in figure. Moment of inertia of this system about an axis through centre O and perpendicular to its plane is
 View Solution
View SolutionFour particles each of mass m are placed at the corners of a square of side length l. The radius of gyration of the system about an axis perpendicular to the plane of square and passing through its centre is
View SolutionFour thin rods same mass M and same length l, from a square as shown in figure. Moment of inertia of this system about an axis passing through one corner of the square and perpendicular to its plane is
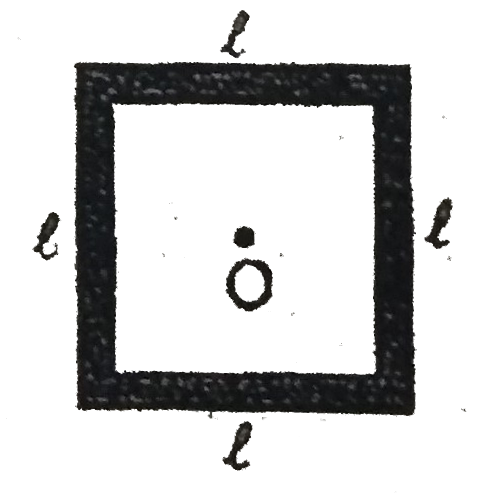 View Solution
View SolutionRadius of gyration of disc rotating about an axis perpendicular to its plane passing through through its centre is (If R is the radius of disc)
View SolutionFour particles each of mass m are placed at the corners of a square of side length l. The radius of gyration of the system the moment of inertia of four bodies about an axis perpendicular to the plane of frame and passing through a corner is
View Solution

