A block of mass m1 resting on a frictionless horizontal surface is connected to a spring of spring constant k that is anchored in a near by wall. A block of mass m2 is placed on the top of the first block. The coefficient of static friction between the two blocks is μ Assuming that the two blocks move together as a unit, find the period of oscillation of the system and also find maximum oscillation amplitude that permits the two bodies to move as a unit.
2π√m1+m2k, (μm2g)/k
2π√m1+m2k, (μm1g)/k
2π√m1+m2k,(μ(m1+m2))/k
2π√m1+m2k, (μg(m1+m2))/k
To solve the problem, we will break it down into two parts: finding the period of oscillation of the system and determining the maximum oscillation amplitude that allows the two blocks to move together as a unit.
Step 1: Finding the Period of Oscillation
1. Identify the total mass of the system:
The total mass M of the system when both blocks move together is:
M=m1+m2
2. Use the formula for the period of a mass-spring system:
The period T of oscillation for a mass-spring system is given by:
T=2π√Mk
Substituting the total mass into the formula:
T=2π√m1+m2k
Step 2: Finding the Maximum Oscillation Amplitude
1. Determine the maximum static friction force:
The maximum static friction force Fmax that can act between the two blocks is given by:
Fmax=μm2g
where μ is the coefficient of static friction, m2 is the mass of the top block, and g is the acceleration due to gravity.
2. Relate the maximum friction force to the maximum acceleration:
The maximum force acting on the top block due to the oscillation is related to the maximum acceleration amax of the oscillating system:
Fmax=m2amax
The maximum acceleration can also be expressed in terms of angular frequency ω:
amax=ω2Amax
where Amax is the maximum amplitude.
3. Substituting for angular frequency:
The angular frequency ω is given by:
ω=√kM=√km1+m2
Therefore, the maximum acceleration becomes:
amax=(√km1+m2)2Amax=km1+m2Amax
4. Setting the two expressions for force equal:
Equating the two expressions for the maximum force:
μm2g=m2km1+m2Amax
5. Solving for maximum amplitude:
Dividing both sides by m2 (assuming m2≠0):
μg=km1+m2Amax
Rearranging gives:
Amax=μg(m1+m2)k
Final Results
- The period of oscillation T is:
T=2π√m1+m2k
- The maximum oscillation amplitude Amax is:
Amax=μg(m1+m2)k
The total mass M of the system when both blocks move together is:
M=m1+m2
The period T of oscillation for a mass-spring system is given by:
T=2π√Mk
Substituting the total mass into the formula:
T=2π√m1+m2k
The maximum static friction force Fmax that can act between the two blocks is given by:
Fmax=μm2g
where μ is the coefficient of static friction, m2 is the mass of the top block, and g is the acceleration due to gravity.
The maximum force acting on the top block due to the oscillation is related to the maximum acceleration amax of the oscillating system:
Fmax=m2amax
The maximum acceleration can also be expressed in terms of angular frequency ω:
amax=ω2Amax
where Amax is the maximum amplitude.
The angular frequency ω is given by:
ω=√kM=√km1+m2
Therefore, the maximum acceleration becomes:
amax=(√km1+m2)2Amax=km1+m2Amax
Equating the two expressions for the maximum force:
μm2g=m2km1+m2Amax
Dividing both sides by m2 (assuming m2≠0):
μg=km1+m2Amax
Rearranging gives:
Amax=μg(m1+m2)k
T=2π√m1+m2k
Amax=μg(m1+m2)k
Topper's Solved these Questions
Similar Questions
A block of mass M=1 kg resting on a smooth horizontal surface is connected to a horizontal light spring of spring constant k=6N/m whose other end is fixed to a verticall wall. Another block of mass m=0.5 kg is placed over the block M. If the coefficient of friction between the two blocks μ=0.4, find the maximum kinetic energy (in J) that the system can have for simple hormonic oscillation under the action of the spring.
View SolutionFind the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is μ1 and that between the bigger block and the ground is μ2.
View Solution
Knowledge Check
Two blocks of mass m1 and m2, resting on a frictionless table, are connected by a stretched spring and then released -
Aratio of speed of blocks is m2/m1Bratio of kinetic energy of blocks is m2/m1Ccentre of mass will move towards heavier blockDmechanical energy will remain conserve in this processA block of mass m is placed at rest on an inclination θ to the horizontal.If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
AmgBμmgcosθCmgsinθDμmgtanθA block of mass m is placed on the top of another block of mass M as shown in the figure. The coefficient of friction between them is μ.

The maximum acceleration with which the block M may move so that m also moves along with it isAμgBμMmgCμmMgDgμ
A block A of mass m1 is placed on a horizontal frictionless table. It is connected to one end of a light spring of force constant k whose other end is fixed to a wall. A small block B of mass m2 is placed on block A. The coefficient of static friction between the blocks is μ. The system is displaced slightly from its equilibrium position and released. What is the maximum amplitude of the resulting simple harmonic motion of the system so that the upper block does not slip over the lower block ?
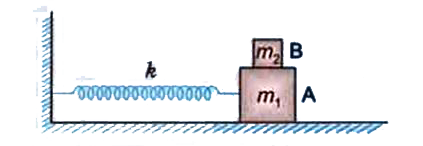
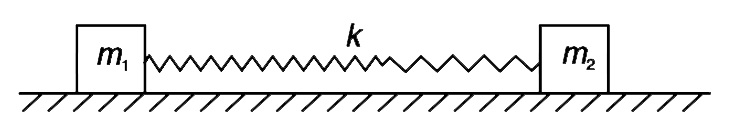
View SolutionTwo blocks rest on a smooth horizontal surface. They are connected by a spring of force constant k. If the system is set into oscillation find its time period.
View SolutionA block of mass M is being pulley along horizontal surface .The coefficient of friction the block and the surface is μ If another block of mass M/2 is placed on the block and it is pulled is again pulled on the surface , the coefficient of friction the block and the surface will be
View SolutionThe block of mass M moving on the frictionless horizontal surface collides with the spring constant k and compresses it by length L . The maximum momention of the block after collision is

View SolutionA block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?

View Solution
