Random variable X takes the integral values from 1 to 100 having equal probability. Then find E(X), E(X2) and Var(X).
Text Solution
The correct Answer is:1012,67672,33334
|
Topper's Solved these Questions
- PROBABILITYBOOK - KUMAR PRAKASHANCHAPTER - PROBABILITYEXERCISE - EXERCISE -13.1
23 Videos
- PROBABILITYBOOK - KUMAR PRAKASHANCHAPTER - PROBABILITYEXERCISE - EXERCISE -13.2
30 Videos
- MATRICES BOOK - KUMAR PRAKASHANCHAPTER - MATRICES EXERCISE - Practice Paper - 3 (Section - D)
1 Videos
- RELATIONS AND FUNCTIONS BOOK - KUMAR PRAKASHANCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Practice Paper - 1 (Section - D)
2 Videos
Similar Questions
Knowledge Check
A random variable X takes values 0, 1, 2, 3,… with probability proportional to (x+1)(15)x.
P(X≥2) equalsA1125B1325C11125D13125A r.v. X assumes values 1, 2, 3, …, n with equal probabilities. If Var (X) = E (X), then n =
A11B5C6D7A r.v. X assumes values 1, 2, 3, …, n with equal probabilities. If Var (X) = 4 E (X), then n =
A49B23C25D24
A random variable X has the following probability distribution:
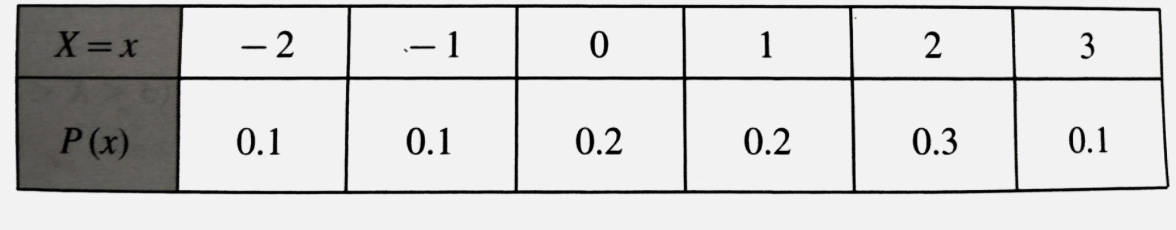
Then E(X) = …….
View SolutionIf a random variable "X" takes values (−1)k2kk,k=1,2,3,... with probabilities P(X=k)=12k, then E(X)
View SolutionThe random variable X can take only the values 0,1,2. Given that P(X=0)=P(X=1)=p and that E(X2)=E(X); find the value of p.
View SolutionThe random variable X can take only the values 0, 1, 2. Given that P(X=0) = P(X= 1) = p and E(X2)=E(X) , then find the value of p
View SolutionThe random variable X can take only the values 0;1;2. Giventhat P(X=0)=P(X=1)=p and that E(X2)=E(X); find the value of p.
View Solution

