Consider a hypothetical planet which is very long and cylinderical. The density of the planet is , its radius is .
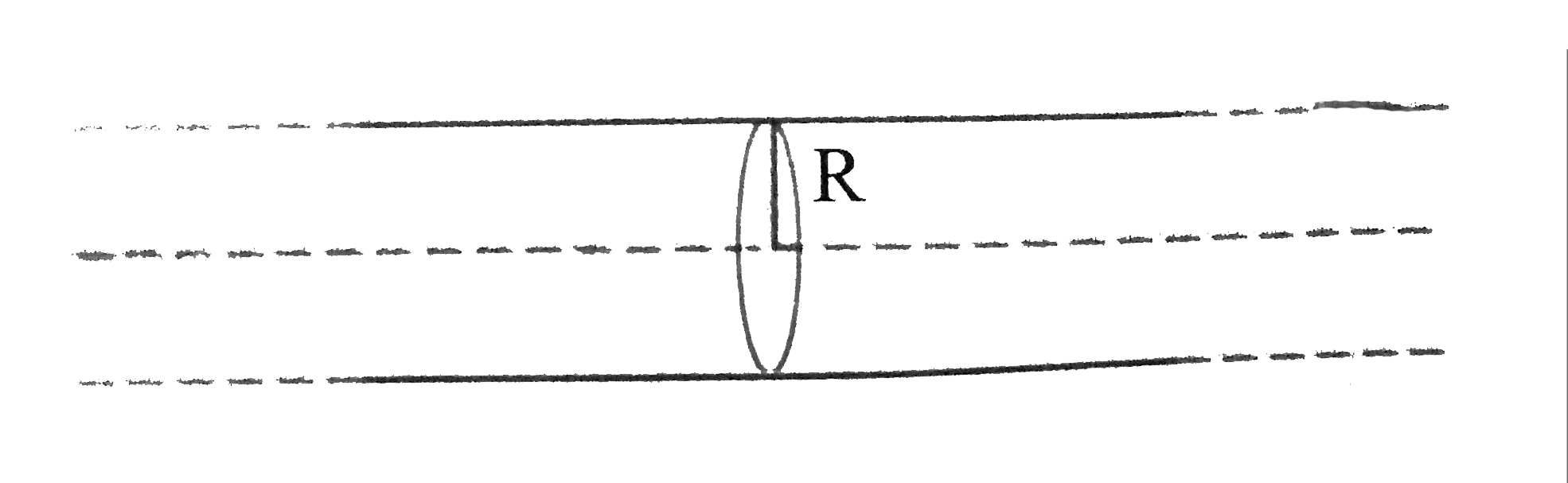
What is the possible orbital speed of the satellite in moving around the planet in circular orbit in a plane which is perpendicular to the axis of planet?Solution in Marathi
The correct Answer is:B
Step by step video & image solution for Consider a hypothetical planet which is very long and cylinderical. The density of the planet is rho, its radius is R. What is the possible orbital speed of the satellite in moving around the planet in circular orbit in a plane which is perpendicular to the axis of planet? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
m द्रव्यमान का एक उपग्रह,M द्रव्यमान के ग्रह के चारों ओर R त्रिज्या की वृत्तीय कक्षा में घूम रहा है, तो इसकी कुल ऊर्जा E होगी :
View SolutionA satellite is in a circular orbit around a planet . Its period of revolution is T, radius of the orbit is R, orbital velocity V and acceleration .a. , then
View SolutionA satellite is in a circular orbit around a planet . Its period of revolution is T, radius of the orbit is R, orbital velocity V and acceleration .a. , then
View SolutionA satellite is in a circular orbit around a planet. Its period of revolution is T, radius of the orbit is R, orbital velocity V and acceleration 'a', then
View SolutionA satellite is in a circular orbit around a planet. Its period of revolution is T, radius of the orbit is R, orbital velocity V and acceleration 'a', then
View Solutionएक ग्रह के चारो और वृत्तीय कक्षा में घुमने वाले उपग्रह का आवर्तकाल निर्भर नहीं करता है
View SolutionAssume that the earth moves around the sun in circular orbit of radius R and there exists a planet which also moves around the sun in a circular orbit with an radius of the orbit of the planet is
View SolutionAssume that the earth moves around the sun in a circular orbit of radius R and there exists a planet which also moves around the sun in a circular orbit with an angular speed twice as large as that of the earth. The radius of the orbit of the planet is
View Solution
