दो संकेन्द्रीय गोलीय कोशों के द्रव्यमान क्रमशः m1 व m2 तथा त्रिज्यायें r1 व r2(r2>r1) हैं। इस निकाय द्वारा द्रव्यमान m3 के कण पर बल क्या होगा, यदि कण केन् से दूरी r(r1<r<r2) पर रखा हो?
बाहरी कोश द्वारा बिन्दु ? पर कोई प्रभाव नहीं डाला जायेगा।
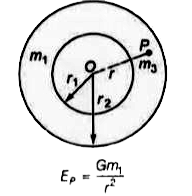
अतः P पर स्थित द्रव्यमान m3 पर बल,
F=(m3Ep) या F=Gm1m3r2
क्षेत्र Ep तथा F दोनों O की ओर हैं।
Similar Questions
Two satellites of masses m1andm2(m1>m2) are moving around the earth in orbits of radii r1andr2(r1>r2). Which one of the following statements about their velocities is correct?
02:10View SolutionPosition vector of centre of two particles of masses m1 and m2 whose position vectors r1 and r2 then rcm?
01:28View SolutionTwo planets of radii R1 and R2 have masses m1 and M2 such that M1M2=1g. The weight of an object on these planets is w1 and w2 such that w1w2=49. The ratio R1R2
03:32View SolutionThe distance between two particles of masses m1andm2 is r. If the distance of these particles from the centre of mass of the system are r1andr2 respectively, then show that r1=r(m2m1+m2)andr2=r(m1m1+m2)
02:55View SolutionTwo satellites of masses m_(1) and m_(2) (m_(1) gt m_(2)) are revolving the earth in circular orbits of radius r_(1) and r_(2)(r_(1) gt r_(2)) respectively. Which of the following statement is true regarding their velocities v_(1) and v_(2) .
View SolutionTwo satellites of masses m1 and m2(m1>m2) are revolving the earth in circular orbits of radius r1 and r2(r1>r2) respectively. Which of the following statement is true regarding their velocities v1 and v2.
View Solutionमान लीजिये r1 और r2 पर स्थित दो द्रव्यमानो m1 तथा m2 के बीच गुरुत्वाकर्षण बलों F1 तथा F2 के लिए न्यूटन के गुरुत्वाकर्षण नियम को F1=−F2=−r12r312GM20(m1m2M20)n द्वारा व्यक्त किया जाता है। यहाँ M0 एक स्थिरांक है जिसकी विमा द्रव्यमान की है। r12=r1−r2 तथा n कोई संख्या है। ऐसे प्रकरण में
08:35View Solution

