A particle travels from point A to B on a circular path of radius 15/πcm. If the arc length AB be 10 cm , find the displacement (Figure)
Text Solution
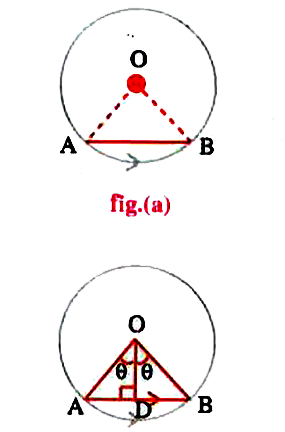
Joining the initial (A) and final (B) positions of the body, the straight line AB is obtained which is its displacement (Figure)
We know ,
Circumferencearc length=360∘Angle subtended by are at centre
∴2π×15π10=360∘20
⇒2θ=120∘. ⇒θ60∘
Now from right angle ΔADO,
AD=OAsinθ=(15π)sin60∘=15√32π
∴AB=2AD=15√3πcm along A to B
Note,
Distance is scalar, but displacement is a vector quantiy.
Distance ≥ displacement
If a particle stars from a point and reaches the same point at the end of its journey, then displacement is zero. However distance covered is not zero .
The magnitude of displacement cannot exceed the distance of a particle in motion .
|
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINEBOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Very Short Answer Type Questions
15 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - EXERCISE (LEVEL 1)
22 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH SERIESCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - Exercise -3
31 Videos
- MOTION IN A PLANE BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANE EXERCISE - Practice Exercise
84 Videos
- NEWTONS LAWS OF MOTION BOOK - AAKASH SERIESCHAPTER - NEWTONS LAWS OF MOTION EXERCISE - ADDITIONAL PRACTICE EXERCISE PRACTICE SHEET (ADVANECED) (Matrix Matching Type Questions)
1 Videos
Similar Questions
Knowledge Check
A particle travels from A to B path shown in figure, then the displacement of particle is :
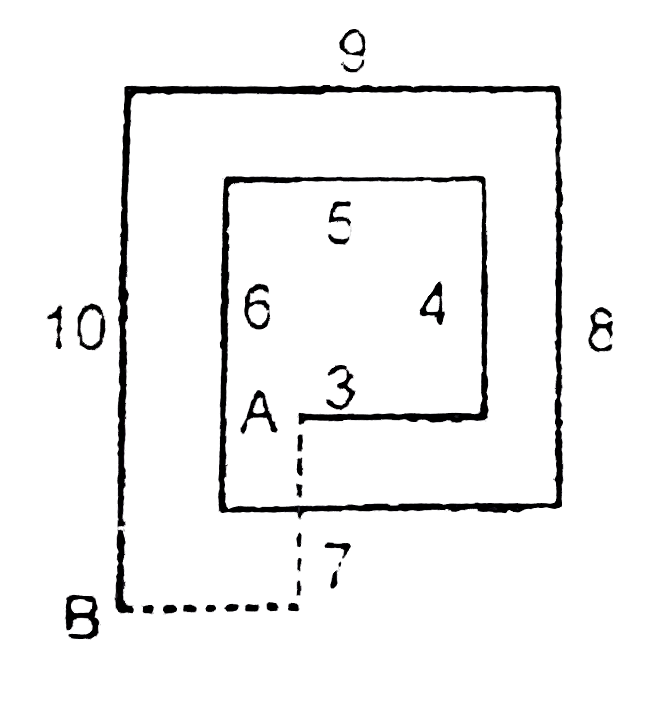 .A2√2mB4√2mC52mDnone of these
.A2√2mB4√2mC52mDnone of theseA particle moves from A to B in a circular path of radius R covering an angle θ as shown in figure. Find the ratio of magnitude of distance and magnitude of displacement of the particle.
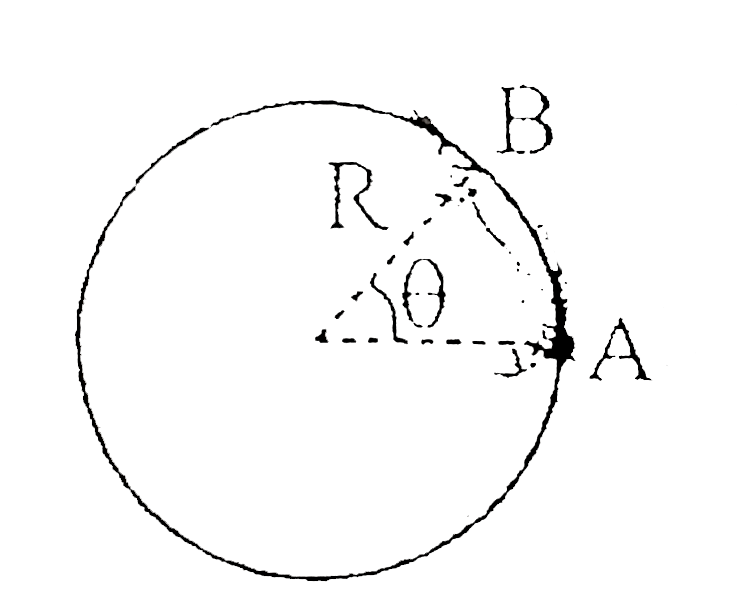 Aθ2.sinθBθ2sin.θ2Cθ2sinθDθsin.θ2
Aθ2.sinθBθ2sin.θ2Cθ2sinθDθsin.θ2A particle moves through angular displacement θ on a circular path of radius' r'. The linear displacement will be:
A2rsin(θ2)B2rcos(θ2)C2rtan(θ2)D2rcot(θ2)
A particle completes 1.5 revolutions in a circular path of radius 2 cm. The angular displacement of the particle will be – (in radian)
View SolutionFind the area of the sector of a circle of radius 8 cm and arc with length 15 cm.
View SolutionA particle is movingin circular path of radius 7 cm. Find the ratio of its displacement to the distance covered after the completion of a half
View SolutionA particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B

View SolutionA particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B

View Solution
