Born Haber Cycle
Step by step video solution for Born Haber Cycle by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Types OF Solvent - Polar-non Polar || Hydration and HE || Application OF HE-Size || Ionic Mobility || Conductivity || Lattice Energy || Solubility OF Ionic Compound || Born-Haber Cycle || Hydrides-metallic and non-metallic || Composition OF Acidic Strength
View SolutionProve that the formation of NaCI2 is highly endothermic and energetically unfavorable from the following data using Born-Haber Cycle
Heat of sublimation ofNa=109kJ/mol1stIEofNa=494kJ/mol1stIEofNa+=4563kJ/molBond Energy ofCI2=242kJ/molElectron gain enthalpy ofCI=−347kJ/molLattice energy ofNaCI2=2560kJ/molView Solution
Knowledge Check
- Question 1 - Select One
Which of the following type of energies are involved in Born Haber's cycle?
AΔsubHBIonisation enegryCBond dissociation enegryDLattice enegry - Question 2 - Select One
Born-Haber cycle is used to determine
AElectrongativelyBLattice energyCBoth the theseDNone of these - Question 3 - Select One
Born-Harber cycle is used to determine:
ALattice energyBElectron affinityCCrystal energyDAll of these
Calculate the lattice enthalpy of KCl from the following data by Born- Haber's Cycle.
Enthalpy of sublimation of K=89kJmol–1
Enthalpy of dissociation of Cl=244kJmol–1
Ionization enthalpy of potassium = 425kJmol–1
Electron gain enthalpy of chlorine = –355kJmol–1
Enthalpy of formation of KCl=–438kJmol−1View SolutionCalculate the lattice energy of NaCI crystal from the following data by the use of Born-Haber cycle. Sublimation energy of Na=26kcal/gatom, dissociation energy of CI2=54kcal/mole, ionisation energy of Na(g)=117kcal/mol, electron affinity for CI(g)=84kcal/g atom, heat of formation of NaCI=−99kcal/mole.
View SolutionCalculate value of -U/100, for AB(s), from following data of Born-Haber's cycle. [where U is lattice energy in kJ/mol]
 View Solution
View SolutionHow many steps are involved in the Born-Harber cycle for sodium chloride?
View SolutionConsider the following Born-Haber's cycle:
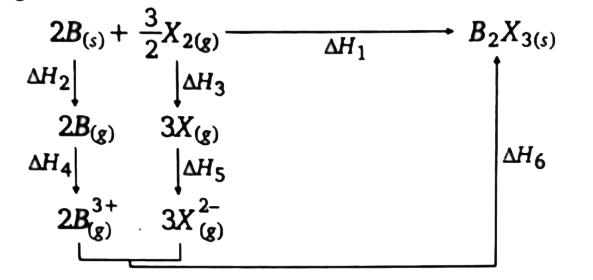
(Where ΔH1,ΔH2,ΔH3,ΔH4,ΔH5andΔH6 are in kJ/mol)
Then according to given information the correct statement is/are:View Solution

