Secants containing chords RS and PQ of a circle intersect each other in point A in the exterior of a circle. If m(arcPCR)=26∘ and m(arcQDS)=48∘, then find m∠SPQ
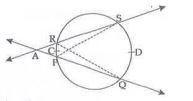 Solution in Marathi
Solution in Marathi
Step by step video & image solution for Secants containing chords RS and PQ of a circle intersect each other in point A in the exterior of a circle. If m(arc PCR)=26^@ and m(arc QDS)=48^@, then find m/_SPQ by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two circles of radii 5.5 cm and 3.3 cm respectively touch each other.What is the distance between their centres?
View SolutionRewrite the following statement in 'if-then' form. 'Diagonals of a parallelogram bisect each other.'
View SolutionSeg AB is parallel to Y-axis and co-ordinates of point A are (1,3), then co-ordinates of point B can be……….a) (3,1) b) (5,3) c) (3,0) d) (1,-3)
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionFind the area of the shaded region, given that the radius of each circle is equal to 5 cm.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View SolutionThe inequation that best describes the following graph is _________
View SolutionIn fig. O is the center of the circle. Find the value of x.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_S01_031_Q01.png" width="80%"gt
View SolutionIn the following figure, if O is the center of the circle and ¯¯¯¯¯¯AB is the diameter, then find ∠ACB.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C12_E02_009_Q01.png" width="80%"gt
View SolutionConsider a point M inside a quadrillated PQRS. If M be the point of intersection of angle bisectors PE and QF, then _____
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C15_E04_011_Q01.png" width="80%"gt
View SolutionA circle with centre 'O' . Chord PQ≅ chord RS . M(arc PXQ) =260∘ . Then find m (arc RXS).
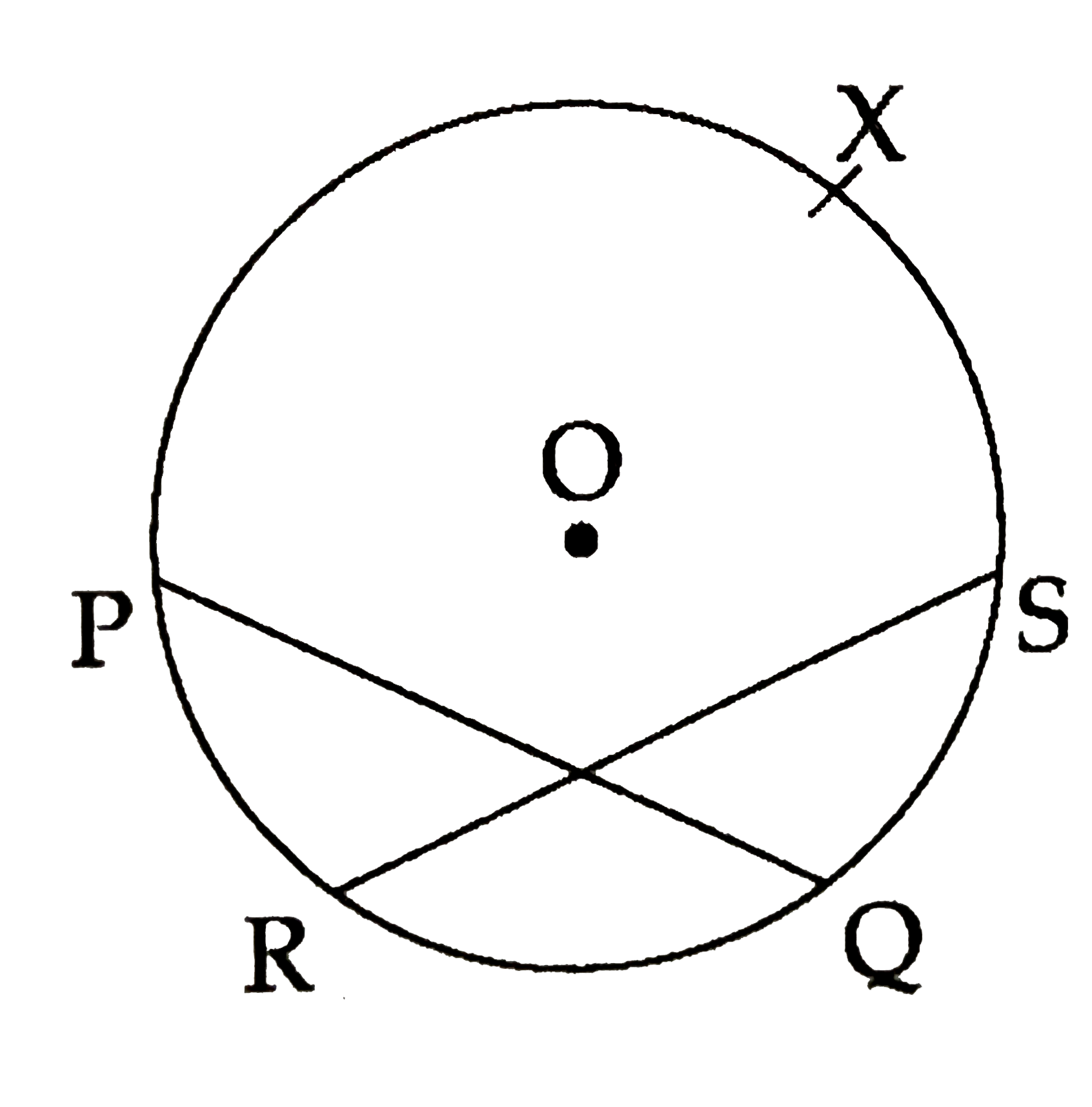
View Solution
