An open box is to be cut of piece of square card board of side 18 cm
by cutting of equal squares from the corners and turning up the sides.
Find the maximum volume of the box.Solution in Marathi
Step by step video & image solution for An open box is to be cut of piece of square card board of side 18 cm by cutting of equal squares from the corners and turning up the sides. Find the maximum volume of the box. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- Applications of DerivativesBook:CHETANA PUBLICATIONChapter:Applications of DerivativesExercise:Exercise
Explore 144 Videos
- APPLICATION OF DEFINITE INTEGRATIONBook:CHETANA PUBLICATIONChapter:APPLICATION OF DEFINITE INTEGRATIONExercise:EXERCISE
Explore 80 Videos
- BINOMIAL DISTRIBUTIONBook:CHETANA PUBLICATIONChapter:BINOMIAL DISTRIBUTIONExercise:EXERCISE
Explore 108 Videos
Similar Questions
Find the equation of tangent and normal to the curve at the given point on it.: y=2x3−x2+2 at (12,2)
View SolutionFind the area of the region bounded by the following curves,X-axis and the given lines.(iv)y=sin x,x=0,x=π2

View SolutionA die is thrown 6 times.If 'getting an odd number' is a success,find the probability of (i)5 sucesses
View SolutionA box with open top is made from a square piece of metal sheet of side 24cm by cutting equal small squares from each comer and turning up the edges If the volume of the box is maximum then the dimensions of the box are:
View SolutionA box without lid having maximum volume is made out of square metal sheet of edge60cms by cutting equal square pieces from the four corners and turning up the projecting pieces to make the sides of the box.The height of the box is
View SolutionAn open box is to be made out of a piece of cardboard measuring (24cm×24cm) by cutting off equal square from the corners and turning up the sides. Find the height of the box when it has maximum volume.
View SolutionAn open box is to be made out of a piece of a square card board of sides 18 cm by cutting off equal squares from the corners and turning up the sides. Find the maximum volume of the box.
View SolutionA box of maximum volume with top open is to be made by cutting out four equal squares from four corners of a square tin sheet of side length a feet and then folding up the flaps. Find the side of the square cut-off.
View SolutionA box is to be made from a sheet 12×12 sq.cm, by cutting equals squares from the four corners and turning up its sides. Find the length of the side of the square to be cut out, in order to obtain a box of the largest possible volume?
View Solutionटीन का एक वर्गाकार टुकड़ा जिसकी भुजा 18 cm है, के कोनो से वर्गाकार भाग काटकर खुले मुख का बॉक्स बनाया जाता है | कटे हुए वर्ग की भुजा क्या होनी चाहिए ताकि बॉक्स का आयतन महत्तम हो |
View Solutionटीन के एक वर्गाकार टुकड़े की भुजा 24 cm है | इससे एक खुला बॉक्स बनाया जाता है | इसके प्रत्येक कोने से एक वर्गाकार टुकड़ा काटा जाता है और बॉक्स बनाया जाता है | बॉक्स का महत्तम आयतन ज्ञात कीजिए |
View Solutionटीन का एक वर्गाकार टुकड़ा जिसकी भुजा 12 cm है, से एक खुला बॉक्स बनाया जाता है, इस टुकड़े के चारों कोनो से एक वर्गाकार भाग काटकर एक बॉक्स बनाया गया है | बॉक्स का अधिकतम आयतन ज्ञात कीजिए |
View SolutionAn open box is to be made from a square piece of material, 24 cm on a side, by cutting equal squares from the corners and turning up the sides as shown in figure. Express volume V of the box as a function of x.
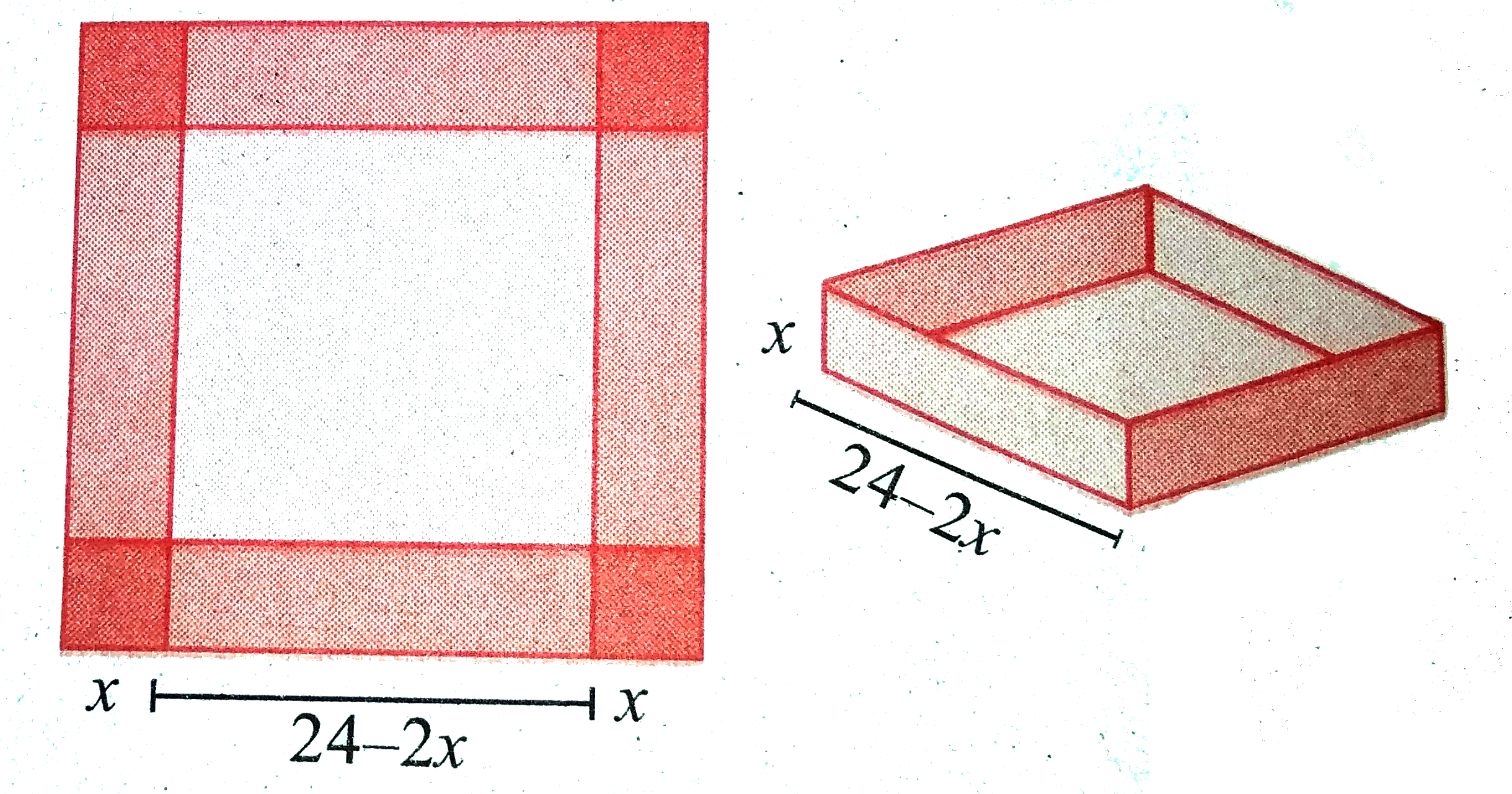
View SolutionAn open top box of maximum possible volume from a square piece of tin of side 'a' is to be made by cutting equal squares out of the corners and then folding up the tin to form the sides. The length of a side of square cut out is
View SolutionAn open box of maximum volume is made from a square piece of tin of side a' by cutting for four equal square pieces from four corners and folding up the tin then length of square cut is 'a'6
View SolutionA box of maximum volume with top open is to be made by cutting out four equal squares from four corners of a square tin sheet of side length a feet and then folding up the flaps. Find the side of the square cut-off.
View Solution