Two wires of same length and thickness having specific resistance cm respectively are connected in parallel. The effective resistivity is . The value of p, to the nearest integer, is ...........
To solve the problem of finding the effective resistivity p of two wires connected in parallel, we can follow these steps:
Step 1: Understand the Given Data
We have two wires with specific resistances:
- Wire 1: ρ1=6Ωcm
- Wire 2: ρ2=3Ωcm
Both wires have the same length L and the same cross-sectional area A.
Step 2: Calculate the Resistance of Each Wire
The resistance R of a wire can be calculated using the formula:
R=ρLA
Since the lengths and cross-sectional areas are the same for both wires, we can express the resistances as:
- Resistance of Wire 1:
R1=6LA
- Resistance of Wire 2:
R2=3LA
Step 3: Calculate the Equivalent Resistance in Parallel
For two resistors in parallel, the equivalent resistance Req is given by:
1Req=1R1+1R2
Substituting the values of R1 and R2:
1Req=A6L+A3L
Finding a common denominator:
1Req=A6L+2A6L=3A6L=A2L
Thus, the equivalent resistance Req is:
Req=2LA
Step 4: Calculate the Effective Resistivity p
The effective resistivity p can be calculated using the formula for resistance:
Req=pLA
Setting the two expressions for Req equal to each other:
pLA=2LA
Cancelling L and A (assuming they are not zero):
p=2
Step 5: Round to the Nearest Integer
Since the problem asks for the value of p to the nearest integer, we find that:
p≈2
Thus, the final answer is:
2
We have two wires with specific resistances:
- Wire 1: ρ1=6Ωcm
- Wire 2: ρ2=3Ωcm
The resistance R of a wire can be calculated using the formula:
R=ρLA
Since the lengths and cross-sectional areas are the same for both wires, we can express the resistances as:
- Resistance of Wire 1:
R1=6LA
- Resistance of Wire 2:
R2=3LA
For two resistors in parallel, the equivalent resistance Req is given by:
1Req=1R1+1R2
Substituting the values of R1 and R2:
1Req=A6L+A3L
Finding a common denominator:
1Req=A6L+2A6L=3A6L=A2L
Thus, the equivalent resistance Req is:
Req=2LA
The effective resistivity p can be calculated using the formula for resistance:
Req=pLA
Setting the two expressions for Req equal to each other:
pLA=2LA
Cancelling L and A (assuming they are not zero):
p=2
Since the problem asks for the value of p to the nearest integer, we find that:
p≈2
2
Similar Questions
A wire of 36Omega resistance,is cut into 'n' equal pieces and are joined in parallel.The effective resistance is 1Omega .The value of 'n' is
View SolutionTwo cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View Solution
Knowledge Check
Two wires of resistance 10Ω and 5Ω are connected in series. The effective resistance is __________ Omega.
A15B20C30D40If Four resistors each of 1Ω are connected in parallel the effective resistances will be
A0.5ΩB0.25ΩC4ΩD2ΩA wire has a resistance of 6Ω. It is cut into two parts and both half values are connected in parallel. The new resistance is ....
A12ΩB1.5ΩC3ΩD6Ω
Two cells of emfs 1.5 V and 2.0 V having internal resistances 0.2Ω and 0.3Ω respectively are connected in parallel. Calculate the emf and internal resistance of the equivalent cell.
View SolutionTwo cells, of voltage 10 V and 2 V and internal resistances 10Ω and 5Ω respectively, are connected in parallel as shown in Fig. Find the effective voltage and effective resistance of the combination.
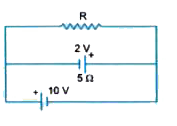
View SolutionYou have two metallic wires of resistances 6Ωand3Ω. How will you connect these wires to get the effective resistance of 2Ω?
View SolutionHow can the resistances of 2Ω,3Ω and 6Ω be connected to give an effective resistance of 4Ω ?
View SolutionA set of n identical resistors, each of resistance RΩ, when connected in series have an effective resistance XΩ and when the resistors are connected in parallel, their effective resistance is YΩ. Find the relation between R, X and Y.
View Solution
