Which set can be the universal set for above sets? Solution in Marathi
Step by step video & image solution for Which set can be the universal set for above sets? by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
Similar Questions
Choose the correct alternative answer for each of the following questions : M = {1, 3, 5}, N = {2, 4, 6,}, then M∩N=?
01:05View SolutionWhich one of the following is an irrational number?
03:03View SolutionWhich of the following data is not primary?
02:57View SolutionIf the sets A={1,3,5},B={2,4,6} and C={0,2,4,6,8}. Then, the universal set of all the three sets A, B and C can be ………
04:53View SolutionDistinguish :
Null set and universal set44:48View SolutionDistinguish :
Universal set and complement of a set01:38View SolutionP is the set of all residents in Pune. M is the set of all residents in Madhya Pradesh. I is the set of all residents in Indore. B is the set of all residents in India. H is the set of all residents in Maharashtra. (i) Write the subset relation between the sets. (ii) Which set can be the universal set for above sets ?
01:49View SolutionRevision|Set#!#Subset#!#Power Set#!#Universal Set
01:39View SolutionIf the sets A={1,3,5},B={2,4,6} and C={0,2,4,6,8}. Then, the universal set of all the three sets A, B and C can be ………
01:20View SolutionIf I is a universal set, then write the complement of the set N.
03:45View SolutionFind set A,B and Universal set U.
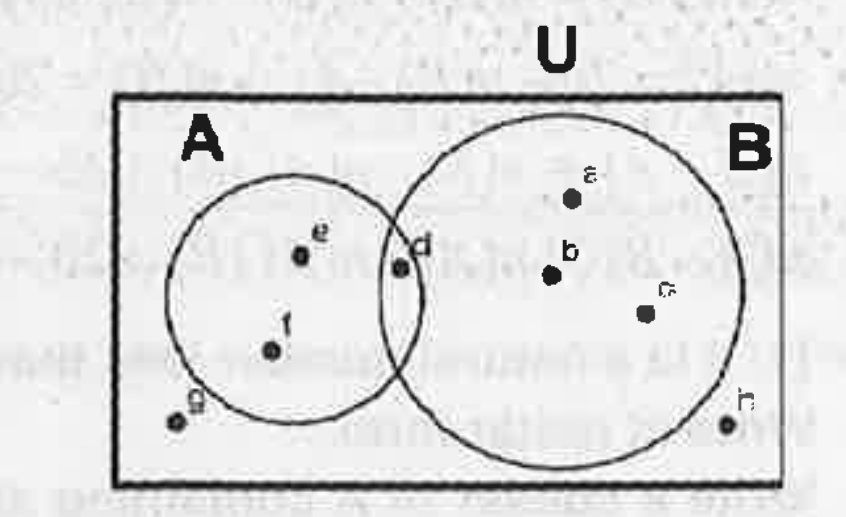 03:57View Solution
03:57View SolutionIf U is the universal set and A is any set U⋂A
50:52View SolutionWhich set of numbers could be the universal set for the sets given below:
A = set of multiples of 5,
B = set of multiples of 7,
C = set of multiples of 12.48:25View SolutionP हा पुण्यातील रहिवाशांचा संच आहे.
M हा मध्यप्रदेशातील रहिवाशांचा संच आहे.
I हा इंदौरमधील रहिवाशांचा संच आहे.
B हा भारतातील रहिवाशांचा संच आहे.
H हा महाराष्ट्रातील रहिवाशाचा संच आहे.
वरील पैकी कोणता संच या उदाहरणात विश्र्वसंच म्हणून घेता येईल.View SolutionUniversal Set#!#Venn Diagram#!#Operations On Set
View SolutionSets#!#Subset, Superset, Universal Set, Power Set
41:00View Solution

