Are the following sets relation ? ϕ from A to B . Determine the domain range and inverse of each of the relations mentioned above.Solution in Odia
ϕ from A to B is a relation.
Topper's Solved these Questions
Similar Questions
If A-{a,b,c,d) mention the type of relations on A given below, which of them are equivalence relations?
{(a, a),(b,b)}
View SolutionWhich of the following is a sequence ?
View SolutionDomain and range of a inverse relation
View SolutionA relation R is shown from sets P to Q in the following diagram:

write this relation in Roaster form and in Set builder form. Also find the domain, co-domain and range of the relation.
View SolutionLet A = { a,b,c }, |B| = {1,2} Determine all the relations from A to B and determine the domain range and inverse of each relation.
View SolutionAre the following sets relation ? A×B from A to B.Determine the domain and range of each of the relations mentioned above.
View SolutionAre the following sets relation ? A×ϕ from A to ϕ. Determine the domain range and inverse of each of the relations mentioned above.
View SolutionAre the following sets relation ? ϕ * B from ϕ to B.
View SolutionAre the following sets relation ? ϕ×ϕ from ϕ to ϕ. Determine the domain range and inverse of each of the relations mentioned above.
View SolutionAre the following sets relation ? ϕ x C from A to B . Determine the domain range and inverse of each of the relations mentioned above.
View SolutionAre the following sets relation ? ϕ×ϕ from A to B. Determine the domain range and inverse of each of the relations mentioned above.
View SolutionDefination || Domain,Range & Inverse OF Relation
View SolutionDetermine the domain and range of the following relations : {(−3,1),(−1,1),(1,0),(3,0)}
View SolutionA relation R is represent from set P to Q in the following diagram:
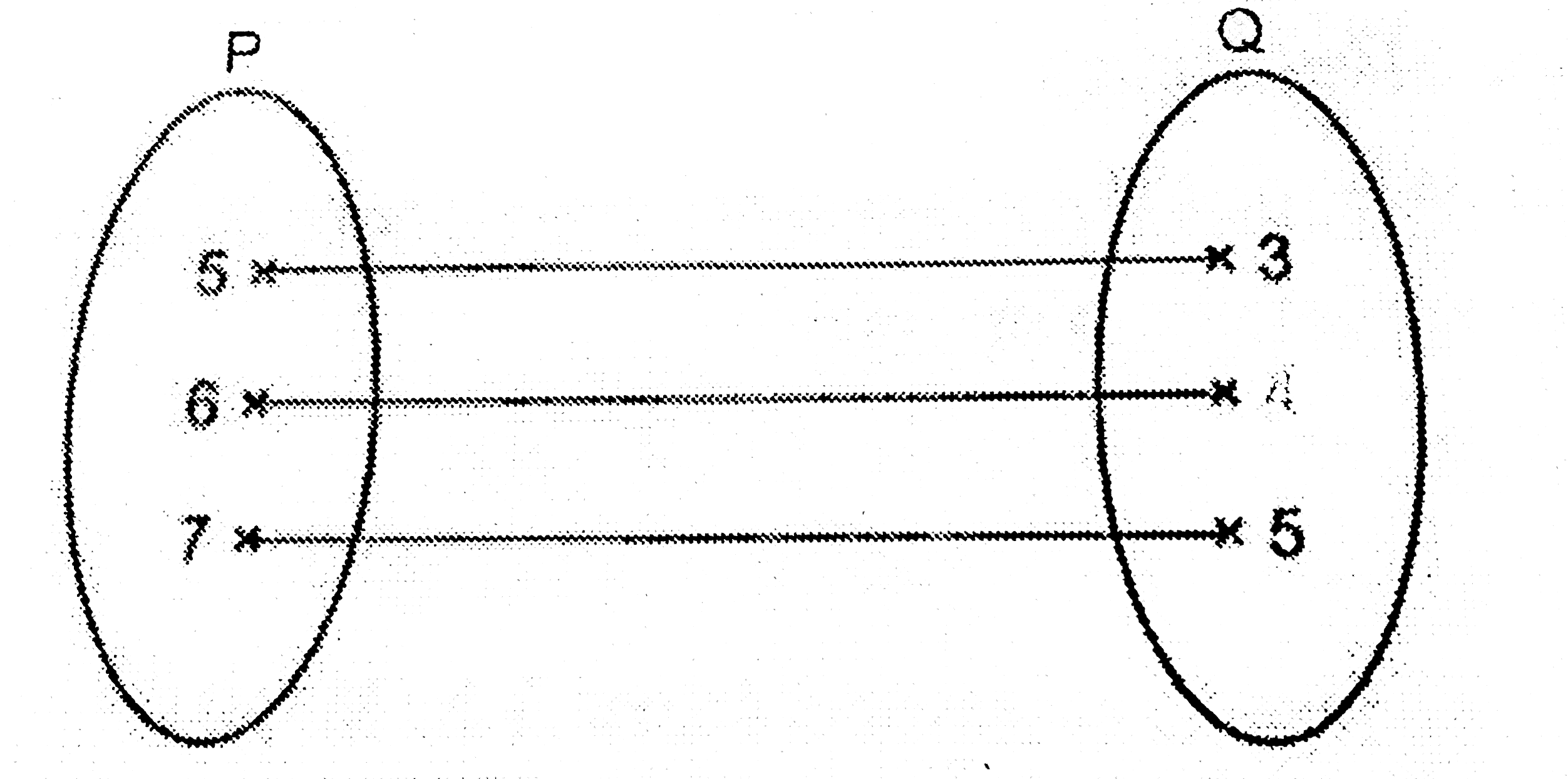
Write it in Roaster and Set builder form. Write the domain and range of the relation.
View SolutionA relation R is shown from sets P to Q in the following diagram:

write this relation in Roaster form and in Set builder form. Also find the domain, co-domain and range of the relation.
View Solution