The latus rectum of the parabola y2=−8x is 2.Solution in Odia
Step by step video & image solution for The latus rectum of the parabola y^2=-8x is 2. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Multiply (2√−3+3√−2) by (4√−3−5√−2)
View SolutionFind the points where the following function are not differentiable.
e|x|
View SolutionFind the vertex , focus, axis, directrix and latus rectum of the following parabola: y2=8x
View SolutionFind the equation of a line joining the vertex of parabola y2=8x to its upper end of latus rectum.
View SolutionLength of Latus Rectum of Parabola y2=10x
View SolutionThe equation of a circle passing through the vertex and the extremites of the latus rectum of the parabola y2=8x is
View SolutionThe length of the latus rectum of the parabola y2+8x−4y−4=0.
View SolutionThe length of the latus rectum of the parabola y2+8x−2y+17=0 is
View SolutionThe area (in square unit ) bounded by the parabola y2=8x and its latus rectum is -
View SolutionFind the coordinate of focus , directrix and latus rectum of parabola y2=8x ?
View SolutionFind the co-ordinate of the focus ,equation of the directrix and length of the Latus Rectum of the Parabola (y2=8x) ?
View SolutionThe latus rectum of the parabola y2=11x is of length
View SolutionFor the following parabola find the coordinates of the foci, the equation of the directrix and the lengths of the latus rectum: y2=8x
View SolutionFind the vertex , focus, axis, directrix and latus rectum of the following parabola: y2=8x
View SolutionFind the Focus, vertex and latus rectum of the parabola y2=8x.
View SolutionEquation of the parabola given in the figure is y2=8x
Find the focus and length of latus rectum of the parabola.
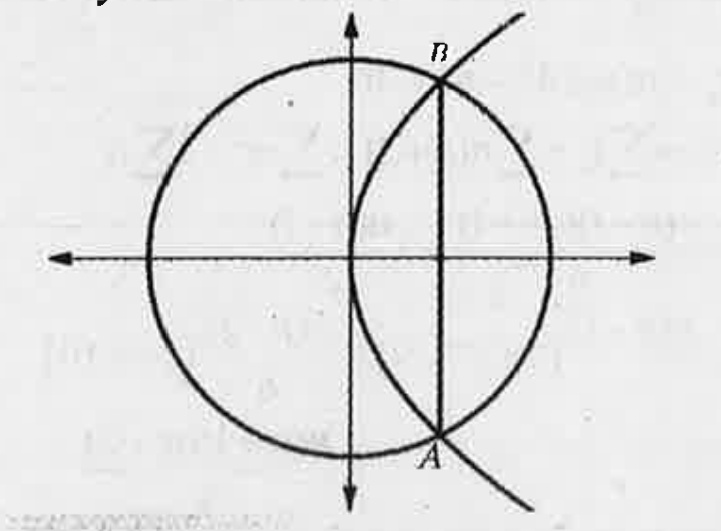 .
.
View Solution
