Assertion: A particle is rotating in a circle with constant speed as shown. Between point A and B , ratio of average acceleration and average velocity v can be stopped in a minimum distance d . If the same car, moving with same speed v takes a circular turn, then minimum safe radius can be 2d .
Reasion: d=v22μg and minimum safe radius =v2μg
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion.
If Assertion is true, true but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:B
To solve the problem, we need to analyze the assertion and reason provided, and then derive the necessary relationships between average acceleration, average velocity, and the radius of the circular path.
1. Understanding the Assertion:
- The assertion states that a particle moving in a circle with constant speed can stop in a minimum distance d when moving between points A and B. This implies that the average acceleration and average velocity have a specific relationship.
2. Average Velocity and Average Acceleration:
- The average velocity vavg can be defined as the total displacement divided by the total time taken. For a circular motion, if the particle moves from point A to point B, the average velocity can be expressed as:
vavg=ΔsΔt
- The average acceleration aavg can be defined as the change in velocity divided by the time taken:
aavg=ΔvΔt
3. Using the Stopping Distance:
- The stopping distance d can be derived from the equation of motion:
d=v22a
- Rearranging gives us:
a=v22d
4. Minimum Safe Radius:
- The reason states that the minimum safe radius R for the car taking a circular turn at speed v is given by:
R=v2μg
- Here, μ is the coefficient of friction and g is the acceleration due to gravity.
5. Connecting the Two Concepts:
- From the stopping distance equation, we have:
d=v22μg
- Therefore, if we double the stopping distance, we can express the minimum safe radius as:
R=2d=v2μg
6. Conclusion:
- Both the assertion and the reason are correct. The assertion correctly describes the relationship between average acceleration and average velocity in terms of stopping distance, and the reason correctly describes the minimum safe radius for circular motion.
Final Answer:
- Both the assertion and the reason are correct, but the reason is not the correct explanation for the assertion.
- The assertion states that a particle moving in a circle with constant speed can stop in a minimum distance d when moving between points A and B. This implies that the average acceleration and average velocity have a specific relationship.
- The average velocity vavg can be defined as the total displacement divided by the total time taken. For a circular motion, if the particle moves from point A to point B, the average velocity can be expressed as:
vavg=ΔsΔt
- The average acceleration aavg can be defined as the change in velocity divided by the time taken:
aavg=ΔvΔt
- The stopping distance d can be derived from the equation of motion:
d=v22a
- Rearranging gives us:
a=v22d
- The reason states that the minimum safe radius R for the car taking a circular turn at speed v is given by:
R=v2μg
- Here, μ is the coefficient of friction and g is the acceleration due to gravity.
- From the stopping distance equation, we have:
d=v22μg
- Therefore, if we double the stopping distance, we can express the minimum safe radius as:
R=2d=v2μg
- Both the assertion and the reason are correct. The assertion correctly describes the relationship between average acceleration and average velocity in terms of stopping distance, and the reason correctly describes the minimum safe radius for circular motion.
- Both the assertion and the reason are correct, but the reason is not the correct explanation for the assertion.
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 1 Objective
14 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Level 1 Subjective
13 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Exercise 10.3
4 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct
27 Videos
Similar Questions
Assertion: A particle is rorating in a circle with constant speed as shown. Between point A and B , ratio of average acceleration and average velocity is angular velocity of particle about point O .
Reason: Since speed is constant, angular velocity is also constant.
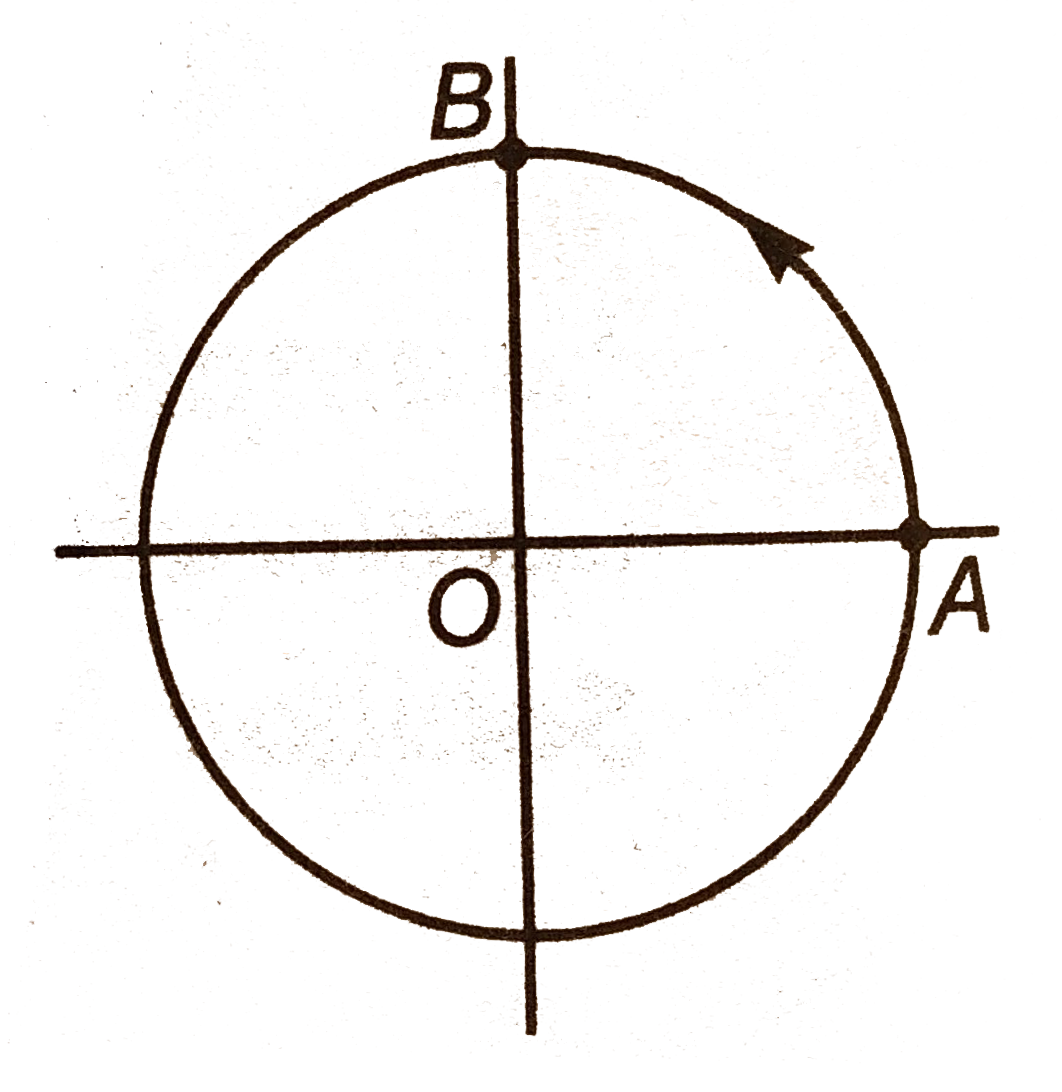
View SolutionIn sine wave , minimum distance between 2 particles always having same speed is
View Solution
Knowledge Check
A particle is moving on a circular path of radius R with constant speed v. During motion of the particle form point A to point B
 AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
AAverage speed is v/2BThe magnitude of average velocity is v/πCThe magnitude of average acceleration is 2v2πRDAverage velocity is zero.
A car moving with speed v on a straight road can be stopped with in distance d on applying brakes. If same car is moving with speed 3v and brakes provide half retardation, then car will stop after travelling distance
View SolutionA car moving with a speed of 40km/h can be stopped by applying the brakes after at least 2 m. If the same car is moving with a speed of 80km/h, what is the minimum stopping distance?
View SolutionA car moving with a speed of 40km/h can be stopped by applying the brakes after at least 2 m. If the same car is moving with a speed of 80km/h, what is the minimum stopping distance?
View SolutionA particle of mass m moves with constant speed v on a circular path of radius r. Find magnitude of average force on it in half revolution.
View SolutionA particle moves in a circle of radius R=2122m with constant speed 1m/s. Find,
(a) magnitude of average velocity and
(b) magnitude of average acceleration in 2 s.
View SolutionA particle is moving on a circular path of radius 1 m with 2 m/s. If speed starts increasing at a rate of 2m/s2, then acceleration of particle is
View SolutionA car moving with a velocity of 40 km/h can be stopped by brekes after travelling at least 5 m. If same car moves at a speed of 80 km/h the minimum stopping distance is
View Solution
