The average resisting force that must act on 5kg mass to reduce its speed from 65 to 15ms−1 in 2s is
(a) 12.5N
(b) 125N
(c) 1250N
(d) None of these
The correct Answer is:B
To solve the problem of finding the average resisting force that must act on a 5 kg mass to reduce its speed from 65 m/s to 15 m/s in 2 seconds, we can follow these steps:
Step 1: Identify the given values
- Initial velocity (u) = 65 m/s
- Final velocity (v) = 15 m/s
- Mass (m) = 5 kg
- Time (t) = 2 s
Step 2: Calculate the acceleration
We can find the acceleration (a) using the formula:
a=v−ut
Substituting the values:
a=15m/s−65m/s2s=−50m/s2s=−25m/s2
Step 3: Calculate the resisting force
The average resisting force (F) can be calculated using Newton's second law:
F=m⋅a
Substituting the values:
F=5kg⋅(−25m/s2)=−125N
Since we are interested in the magnitude of the resisting force, we take the absolute value:
F=125N
Conclusion
The average resisting force that must act on the 5 kg mass is 125 N.
---
- Initial velocity (u) = 65 m/s
- Final velocity (v) = 15 m/s
- Mass (m) = 5 kg
- Time (t) = 2 s
We can find the acceleration (a) using the formula:
a=v−ut
Substituting the values:
a=15m/s−65m/s2s=−50m/s2s=−25m/s2
The average resisting force (F) can be calculated using Newton's second law:
F=m⋅a
Substituting the values:
F=5kg⋅(−25m/s2)=−125N
Since we are interested in the magnitude of the resisting force, we take the absolute value:
F=125N
The average resisting force that must act on the 5 kg mass is 125 N.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective
32 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Subjective Questions
1 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 1 Assertion And Reason
15 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A constant force acting on a body of mass 3kg changes its speed from 2ms−1 to 3.5ms−1 in 25 s. The direction of motion of the body remains unchanged. Calculate magnitude and direction of the force.
View SolutionThe driver of a three wheeler moving with a speed of 36km/h sees a child standing in the middle of the road and brings his vehicle to rest in 4 s just in time to save the child What is the average retarding force on the vehicle ? The mass of three wheeler is 400 kg and mass of the driver is 65 kg.
View Solution
Knowledge Check
A constant force acting on a body of mass of 5 kg change its speed from 5ms−1to10ms−1 in 10 s without changing the direction of motion. The force acting on the body is
A1.5NB2NC2.5ND5N
An object of mass 10kg moves at a constant speed of 10 ms−1. A constant force, that acts for 4 sec on the object, gives it a speed of 2ms−1 in opposite direction. The force acting on the object is :
View SolutionA mass of 1 kg is acted upon by a single force F=(4ˆi+4ˆj)N. Under this force it is displaced from (0,0) to (1m,1m). If initially the speed of the particle was 2 ms−1, its final speed should be
View SolutionWhat force should be applied on a body of mass 500 kg to change its velocity from 4 ms1 to 6 ms−1 in one second ?
View SolutionA constant retarding force of 20 N acts on a body of mass 5 kg moving initially with a speed of 10 ms−1. How long does the body take to stop?
View SolutionA time varying force acts on a particle of mass 5 kg as shown in figure . Find the speed of the particle, in m/s , after 10 s , if particle was intially at rest .
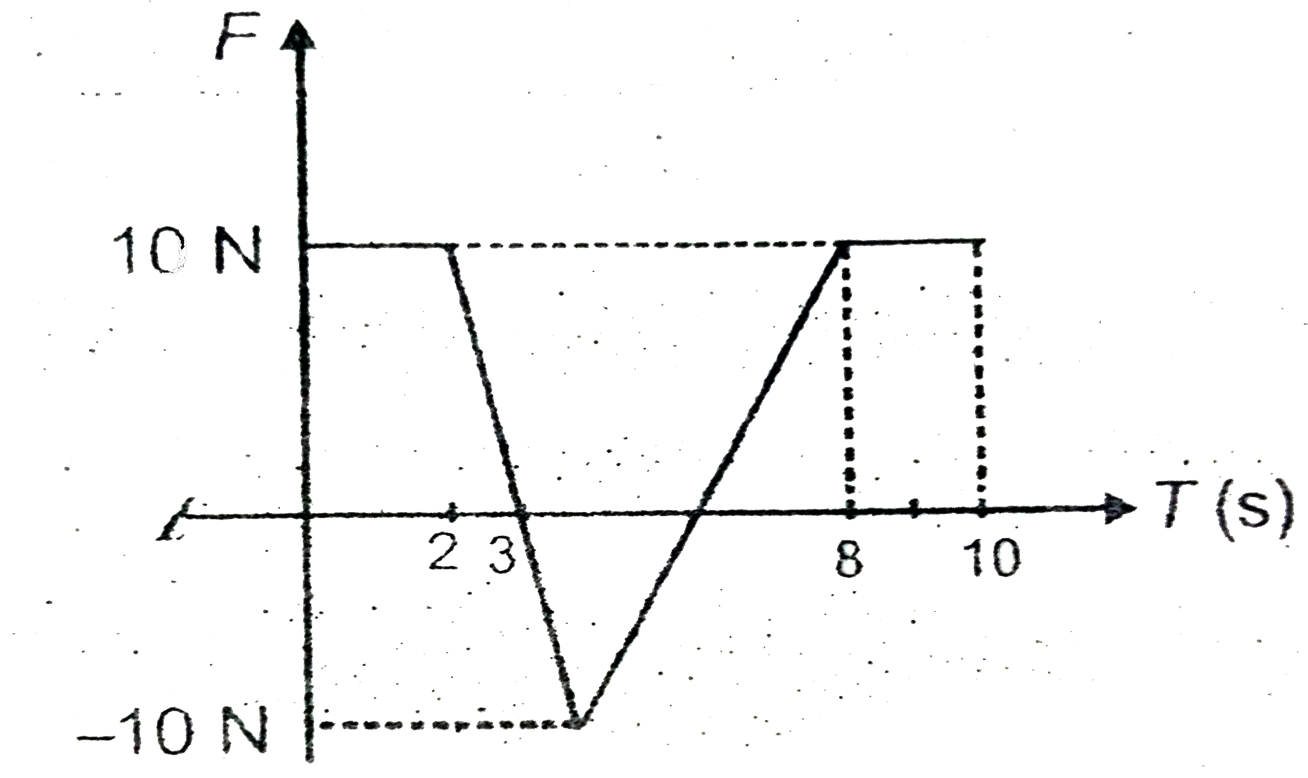
View SolutionCertain force acting on a 20 kg mass changes its velocity from 5m/s→2m/s. calculate the work done by the force.
View SolutionWhen a force 1N acts on 1kg mass at rest for 1s, its final momentum is P. When 1N force acts on 1kg mass at rest through a distance 1m, its final momentum is P1. The ratio of P to P1 is
View Solution
