Water from a tap emerges vertically downwards with an initial spped of 1.0ms−1. The cross-sectional area of the tap is 10−4m2. Assume that the pressure is constant throughout the stream of water, and that the flow is steady. The cross-sectional area of the stream 0.15 m below the tap is
5.0×10−4m2
1.0×10−4m2
5.0×10−5m2
2.0×10−5m2
The correct Answer is:B, D
To solve the problem, we will use the principles of fluid mechanics, specifically the conservation of energy and the continuity equation.
1. Identify Given Values:
- Initial speed of water, v1=1.0m/s
- Cross-sectional area of the tap, A1=10−4m2
- Height below the tap, h=0.15m
- Acceleration due to gravity, g=10m/s2 (assuming standard value)
2. Apply Conservation of Energy:
The total mechanical energy at the tap (point A) must equal the total mechanical energy at a point 0.15 m below (point B). The potential energy at point A is converted into kinetic energy at point B.
The energy equation can be written as:
PEA+KEA=PEB+KEB
Where:
- PE=ρgh (potential energy)
- KE=12ρv2 (kinetic energy)
At point A:
PEA=ρgh+12ρv21
At point B (where potential energy is zero):
PEB=0+12ρv22
Setting them equal:
ρgh+12ρv21=12ρv22
3. Simplify the Equation:
We can cancel ρ from both sides (assuming it is constant):
gh+12v21=12v22
4. Substitute Known Values:
Substitute g=10m/s2, h=0.15m, and v1=1.0m/s:
10⋅0.15+12(1.0)2=12v22
1.5+0.5=12v22
2=12v22
5. Solve for v2:
Multiply both sides by 2:
4=v22
Taking the square root:
v2=2m/s
6. Apply the Continuity Equation:
According to the continuity equation:
A1v1=A2v2
Rearranging gives:
A2=A1v1v2
7. Substitute Known Values:
A2=10−4⋅1.02=10−42=5×10−5m2
Final Answer:
The cross-sectional area of the stream 0.15 m below the tap is A2=5×10−5m2.
---
- Initial speed of water, v1=1.0m/s
- Cross-sectional area of the tap, A1=10−4m2
- Height below the tap, h=0.15m
- Acceleration due to gravity, g=10m/s2 (assuming standard value)
The total mechanical energy at the tap (point A) must equal the total mechanical energy at a point 0.15 m below (point B). The potential energy at point A is converted into kinetic energy at point B.
PEA+KEA=PEB+KEB
Where:
- PE=ρgh (potential energy)
- KE=12ρv2 (kinetic energy)
PEA=ρgh+12ρv21
At point B (where potential energy is zero):
PEB=0+12ρv22
ρgh+12ρv21=12ρv22
We can cancel ρ from both sides (assuming it is constant):
gh+12v21=12v22
Substitute g=10m/s2, h=0.15m, and v1=1.0m/s:
10⋅0.15+12(1.0)2=12v22
1.5+0.5=12v22
2=12v22
Multiply both sides by 2:
4=v22
Taking the square root:
v2=2m/s
According to the continuity equation:
A1v1=A2v2
Rearranging gives:
A2=A1v1v2
A2=10−4⋅1.02=10−42=5×10−5m2
The cross-sectional area of the stream 0.15 m below the tap is A2=5×10−5m2.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.53videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.64videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.34videos
FLUID MECHANICS
- BOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
Water from a tap emerges vertically downward with an initial speed of 3.0 m/s. The cross-sectional area of the tap is 10−4m2 . Assume that the pressure is constant throughout the stream of water and that the flow is steady. The cross-sectional area of the stream 2 m below the tap is:
View SolutionWater from a tap emerges vertically downwards with initial velocity 4ms−1. The cross-sectional area of the tap is A. The flow is steady and pressure is constant throughout the stream of water. The distance h vertically below the tap, where the cross-sectional area of the stream becomes (23)A is (g=10m/s2)
View SolutionWater from a tap emerges vertically downwards with an initial velocity V0. Assume pressure is constant throughout the stream of water and the flow is steady. Find the distance form the tap at which cross-sectional area of stream is half of the cross-sectional area of stream at the tap.
View SolutionA piston of cross-sectional area 100cm2 is used in a hydraulic pressure to exert a force of 107 dyne on the water. The cross-sectional area of the other piston which support a truck of mass 2000 kg is
View SolutionA horizontal pipeline carries water in a streamline flow. At a point along the tube where the cross sectional area is 10−2(m2), the water velcity is 2m/s and the pressure is 8000Pa. The pressure of water at another point where cross sectional area is 0.5×(10)−2(m2) is
View SolutionA horizontal pipeline carries water in a streamline flow. At a point along the pipe, where the cross- sectional area is 10cm2, the water velocity is 1ms−1 and the pressure is 2000 Pa. The pressure of water at another point where the cross-sectional area is 5cm2, is........Pa. (Density of water =103kg.m−3)
View SolutionA horizontal pipeline carries water in a streamline flow. At a point along the pipe, where the cross- sectional area is 10cm2, the water velocity is 1ms−1 and the pressure is 2000 Pa. The pressure of water at another point where the cross-sectional area is 5cm2, is........Pa. (Density of water =103kg.m−3)
View SolutionA stream of water of density ρ, cross sectional area A, and speed u strikes a wall that is perpendicular to the direction of the stream, as shown in the figure below. The water then flows sideways across the wall. The force exerted by the stream on the wall is
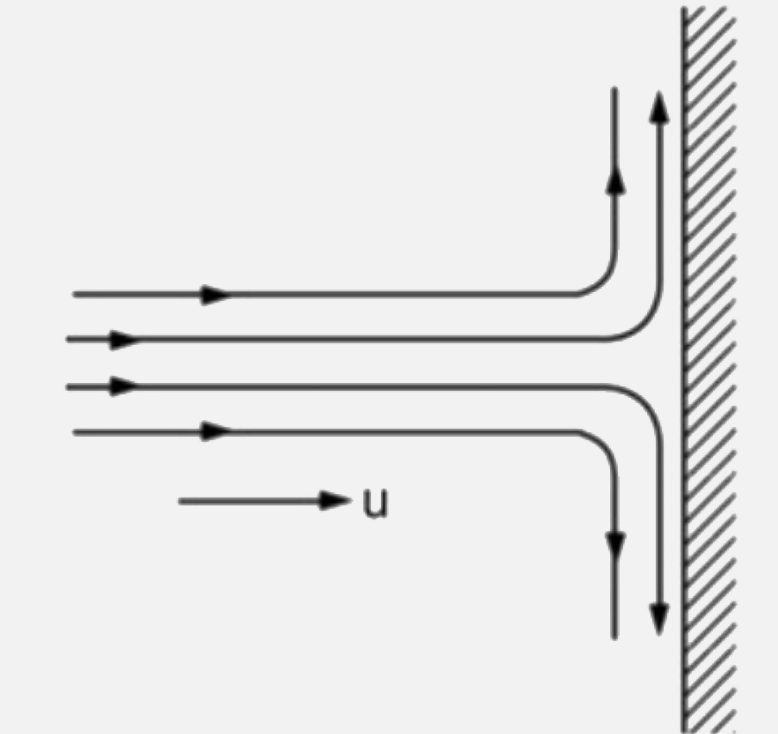 View Solution
View SolutionWater is moving with a speed of 5.18ms−1 through a pipe with a cross-sectional area of 4.20cm2. The water gradually descends 9.66m as the pipe increase in area to 7.60cm2. The speed of flow at the lower level is
View SolutionThe cross-section of a canal is a trapezium in shape. If the canal is 10m wide at the top 6m wide at the bottom and the area of cross-section is 72m2 determine its depth.
View Solution

