A car approaching a crossing C at a speed of 20m/s sounds a horn of frequency 500HZ when 80m from the crossing . Speed of sound in air is 330m/s . What frequency is heard by an observer (at rest) 60m from the crossing on the straight road which crosses car road at right angles ?
To solve the problem step by step, we will apply the principles of the Doppler effect for sound waves.
Step 1: Understand the scenario
A car is approaching a crossing at a speed of 20m/s and sounding a horn of frequency 500Hz. The speed of sound in air is 330m/s. The observer is located 60m from the crossing, and the car is 80m away from the crossing.
Step 2: Determine the position of the observer and the car
- The car is approaching the crossing from a distance of 80m.
- The observer is located 60m from the crossing along a perpendicular road.
Step 3: Calculate the distance from the observer to the car
Using the Pythagorean theorem, we can find the distance PO from the observer to the car:
PO=√(80m)2+(60m)2=√6400+3600=√10000=100m
Step 4: Calculate the angle θ
Using the right triangle formed:
cosθ=adjacenthypotenuse=80100=0.8
Step 5: Determine the components of the velocities
- The velocity of the source Vs=20m/s.
- The velocity of sound V=330m/s.
- The observer is at rest, so Vo=0m/s.
Step 6: Apply the Doppler effect formula
The formula for the apparent frequency f′ heard by the observer is given by:
f′=f⋅V+VoV−Vscosθ
Substituting the known values:
f′=500⋅330+0330−20⋅0.8
Step 7: Calculate Vscosθ
Vscosθ=20⋅0.8=16m/s
Thus, the denominator becomes:
330−16=314m/s
Step 8: Substitute and simplify
Now substituting back into the equation:
f′=500⋅330314
Calculating this gives:
f′=500⋅1.051=525.5Hz
Final Answer
The frequency heard by the observer is approximately 525.5Hz.
---
A car is approaching a crossing at a speed of 20m/s and sounding a horn of frequency 500Hz. The speed of sound in air is 330m/s. The observer is located 60m from the crossing, and the car is 80m away from the crossing.
- The car is approaching the crossing from a distance of 80m.
- The observer is located 60m from the crossing along a perpendicular road.
Using the Pythagorean theorem, we can find the distance PO from the observer to the car:
PO=√(80m)2+(60m)2=√6400+3600=√10000=100m
Using the right triangle formed:
cosθ=adjacenthypotenuse=80100=0.8
- The velocity of the source Vs=20m/s.
- The velocity of sound V=330m/s.
- The observer is at rest, so Vo=0m/s.
The formula for the apparent frequency f′ heard by the observer is given by:
f′=f⋅V+VoV−Vscosθ
Substituting the known values:
f′=500⋅330+0330−20⋅0.8
Vscosθ=20⋅0.8=16m/s
Thus, the denominator becomes:
330−16=314m/s
Now substituting back into the equation:
f′=500⋅330314
Calculating this gives:
f′=500⋅1.051=525.5Hz
The frequency heard by the observer is approximately 525.5Hz.
Topper's Solved these Questions
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Example Type 1
4 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Example Type 2
2 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 Subjective
8 Videos
Similar Questions
A locomotive approaching a crossing at a speed of 80 mi/h sounds a whistle of frequency 400 Hz when 1 mi from the crossing. There is no wind, and the speed of sound in air is 0.200mi/s. What frequency is heard by an observer 0.60 mi from the crossing on the straight road which crosses the railroad at right angles?
View SolutionA locomotive approaching a crossing at a speed of 80 mi/h sounds a whistle of frequency 400 Hz when 1 mi from the crossing. There is no wind, and the speed of sound in air is 0.200mi/s. What frequency is heard by an observer 0.60 mi from the crossing on the straight road which crosses the railroad at right angles?
View SolutionA locomotive approaching a crossing at a speed of 20 ms−1 sounds a whistle of frequency 640 Hz when 1 km from the crossing. There is no wind and the speed of sound in air is 330 ms−1. What frequency is heard by an observer √3 km on the straight road from the crossing at right angle :-
View SolutionA train approachign a railway crossing at a speed of 120kmh−1 sounds a short whistle at frequency 640 Hz when it is 300 m away from the crossing. The speed of sound in air is 340ms−1. What will be the frequency heard by a person standing on a road perpendicular to the track through the crossing at a distance of 400 m from the crossing ?
View SolutionA source of sound of frequency 500 Hz is moving towards an observer with velocity 30 m/s . The speed of sound is 330 m/s . the frequency heard by the observer will be
View SolutionA car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
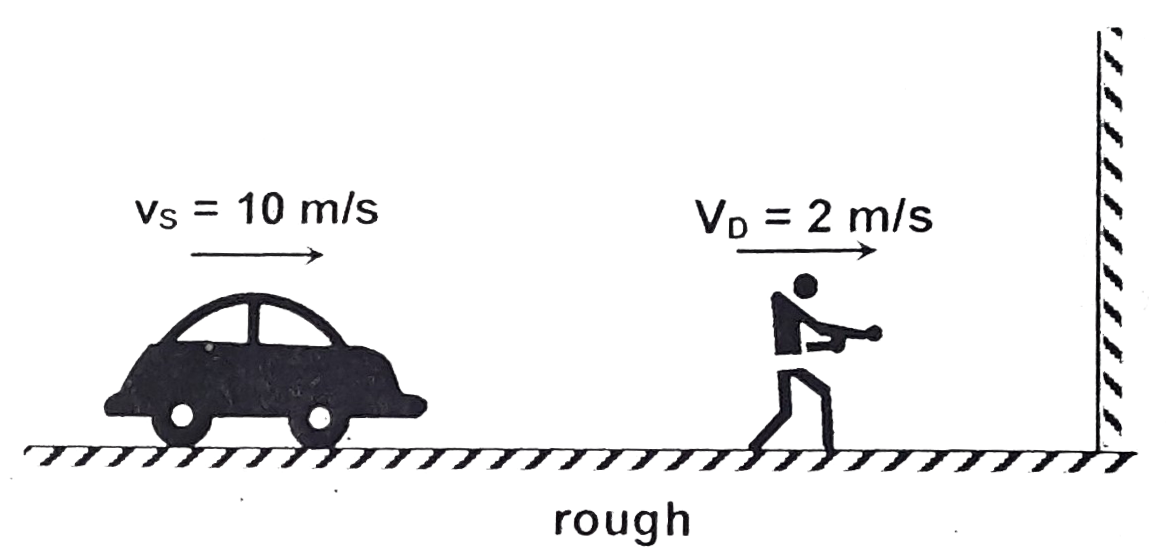
View SolutionThe driver of a car travelling with speed 30ms−1 towards a hill sounds a horn of frequency 600 Hz. If the velocity of sound in air is 330ms−1, the frequency of reflected sound as heard by driver is
View SolutionA police car horn emits a sound at a frequency 240 Hz. When the car is at rest. If the speed of the sound is 330 m/s the frequency heard by an observer who is approching the car at a speed of 11 m/s is
View SolutionA car is approching a railway crossing at a speed of 72kmph. It sounds a horn, when it is 800m away, at 600Hz. If the velocity of sound in air is 330ms−1. The apparent frequency as received by a man at rest near the railway track perpendicular to the road at a distance of 600m from the crossing the

View SolutionA source of sound of frequency 600Hz is placed inside of water. The speed of sound is water is 1500m/s and air it is 300m/s. The frequency of sound recorded by an observer who is standing in air is
View Solution
