If the length of the filament of a heater is reduced by , the power of the heater will
increase by about
increase by about
increase by about
decrease by about
The correct Answer is:B
To solve the problem of how the power of a heater changes when the length of its filament is reduced by 10%, we can follow these steps:
1. Understand the relationship between power, resistance, and length:
The power P of a heater can be expressed using the formula:
P=V2R
where V is the voltage across the heater and R is the resistance of the filament.
2. Relate resistance to length:
The resistance R of the filament can be expressed as:
R=ρLA
where ρ is the resistivity of the material, L is the length of the filament, and A is the cross-sectional area of the filament.
3. Substitute resistance into the power formula:
By substituting the expression for resistance into the power formula, we get:
P=V2AρL
4. Calculate the new length after reduction:
If the length of the filament is reduced by 10%, the new length L′ is:
L′=L−0.1L=0.9L
5. Determine the new power with the reduced length:
Substitute L′ into the power formula:
P′=V2AρL′=V2Aρ(0.9L)=V2A0.9ρL
This can be simplified to:
P′=10.9⋅V2AρL=P0.9
6. Calculate the ratio of new power to old power:
The ratio of the new power P′ to the old power P is:
P′P=10.9≈1.111
7. Determine the percentage increase in power:
The percentage increase in power can be calculated as:
Percentage Increase=(P′−PP)×100=(1.111P−PP)×100=(1.111−1)×100≈11.1%
Final Answer:
The power of the heater will increase by approximately 11% when the length of the filament is reduced by 10%.
The power P of a heater can be expressed using the formula:
P=V2R
where V is the voltage across the heater and R is the resistance of the filament.
The resistance R of the filament can be expressed as:
R=ρLA
where ρ is the resistivity of the material, L is the length of the filament, and A is the cross-sectional area of the filament.
By substituting the expression for resistance into the power formula, we get:
P=V2AρL
If the length of the filament is reduced by 10%, the new length L′ is:
L′=L−0.1L=0.9L
Substitute L′ into the power formula:
P′=V2AρL′=V2Aρ(0.9L)=V2A0.9ρL
This can be simplified to:
P′=10.9⋅V2AρL=P0.9
The ratio of the new power P′ to the old power P is:
P′P=10.9≈1.111
The percentage increase in power can be calculated as:
Percentage Increase=(P′−PP)×100=(1.111P−PP)×100=(1.111−1)×100≈11.1%
The power of the heater will increase by approximately 11% when the length of the filament is reduced by 10%.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - OBJECTIVE_TYPE
1 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 1 Subjective
53 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Level 1 Assertion And Reason
11 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Medical entrances gallery
25 Videos
Similar Questions
The voltage across a bulb is decreased by 2%. Assuming that the resistance of the filament remains unchanged, the power of the bulb will
View SolutionA cylinderical brass boiler of radius 15 cm and thickness 1.0 cm is filled with water and placed on an elerctric heater. If the If the water boils at the rate of 200g/s, estimate the temperature of the heater filament. Thermal conductivity of brass=109J/s/m∘C and heat of vapourization of water =2.256×103J/g.
View Solution
Knowledge Check
If the length of a rectangle is increased by 30% and its width is increased by 10%, by what percentage will be the area of the rectangle be increased?
A0.33B0.37C0.4D0.43
A gas is filled in a cylinder. its temperature is increased by 20% on the Kelvin scale and volume is reduced by 10% How much percentage of the gas has to leak for pressure to remind constant ?
View SolutionIf a given volume of water in a 220V heater is boiled in 5min, then how much time will it take for the same volume of water in a 110V heater to be boiled?
View SolutionTwo point charges repel each other with a force of 100N. One of the charges is increased by 10% and the other is reduced by 10% . The new force of repulsion at the same distance would be
View SolutionA heater joined in series with a 50W bulb is connected to the mains . If the 50W bulb is replaced by a 100W bulb , then will the heater now give more heat , less heat, or same heat ? Why ?
View SolutionThe resistance of the filament of a lamp increases with the increase in temperature. A lamp rated 100W and 220V is connected across 220V power supply. If the voltage drops by 10%, then the power of the lamp will be
View SolutionAn ideal monoatomic gas is confined in a cylinder by a spring-loaded piston of cross-section 8.0×10−3m2. Initially the gas is at 300K and occupies a volume of 2.4×10−3m3 and the spring is in its relaxed (unstretched, unompressed) state, fig. The gas is heated by a small electric heater until the piston moves out slowly by 0.1m. Calculate the final temperature of the gas and the heat supplied (in joules) by the heater. The force constant of the spring is 8000N/m, atmospheric pressure is 1.0×105Nm−2. The cylinder and the piston are thermally insulated. The piston is massless and there is no friction between the piston and the cylinder. Neglect heat loss through lead wires of the heater. The heat capacity of the heater coil is negligible. Assume the spring to be massless.
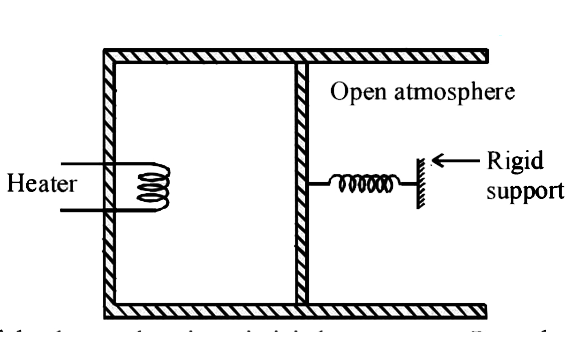
View SolutionAn ideal monoatomic gas is confined in a cylinder by a spring-loaded piston of cross-section 8.0×10−3m2. Initially the gas is at 300K and occupies a volume of 2.4×10−3m3 and the spring is in its relaxed (unstretched, unompressed) state, fig. The gas is heated by a small electric heater until the piston moves out slowly by 0.1m. Calculate the final temperature of the gas and the heat supplied (in joules) by the heater. The force constant of the spring is 8000N/m, atmospheric pressure is 1.0×105Nm−2. The cylinder and the piston are thermally insulated. The piston is massless and there is no friction between the piston and the cylinder. Neglect heat loss through lead wires of the heater. The heat capacity of the heater coil is negligible. Assume the spring to be massless.

View Solution
