The figure shows the variation of photocurrent with anode potential for a photosensitve surface for three different radiations. Let la,lbandlc be the curves a, b and c, respectively 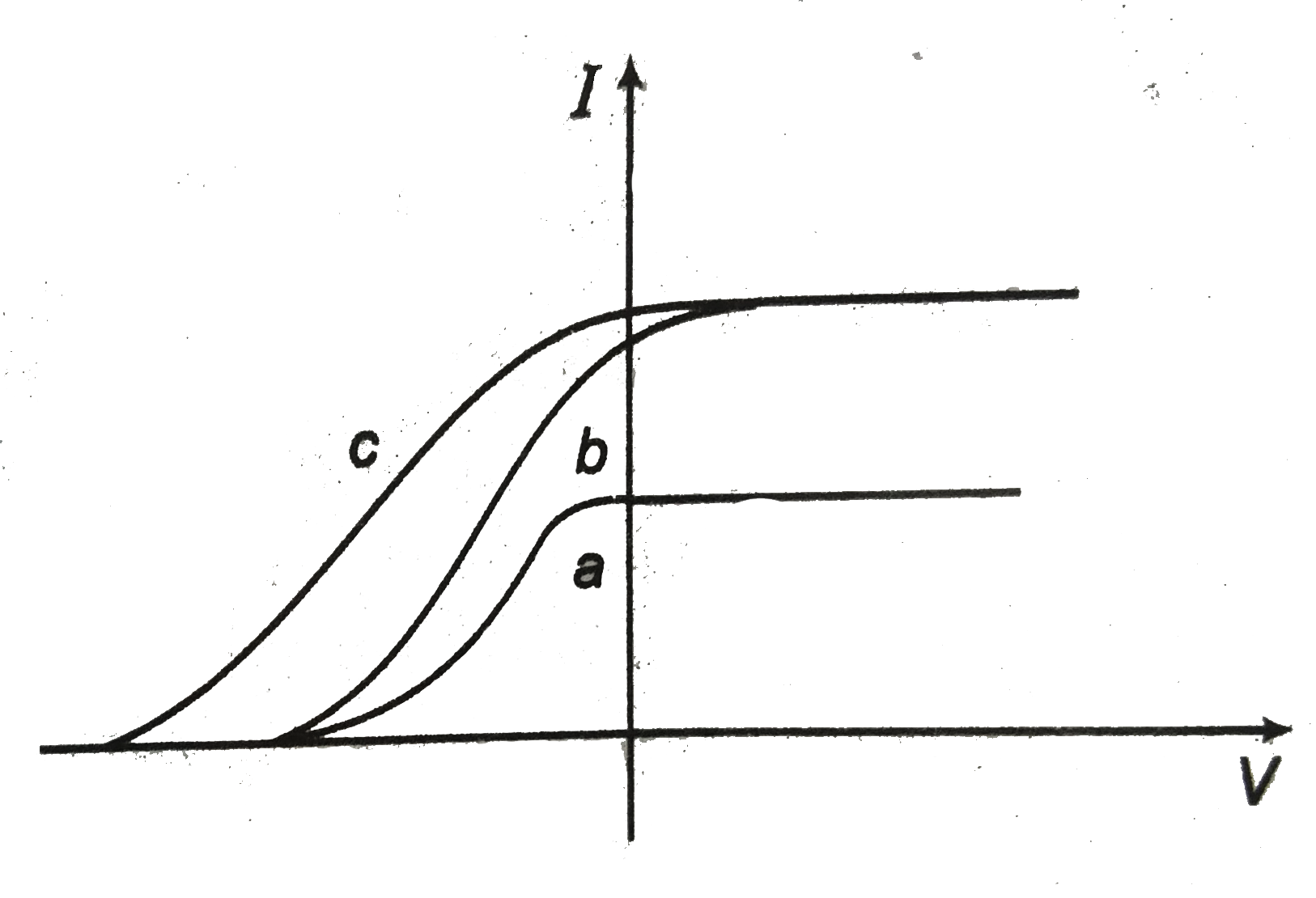
(a) fa=fbandla≠lb (b) fa=fcandla=lc
(c ) fa=fbandla=lb (d) fb=fcandlb=lc
The correct Answer is:A
Saturation current is proportional to intensity while
stopping potential increases with increases in
frequency.
Hence, fa=fbwhi≤ia<lb
Therefore, the correct option is (a).
Topper's Solved these Questions
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level -1 Assertion And Reason
10 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Level 1 Objective
37 Videos
- MODERN PHYSICS - 1BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 1EXERCISE - Exercise 33.3
6 Videos
- MODERN PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICSEXERCISE - Integer Type Questions
17 Videos
- MODERN PHYSICS - 2BOOK - DC PANDEY ENGLISHCHAPTER - MODERN PHYSICS - 2EXERCISE - Level 2 Subjective
10 Videos
Similar Questions
Knowledge Check
The fig. shows the variation of photon current with anode potential for a photo-sensitive surface for three different radiation. Let Ia,IbandIc be the intensities and fa,fbandfc be the frequency for the curves a,b and c respectively.
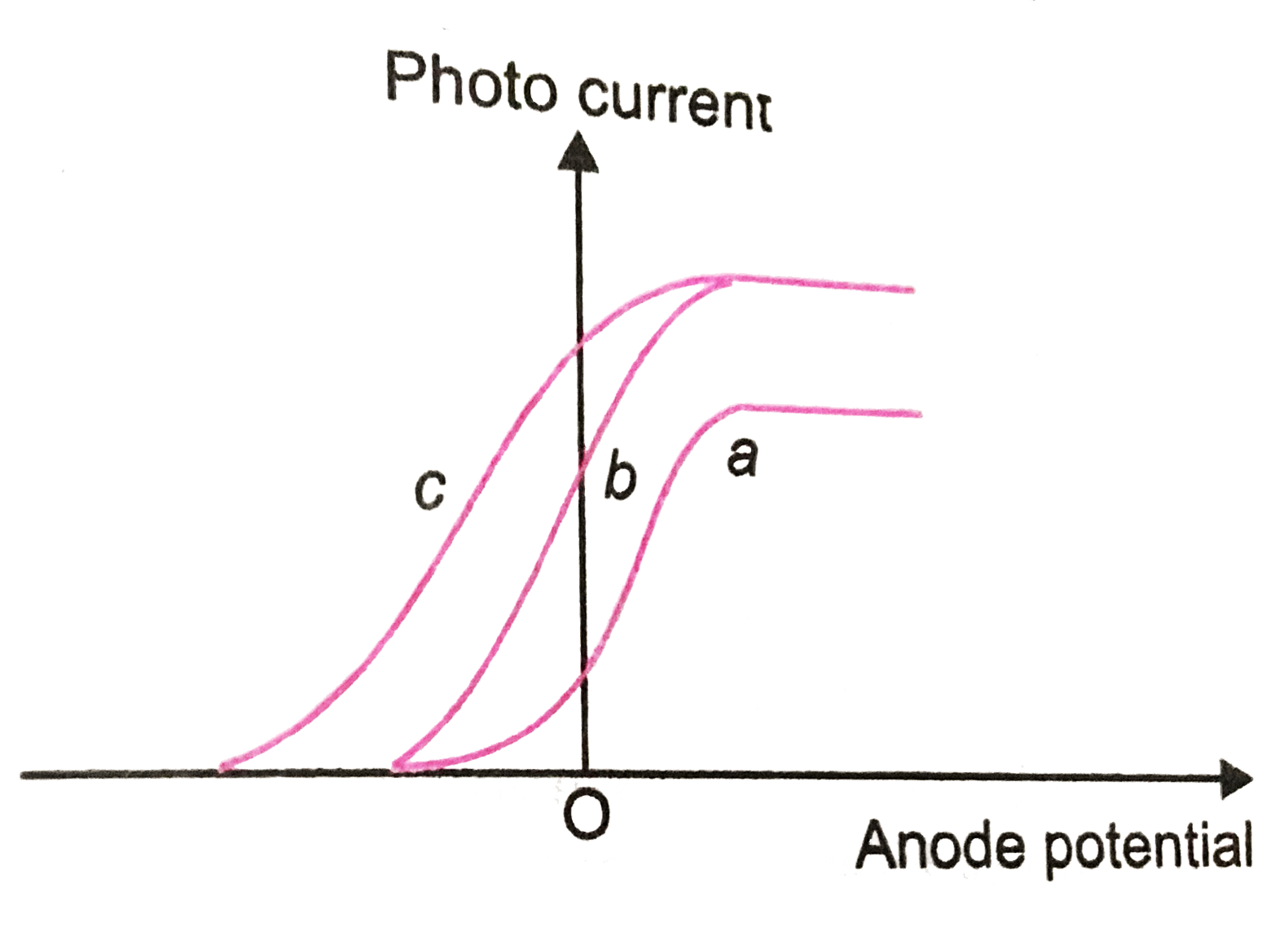 Ava=vbandIa≠IbBva=vcandIa=IcCva=vbandIa=IbDvb=vcandIb=Ic
Ava=vbandIa≠IbBva=vcandIa=IcCva=vbandIa=IbDvb=vcandIb=Ic
If the dimensions of B2l2C are [MaLbTc], then the value of a+b+c is
[Here B,l and C represent the magnitude of magnetic field, length and capacitance respectively]
View SolutionABCD is a parallelogram. L and M are points on AB and DC respectively and AL=CM. prove that LM and BD bisect each other.
View SolutionIn Figure, AD is a median and BL, CM are perpendiculars drawn from B and C respectively on AD and AD produced. Prove that BL=CM
View SolutionWhen a string is divided into three segments of length l1,l2 and l3 the fundamental frequencies of these three segments are f1,f2 and f3 respectively. The original fundamental frequency f of the string is
View SolutionConsider two sets A = {a, b}, B = {e, f}. If maximum numbers of relations from A to B, A to A, B to B are l, m, n respectively then the value of 2l - m - n is
View SolutionIn a △ABC, If L and M are points on AB and AC respectively such that LM∣∣BC. Prove that: ar ( △LCM)=ar (△LBM)
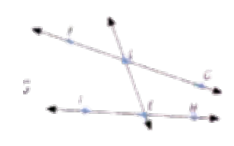
View SolutionIn the given figure . Which of the following are non collinear points ?
L and E
(b) F.L and G
( c ) I. E and H
(d) F.L and E
View Solution
