Potential energy v/s position curve for one dimensional conservative field is shown. Force at A and B is respectively.

Positive, Positive
Positive, Negative
Negative, Positive
Negative, Negative
The correct Answer is:B
Step by step video & image solution for Potential energy v//s position curve for one dimensional conservative field is shown. Force at A and B is respectively. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - A Only One Option is Correct
42 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - B More than One Option is Correct
26 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
Potential energy of a body in position A is-40J. Work done by conservative force in moving the body from A to B is−20J. Find potential energy of the body in position B.
View SolutionThe potential energy of a particle in a conservative field is U =a/r^3-b/r^2, where a and b are positive constants and r is the distance of particle from the centre of field. For equilibrium, the value of r is
View SolutionThe potential energy ‘V’ of a particle moving along the positive x-direction in a conservative force field varies as shown in the figure. The total energy is E=2. Draw the corresponding kinetic energy K vs x graph.
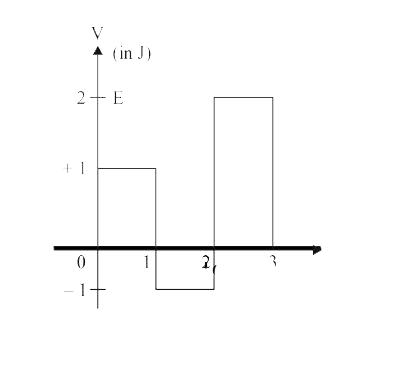
View SolutionA : The potential energy can be defined only in conservative field.
R : The value of potential energy depends on the reference level (level of zero potential energy).
View SolutionSTATEMENT-1 Positive potential energy means that a positive work will be done on the body by the conservative force while returning to its reference position.
STATEMENT-2 The absolute value of potential energy depends on reference.
View SolutionA : The potential energy of a system increases when work is done by conservative force.
R : Kinetic energy can change into potential energy and vice-versa.
View SolutionA potential energy function for a two-dimensional force is the form U=3x2y−7x. Find the force that acts at the point (x,y).
View SolutionThe potential energy of an object of mass m moving in xy plane in a conservative field is given by U = ax + by , where x and y are position coordinates of the object. Find magnitude of its acceleration :-
View SolutionThe potential energy of a conservative force field is given by
U=ax2−bx
where, a and b are positive constants. Find the equilibrium position and discuss whether the equilibrium is stable, unstable or neutral.
View SolutionA particle moves in one dimension in a conservation force field. The potential energy is depicted in the graph below.
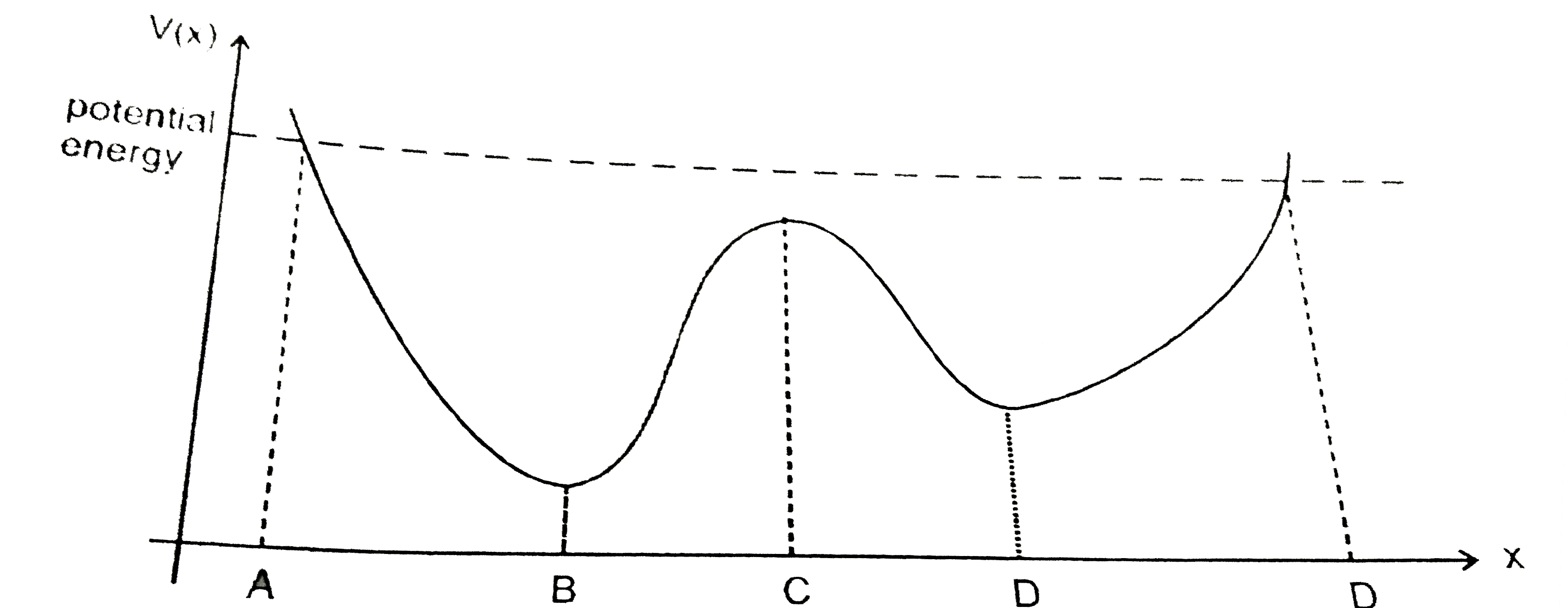
If the particle starts to move from rest from the point A, then
View Solution
