What is β value for transistor whose α=0.98?
29
38
49
56
The correct Answer is:C
To find the value of β for a transistor given that α=0.98, we can use the relationship between α and β. The formula is:
β=α1−α
1. Write down the formula: Start with the relationship between α and β.
\(
\beta = \frac{\alpha}{1 - \alpha}
\)
2. Substitute the value of α: We know that α=0.98, so we substitute this value into the formula.
\(
\beta = \frac{0.98}{1 - 0.98}
\)
3. Calculate 1−α: Now, calculate 1−0.98.
\(
1 - 0.98 = 0.02
\)
4. Substitute back into the equation: Replace 1−α in the formula with 0.02.
\(
\beta = \frac{0.98}{0.02}
\)
5. Perform the division: Now, divide 0.98 by 0.02.
\(
\beta = 49
\)
6. Conclusion: The value of β for the transistor is 49.
Final Answer:
\(
\beta = 49
\)
\(
\beta = \frac{\alpha}{1 - \alpha}
\)
\(
\beta = \frac{0.98}{1 - 0.98}
\)
\(
1 - 0.98 = 0.02
\)
\(
\beta = \frac{0.98}{0.02}
\)
\(
\beta = 49
\)
\(
\beta = 49
\)
Topper's Solved these Questions
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- ROTATIONAL MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - ROTATIONAL MOTIONEXERCISE - Integer Type Questions
17 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Integer type questions
14 Videos
Similar Questions
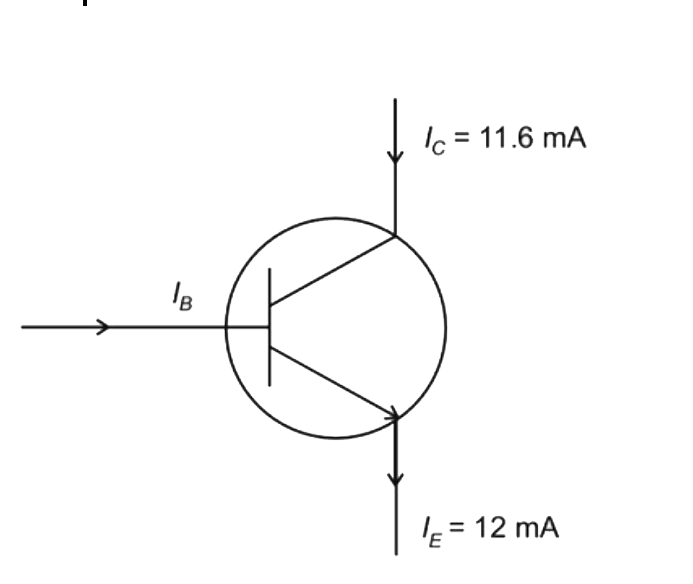
The value of transistor parameters , βandα respectively, for the given figure is
View SolutionThe value of β of a transistor is 19. The value of α will be
View SolutionChoose the corrector relation between the transistor parameters α and β.
View SolutionThe relation between α and β of a transistor is
View SolutionGive a relation between αandβ for a transistor. (Derivation is not required)
View SolutionIn a transistor, the value of α=0.9. Then the value of β is:
View SolutionIf αandβ are the roots of the equation x2+x+1=0, then what is the equation whose roots are α19andβ7 ?
View SolutionThe roots of the equation ax2+bx+c=0 are αandβ. Form the quadratic equation whose roots are α+1βandβ+1α.
View SolutionIf sinα=Asin(α+β),A≠0, then
The value of tanα is
View SolutionThe relation between α and β parameters of current gains for a transistors is given by
View Solution
